
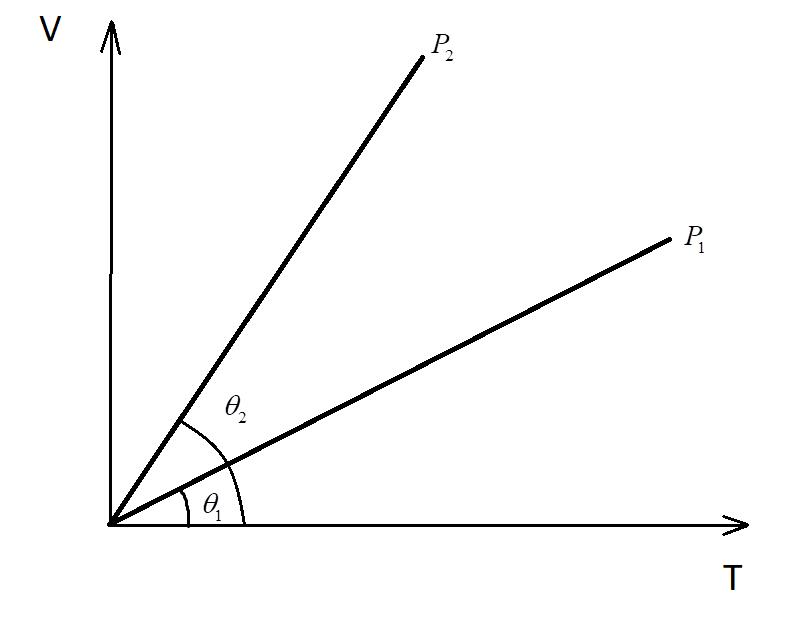
In the given (V-T) diagram, what is the relation between pressures ${P_1}$ and ${P_2}$?

Answer
435.6k+ views
Hint: The linear curve is passing through origin in volume (V) versus temperature (T) suggests that the given thermodynamic process is isobaric. In the isobaric process, the pressure of the thermodynamic system remains constant. To get the required relationship between the pressures for the given curves we will compare the slope of each curve and use the relation: slope $ = \left( {\dfrac{{nR}}{P}} \right)$, where n is the number of moles, R is the universal gas constant and P is the pressure.
Complete answer:
Consider the volume-temperature graph with two lines at different pressures ${P_1}$ and ${P_2}$.
Step I: Establish the relation between volume
An isobaric process is a constant pressure process. i.e., in an isobaric process, we have
$P = $Constant…………. (i)
By using ideal gas law:
$PV = nRT$
$ \Rightarrow \dfrac{V}{T} = \dfrac{{nR}}{P}$………………….. (ii)
Using equation (i) in (ii), we get
$ \Rightarrow \dfrac{V}{T} = $Constant
$ \Rightarrow V = CT$…………………… (iii), where C is a constant which is equal to $\left( {\dfrac{{nR}}{P}} \right)$.
∴ $V \propto T$
From equation (ii) we can say that the V-T graph is a straight line passing through the origin.
Step II: Calculate the slope of the isobaric curve.
From equation (ii)
$V = \dfrac{{nRT}}{P}$……. (iv)
Differentiating equation (iv) with respect to T.
$\dfrac{{dV}}{{dT}} = \dfrac{{nR}}{P} = $Slope
i.e., Slope$ \propto \dfrac{1}{P}$
So, if the slope of the curve increases then the pressure will decrease and vice versa.
Since from the given V-T graph:
${\theta _2} > {\theta _1}$
$ \Rightarrow $The slope of curve 2 > Slope of curve 1
$ \Rightarrow {P_2} < {P_1}$
Hence, the pressure of the second is lesser than the pressure in the first curve.
Note:
Students should note that in the isobaric process the pressure will remain constant and the V-T graph for the process is a straight line passing through the origin. While in the case of the P-V graph it will be horizontal and parallel to the volume axis.
Complete answer:
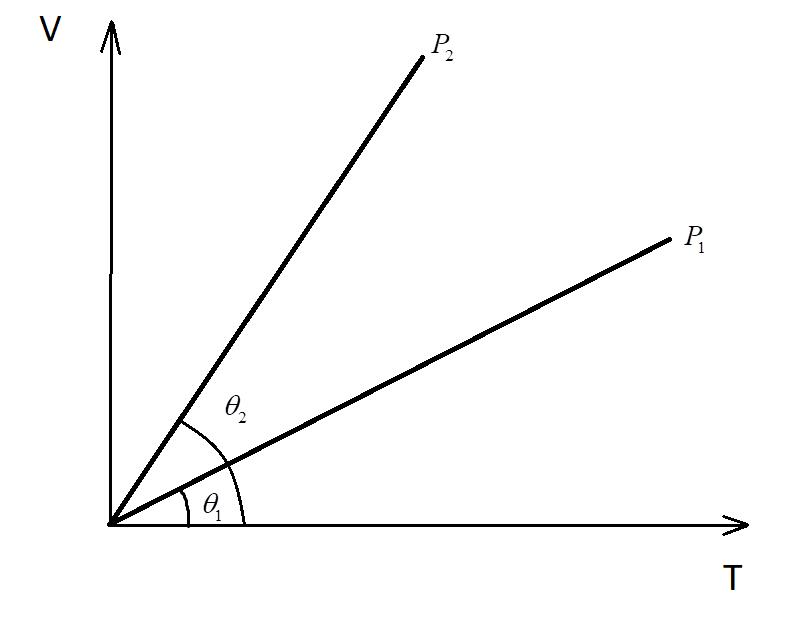
Consider the volume-temperature graph with two lines at different pressures ${P_1}$ and ${P_2}$.

Step I: Establish the relation between volume
An isobaric process is a constant pressure process. i.e., in an isobaric process, we have
$P = $Constant…………. (i)
By using ideal gas law:
$PV = nRT$
$ \Rightarrow \dfrac{V}{T} = \dfrac{{nR}}{P}$………………….. (ii)
Using equation (i) in (ii), we get
$ \Rightarrow \dfrac{V}{T} = $Constant
$ \Rightarrow V = CT$…………………… (iii), where C is a constant which is equal to $\left( {\dfrac{{nR}}{P}} \right)$.
∴ $V \propto T$
From equation (ii) we can say that the V-T graph is a straight line passing through the origin.
Step II: Calculate the slope of the isobaric curve.
From equation (ii)
$V = \dfrac{{nRT}}{P}$……. (iv)
Differentiating equation (iv) with respect to T.
$\dfrac{{dV}}{{dT}} = \dfrac{{nR}}{P} = $Slope
i.e., Slope$ \propto \dfrac{1}{P}$
So, if the slope of the curve increases then the pressure will decrease and vice versa.
Since from the given V-T graph:
${\theta _2} > {\theta _1}$
$ \Rightarrow $The slope of curve 2 > Slope of curve 1
$ \Rightarrow {P_2} < {P_1}$
Hence, the pressure of the second is lesser than the pressure in the first curve.
Note:
Students should note that in the isobaric process the pressure will remain constant and the V-T graph for the process is a straight line passing through the origin. While in the case of the P-V graph it will be horizontal and parallel to the volume axis.
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Trending doubts
10 examples of friction in our daily life

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

State and prove Bernoullis theorem class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

Write down 5 differences between Ntype and Ptype s class 11 physics CBSE




