
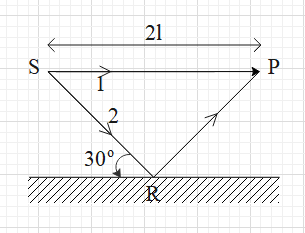
Interference pattern is observed at P due to superposition of two waves coming out from a source ‘S’ as shown in the figure. The value of ‘l’ for which maxima is obtained at ‘P’ is (R is perfect reflecting surface)

Answer
479.7k+ views
4 likes
Hint: When two waves from the same source superimpose at a point, maxima is obtained at the point if the path difference between the two waves is an integer multiple of the wavelength of the wave. Find the path of both waves. Find the difference in both and find the value of l.
Formula used:’
Complete step-by-step answer:
When two waves from the same source superimpose at a point, maxima is obtained at the point if the path difference between the two waves is an integer multiple of the wavelength of the wave.
i.e.
The wave 1 is directly reaching the point P. Therefore, the path covered by it is equal to
Let us measure the path covered by wave 2. We can see that this wave reflects at surface R and then reaches point P.
From the figure the incident angle (i) at the surface R is
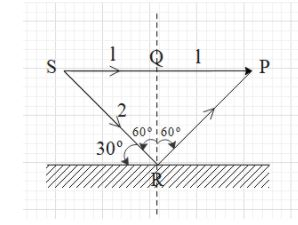
Now, consider
In this triangle,
The
The path covered by the wave 2 is
However, the wave 2 is reflecting at surface R, and will change its phase by
Therefore, the total path of wave 2 is
The path difference will be
And
So, the correct answer is “Option C”.
Note: If we do not know that a change in phase by
Formula used:’
Complete step-by-step answer:
When two waves from the same source superimpose at a point, maxima is obtained at the point if the path difference between the two waves is an integer multiple of the wavelength of the wave.
i.e.
The wave 1 is directly reaching the point P. Therefore, the path covered by it is equal to
Let us measure the path covered by wave 2. We can see that this wave reflects at surface R and then reaches point P.
From the figure the incident angle (i) at the surface R is

Now, consider
In this triangle,
The
The path covered by the wave 2 is
However, the wave 2 is reflecting at surface R, and will change its phase by
Therefore, the total path of wave 2 is
The path difference will be
And
So, the correct answer is “Option C”.
Note: If we do not know that a change in phase by
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




