
Justify the statement – “Uniform circular motion is an accelerated motion “. Hence derive an expression for the centripetal acceleration of an object in a uniform circular motion in a plane.
Answer
504.6k+ views
Hint: Uniform circular motion in a plane means that the angular velocity of the particle undergoing circular motion is constant with time. One should always take care of the difference between scalar and vector quantity. Speed is a scalar quantity and on the other, velocity is a vector quantity. If the speed is constant, it doesn’t imply that the acceleration is zero but if the velocity is constant, it always implies that acceleration is zero or motion is non-accelerated.
Formula used:
Complete step by step answer:
To understand the statement mathematically, let’s consider a particle undergoing circular motion with angular speed
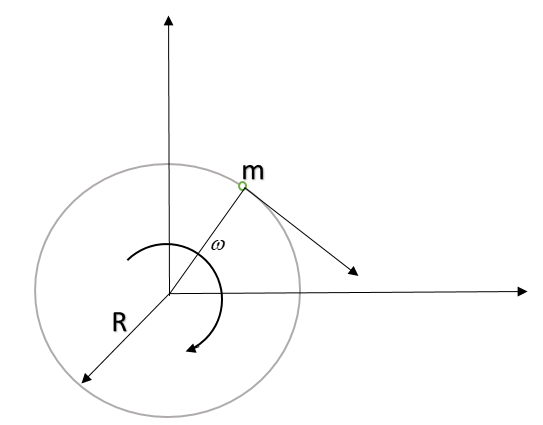
Starting from t=0, the angle turned by the particle after ‘t’ time will be
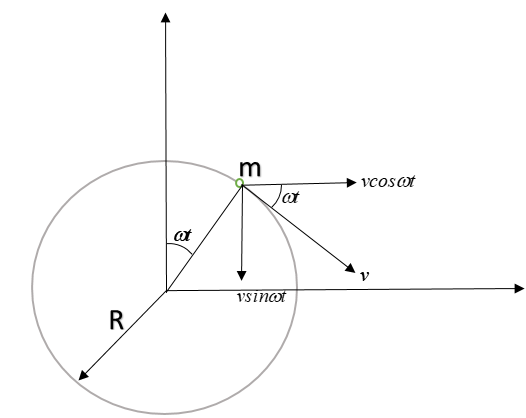
Let the magnitude of linear velocity of the particle be ‘
To find the acceleration of the particle, let's differentiate the velocity expression.
Putting
Hence we can clearly see that acceleration is non zero. Hence uniform circular motion is accelerated motion. So, the statement is justified.
Expression for centripetal acceleration of a body undergoing circular motion:
As we derived
To find the magnitude:
Hence centripetal acceleration is
Note:
As we derived the expression for the centripetal acceleration of the body undergoing uniform circular motion, we can see that the magnitude of this acceleration is constant with time. One should always analyze the quantities with their directions and magnitude separately. For example, the magnitude of centripetal acceleration is
Formula used:
Complete step by step answer:
To understand the statement mathematically, let’s consider a particle undergoing circular motion with angular speed

Starting from t=0, the angle turned by the particle after ‘t’ time will be

Let the magnitude of linear velocity of the particle be ‘
To find the acceleration of the particle, let's differentiate the velocity expression.
Putting
Hence we can clearly see that acceleration is non zero. Hence uniform circular motion is accelerated motion. So, the statement is justified.
Expression for centripetal acceleration of a body undergoing circular motion:
As we derived
To find the magnitude:
Hence centripetal acceleration is
Note:
As we derived the expression for the centripetal acceleration of the body undergoing uniform circular motion, we can see that the magnitude of this acceleration is constant with time. One should always analyze the quantities with their directions and magnitude separately. For example, the magnitude of centripetal acceleration is
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Find the missing frequency in the following distribution class 11 maths CBSE

Difference between physical and chemical change class 11 chemistry CBSE

Number of oneone functions from A to B where nA 4 and class 11 maths CBSE




