
Kinetic energy of the system in centre of mass frame is-
(A).
(B).
(C).
(D).
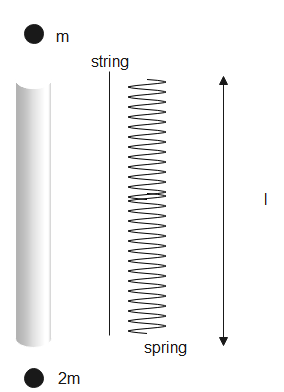
(a)
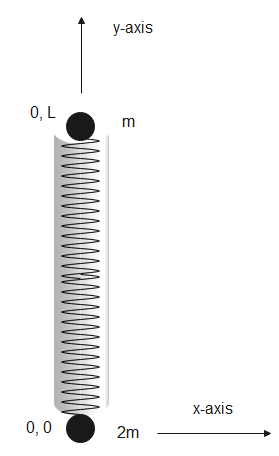
(b)


Answer
455.1k+ views
Hint: The system contains two masses connected to a cylinder with a spring of length L. The system will rotate about its centre of mass. The centre will lie more towards the heavier mass and the motion is purely rotational. The rotational kinetic energy is analogous to translational kinetic energy and it depends on the moment of inertia and rotational velocity.
Formulas used:
Complete answer:
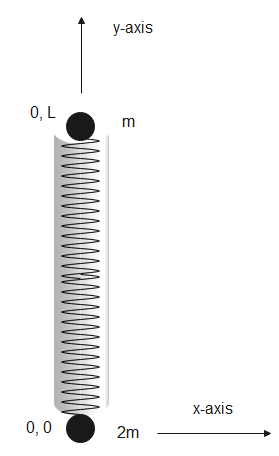
In the given figure, the cylinder will have only rotational kinetic energy.
The position of centre of mass for the frame is given by-
Here,
Given,
Therefore the position of the centre of mass is at
The rotational kinetic energy is given by-
Here,
The moment of inertia for the cylinder is
Let the initial angular velocity be
Therefore, the kinetic energy of the system about its centre of mass is
Hence, the correct option is (C).
Note:
The motion of the system was pure rotational. The centre of mass is the point of symmetry of the system. Since, the system lies entirely on the y-axis, its centre of mass will also lie on the y-axis. The moment of inertia is analogous to the mass. The system is an isolated system as no external force or torque is acting on it.
Formulas used:
Complete answer:

In the given figure, the cylinder will have only rotational kinetic energy.
The position of centre of mass for the frame is given by-
Here,
Given,
Therefore the position of the centre of mass is at
The rotational kinetic energy is given by-
Here,
The moment of inertia for the cylinder is
Let the initial angular velocity be
Therefore, the kinetic energy of the system about its centre of mass is
Hence, the correct option is (C).
Note:
The motion of the system was pure rotational. The centre of mass is the point of symmetry of the system. Since, the system lies entirely on the y-axis, its centre of mass will also lie on the y-axis. The moment of inertia is analogous to the mass. The system is an isolated system as no external force or torque is acting on it.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




