
What is the lateral displacement when a ray of light falls normally on a glass slab?
Answer
441.5k+ views
1 likes
- Hint: Use Snell’s law i.e.
Complete step-by-step solution -
A glass slab is in cuboidal shape (opposite surfaces parallel to each other). Let us first understand what happens when a light ray enters a glass slab. When a light ray passes through a glass slab it is refracted twice at the two parallel faces and finally emerges parallel to its incident direction. Suppose a light ray enters the glass slab from air. It will first be refracted at this surface.
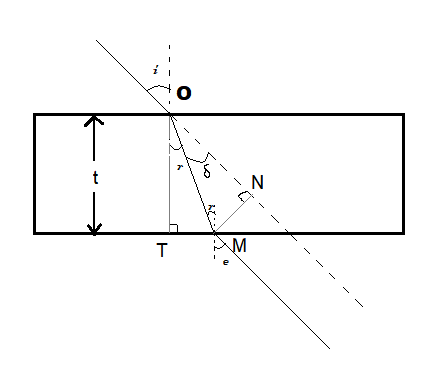
Use Snell’s law i.e.
Afterwards, the refracted ray will travel through the glass and will be incident on the opposite surface. Here, as you can see in the given figure, the angle of incident at surface 2 will be equal to the angle of refraction at surface 1 (since the normal at the two surfaces are parallel).
Let the angle of refraction at surface two be ‘e’. Now, use Snell’s law.
Compare equations (1) and (2). We get,
As you can see in the given figure,
In
Substitute the value of OM from equation (4) into equation (3).
Therefore, we got the expression for lateral displacement. Now, if the light ray is incident normally on the surface,
Note: When a light ray falls on a glass slab, it is not that there will be a lateral displacement every time. One example you saw in the question. Another example is the internal reflection of the ray. The ray does refract into the outside medium, but it is reflected back into the same medium. This is only when the ray travels from a denser medium to a rarer medium.
Complete step-by-step solution -
A glass slab is in cuboidal shape (opposite surfaces parallel to each other). Let us first understand what happens when a light ray enters a glass slab. When a light ray passes through a glass slab it is refracted twice at the two parallel faces and finally emerges parallel to its incident direction. Suppose a light ray enters the glass slab from air. It will first be refracted at this surface.

Use Snell’s law i.e.
Afterwards, the refracted ray will travel through the glass and will be incident on the opposite surface. Here, as you can see in the given figure, the angle of incident at surface 2 will be equal to the angle of refraction at surface 1 (since the normal at the two surfaces are parallel).
Let the angle of refraction at surface two be ‘e’. Now, use Snell’s law.
Compare equations (1) and (2). We get,
As you can see in the given figure,
In
Substitute the value of OM from equation (4) into equation (3).
Therefore, we got the expression for lateral displacement. Now, if the light ray is incident normally on the surface,
Note: When a light ray falls on a glass slab, it is not that there will be a lateral displacement every time. One example you saw in the question. Another example is the internal reflection of the ray. The ray does refract into the outside medium, but it is reflected back into the same medium. This is only when the ray travels from a denser medium to a rarer medium.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




