
What is lattice enthalpy? How do you calculate the lattice enthalpy of sodium chloride by using Born-Haber’s cycle?
Answer
505.8k+ views
4 likes
Hint: Lattice enthalpy is related to the formation of crystalline solid from its ions. The Born-Haber cycle is a stepwise process to calculate lattice enthalpy. Now, try to answer this question accordingly.
Complete step by step answer:
Let’s talk about the Lattice Enthalpy -
Lattice Enthalpy - The lattice enthalpy of a crystalline solid is a measure of the energy released when the ions are combined to make this compound.
The lattice enthalpy is indirectly determined by the use of the Born-Haber Cycle. This procedure is based on Hess's law.
Now, Let’s find the lattice enthalpy of sodium chloride by using Born-Haber’s cycle -
We begin by writing the formation reaction, which is by definition from the elemental states at 25
Our goal is to transform the reactants into their ionic gases, as that is the reaction that describes the process for which "lattice energy" is defined.
* Solid sodium atom sublimes to gaseous atom by absorbing heat energy.
* Gaseous sodium atoms absorb the ionization energy to release one electron and form gaseous sodium ions.
* Diatomic gaseous chlorine breaks into two individual atoms by absorbing bond energy, such that each chlorine atom absorbs half of the bond energy of chlorine molecules.
* Chlorine atoms accept an electron to form chloride ions and release energy equivalent to electron affinity.
* Gaseous sodium ion and gaseous chloride ion combine to form a solid sodium-chloride molecule and release energy equivalent to lattice energy.
Summation of enthalpy of all the processes from all five steps gives the net enthalpy of formation of solid crystalline sodium chloride from sodium and chlorine in their standard conditions of solid and gas respectively.
For 1 mol of NaCl(s) -
The enthalpies are represented as a cycle in the figure.
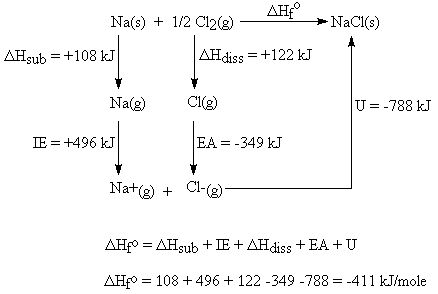
So,
or,
or, Lattice enthalpy (U or
U = -411 - (108+496+122-349)
U = -411 -108 -496 -122 +349
U = – 788 kJ/mol
Therefore, we defined the lattice enthalpy and calculated its value for Sodium Chloride.
Note: Hess law’s statement, “The enthalpy change of a reaction is the same at constant volume and pressure whether it takes place in single or multiple steps long as the initial reactants and the final products remain the same.”
Lattice energy is relevant to many practical properties including solubility, hardness, and volatility.
Complete step by step answer:
Let’s talk about the Lattice Enthalpy -
Lattice Enthalpy - The lattice enthalpy of a crystalline solid is a measure of the energy released when the ions are combined to make this compound.
The lattice enthalpy is indirectly determined by the use of the Born-Haber Cycle. This procedure is based on Hess's law.
Now, Let’s find the lattice enthalpy of sodium chloride by using Born-Haber’s cycle -
We begin by writing the formation reaction, which is by definition from the elemental states at 25
Our goal is to transform the reactants into their ionic gases, as that is the reaction that describes the process for which "lattice energy" is defined.
* Solid sodium atom sublimes to gaseous atom by absorbing heat energy.
* Gaseous sodium atoms absorb the ionization energy to release one electron and form gaseous sodium ions.
* Diatomic gaseous chlorine breaks into two individual atoms by absorbing bond energy, such that each chlorine atom absorbs half of the bond energy of chlorine molecules.
* Chlorine atoms accept an electron to form chloride ions and release energy equivalent to electron affinity.
* Gaseous sodium ion and gaseous chloride ion combine to form a solid sodium-chloride molecule and release energy equivalent to lattice energy.
Summation of enthalpy of all the processes from all five steps gives the net enthalpy of formation of solid crystalline sodium chloride from sodium and chlorine in their standard conditions of solid and gas respectively.
For 1 mol of NaCl(s) -
The enthalpies are represented as a cycle in the figure.

So,
or,
or, Lattice enthalpy (U or
U = -411 - (108+496+122-349)
U = -411 -108 -496 -122 +349
U = – 788 kJ/mol
Therefore, we defined the lattice enthalpy and calculated its value for Sodium Chloride.
Note: Hess law’s statement, “The enthalpy change of a reaction is the same at constant volume and pressure whether it takes place in single or multiple steps long as the initial reactants and the final products remain the same.”
Lattice energy is relevant to many practical properties including solubility, hardness, and volatility.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

In northern hemisphere 21st March is called as A Vernal class 11 social science CBSE




