
What is the length of a wave dipole at
A.
B.
C.
D. None of these.
Answer
505.5k+ views
Hint: In a half wave dipole antenna, the length of the half wave dipole is equal to the half of the wavelength of the wave. Hence, with the value of the wave length, the length of the half wave can be calculated. The wavelength can be calculated from the relationship between the speed, frequency and wavelength of the wave.
Useful formula:
The length of the half wave dipole,
Where,
The relationship between speed, wavelength and frequency of the wave,
Where,
Given data:
The frequency of the wave,
Complete step by step solution:
The relationship between speed, wavelength and frequency of the wave,
Since, the normal wave moves with the speed of light. So,
Substitute the values of
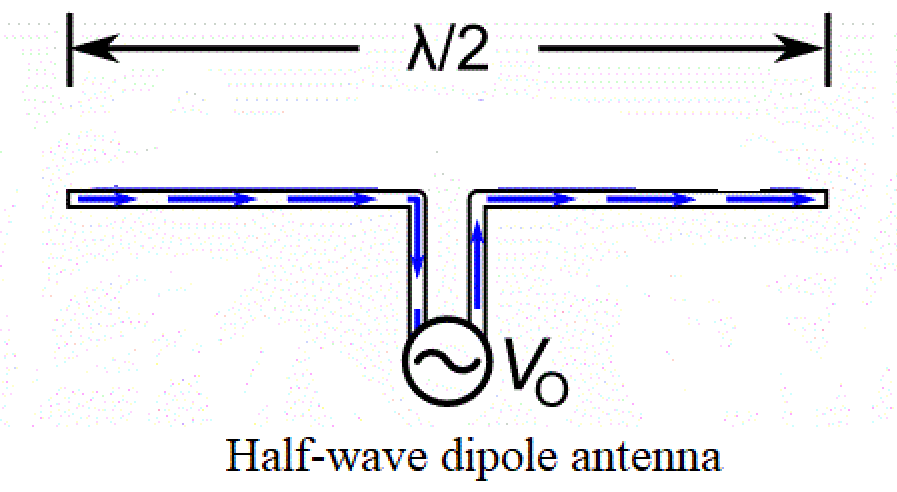
The length of the half wave dipole,
Substitute the value of
Thus, the answer is not given in the option.
Hence, the option (D) is correct.
Note: While solving the relation between the speed, wavelength and frequency of the wave, the speed of the wave is not given in the question. So, it is assumed to be equal to the speed of the light in the medium. And the SI unit of the frequency
Useful formula:
The length of the half wave dipole,
Where,
The relationship between speed, wavelength and frequency of the wave,
Where,
Given data:
The frequency of the wave,
Complete step by step solution:
The relationship between speed, wavelength and frequency of the wave,
Since, the normal wave moves with the speed of light. So,
Substitute the values of

The length of the half wave dipole,
Substitute the value of
Thus, the answer is not given in the option.
Hence, the option (D) is correct.
Note: While solving the relation between the speed, wavelength and frequency of the wave, the speed of the wave is not given in the question. So, it is assumed to be equal to the speed of the light in the medium. And the SI unit of the frequency
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




