Question

Length of the focal chord of the parabola ${{y}^{2}}=4ax$ at the distance p from the vertex is:
A.$\dfrac{2{{a}^{3}}}{p}$
B.$\dfrac{{{a}^{3}}}{{{p}^{2}}}$
C.$\dfrac{4{{a}^{3}}}{{{p}^{2}}}$
D.$\dfrac{{{p}^{2}}}{a}$

Answer 1

Hint: Take 2 points on parabola as $\left( at_{1}^{2},2a{{t}_{1}} \right)$ and $\left( at_{2}^{2},2a{{t}_{2}} \right)$ . use basic formula and find the length of PQ and take length of focal chord PQ as c. Now, find the equation of line PQ. Substitute the value you get in the expression of length of focal chord ‘c’ and get the value of c.

Complete step-by-step answer:
We have been given the equation of parabola as ${{y}^{2}}=4ax$ . We need to find the focal chord of the parabola at a distance p from the vertex. Let us take 2 points on the parabola as P and Q. Now let us consider the coordinates as $P\left( at_{1}^{2},2a{{t}_{1}} \right)$ and $Q\left( at_{2}^{2},2a{{t}_{2}} \right)$ . $O\left( a,o \right)$ is the focus of the parabola.

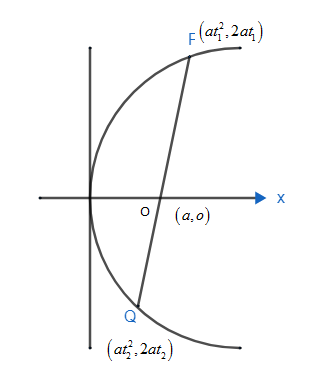
We know the formula for finding slope of line as, $m=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}$ .
Here the slope of OP is equal to the slope of OQ.
i.e. ${{t}_{1}}{{t}_{2}}=-1$ i.e. Slope of OP = Slope of OQ
$\Rightarrow {{t}_{2}}=\dfrac{-1}{{{t}_{1}}}$ .
Hence in the coordinate of Q put ${{t}_{2}}=\dfrac{-1}{{{t}_{1}}}$
$\therefore Q\left( a{{\left( -\dfrac{1}{{{t}_{1}}} \right)}^{2}},2a\left( -\dfrac{1}{{{t}_{1}}} \right) \right)=Q\left( \dfrac{a}{t_{1}^{2}},-\dfrac{2a}{{{t}_{1}}} \right)$ respectively.
Let’s assume ${{t}_{1}}=\dfrac{-1}{{{t}_{2}}}=t$.
Let the length of the focal chord be taken a c.
The distance of PQ can be found by using the formula, distance$\sqrt{{{\left( {{x}_{2}}-{{x}_{1}} \right)}^{2}}+{{\left( {{y}_{2}}-{{y}_{1}} \right)}^{2}}}$
Put $\left( {{x}_{2}},{{y}_{2}} \right)=\left( a{{t}^{2}},2at \right)$, \[\left( {{x}_{1}},{{y}_{1}} \right)=\left( \dfrac{a}{{{t}^{2}}},-\dfrac{2a}{t} \right)\].
Distance PQ\[=\sqrt{{{\left( a{{t}^{2}}-\dfrac{a}{{{t}^{2}}} \right)}^{2}}+{{\left( 2at+\dfrac{2a}{t} \right)}^{2}}}=c\]
Simplifying we get \[\left| \overline{PQ} \right|=\sqrt{{{a}^{2}}{{\left( {{t}^{2}}-\dfrac{1}{{{t}^{2}}} \right)}^{2}}+{{\left( 2a \right)}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}}=c\Rightarrow \sqrt{{{a}^{2}}{{\left( t-\dfrac{1}{t} \right)}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}+4{{a}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}}=c\]
We know that ${{a}^{2}}-{{b}^{2}}=\left( a+b \right)\left( a-b \right)$. Similarly, ${{\left( {{t}^{2}}-\dfrac{1}{{{t}^{2}}} \right)}^{2}}={{\left( t-\dfrac{1}{t} \right)}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}$
$\Rightarrow \left| \overline{PQ} \right|=\sqrt{{{a}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}\left\{ {{\left( t-\dfrac{1}{t} \right)}^{2}}+4 \right\}}=c$ [taking ${{a}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}$common both the terms].
$\Rightarrow \sqrt{{{a}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}\left( {{t}^{2}}+\dfrac{1}{{{t}^{2}}}-2+4 \right)}=c$
$\Rightarrow \sqrt{{{a}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}\left( {{t}^{2}}+\dfrac{1}{{{t}^{2}}}+2 \right)}=c$ we know ${{a}^{2}}+{{b}^{2}}+2ab={{\left( a+b \right)}^{2}}$
Similarly, $\sqrt{{{a}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{2}}}=c\Rightarrow \sqrt{{{a}^{2}}{{\left( t+\dfrac{1}{t} \right)}^{4}}}=c$
Hence it become, $a{{\left( t+\dfrac{1}{t} \right)}^{2}}=c$ ……………… (1)
Now, it is said that the length of the focal chord is at a distance of P from the vertex. Now, let us find the equation of PQ.
Equation of PQ can be found by using the formula, where
$\left( {{x}_{2}},{{y}_{2}} \right)=\left( a{{t}^{2}},2at \right)$, \[\left( {{x}_{1}},{{y}_{1}} \right)=\left( \dfrac{a}{{{t}^{2}}},-\dfrac{2a}{t} \right)\].
So, $y-{{y}_{1}}=m\left( x-{{x}_{1}} \right)$
Where m = slope of line PQ $=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}=\dfrac{2at+\dfrac{2a}{t}}{a{{t}^{2}}-\dfrac{a}{{{t}^{2}}}}=\dfrac{2a\left( t+\dfrac{1}{t} \right)}{a\left( {{t}^{2}}-\dfrac{1}{{{t}^{2}}} \right)}=\dfrac{2\left( t+\dfrac{1}{t} \right)}{\left( t+\dfrac{1}{t} \right)\left( t-\dfrac{1}{t} \right)}=\dfrac{2}{t-\dfrac{1}{t}}$ .
i.e. $m=\dfrac{2}{t-\dfrac{1}{t}}$
therefore, equation of line PQ is $y+\dfrac{2a}{t}=\dfrac{2}{t-\dfrac{1}{t}}\left( x-\dfrac{a}{{{t}^{2}}} \right)$
Let us simplify the expression in
$\begin{align}
  & \Rightarrow y+\dfrac{2a}{t}=\dfrac{2t}{{{t}^{2}}-1}\left( x-\dfrac{a}{{{t}^{2}}} \right) \\
 & \Rightarrow \left( {{t}^{2}}-1 \right)\left( {{t}^{2}}y+2at \right)=2t\left( {{t}^{2}}x-a \right) \\
 & \Rightarrow \left( {{t}^{2}}-1 \right)\left( ty+2a \right)=2\left( {{t}^{2}}x-a \right) \\
 & \Rightarrow t\left( {{t}^{2}}-1 \right)y-2{{t}^{2}}x+a\left( 2{{t}^{2}}-2+2 \right)=0 \\
 & \Rightarrow \left( {{t}^{2}}-1 \right)y-2tx+2at=0 \\
\end{align}$
This is the equation of the focal chord.
It is said that the distance from vertex is p. we know the coordinate of vertex as $\left( 0,0 \right)$ .Thus by distance formula, here $\left( x,y \right)=0$
\[p=\dfrac{\left| A{{x}_{1}}+B{{y}_{1}}+c \right|}{\sqrt{{{A}^{2}}+{{B}^{2}}}}=\dfrac{\left| 26\times 0-\left( {{t}^{2}}-1 \right)\times 0-2at \right|}{\sqrt{{{\left( -2t \right)}^{2}}+{{\left( {{t}^{2}}-1 \right)}^{2}}}}=\dfrac{\left| -2at \right|}{\sqrt{4{{t}^{2}}+{{\left( {{t}^{2}}-1 \right)}^{2}}}}\]
Now, let us simplify this further, we know that ${{a}^{2}}-{{b}^{2}}=\left( a-b \right)\times \left( a+b \right)$.\[\begin{align}
  & p=\dfrac{\left| -2at \right|}{\sqrt{4{{t}^{2}}+{{\left( {{t}^{2}}-1 \right)}^{2}}}}=\dfrac{2at}{\sqrt{{{\left( {{t}^{2}}+1 \right)}^{2}}}}=\dfrac{2at}{{{t}^{2}}+1} \\
 & \Rightarrow \dfrac{{{t}^{2}}+1}{t}=\dfrac{2a}{P} \\
 & \Rightarrow t+\dfrac{1}{t}=\dfrac{2a}{P} \\
\end{align}\]
Now, substitute this in (1)
$\begin{align}
  & a{{\left( t+\dfrac{1}{t} \right)}^{2}}=c\Rightarrow a{{\left( \dfrac{2a}{P} \right)}^{2}}=c \\
 & \Rightarrow c=\dfrac{4{{a}^{3}}}{{{P}^{2}}} \\
\end{align}$
Length of focal chord $c=\dfrac{4{{a}^{3}}}{{{P}^{2}}}$. Hence, we got the required length as $\dfrac{4{{a}^{3}}}{{{P}^{2}}}$.

Note: The length of a focal chord of a parabola varies inversely as the square of the distance from its vertex. If we have length of segments of focal chords as ${{l}_{1}}$ and ${{l}_{2}}$ then we can find the latus rectum as $\dfrac{4{{l}_{1}}{{l}_{2}}}{{{l}_{1}}+{{l}_{2}}}$.