Question

Let PQ be a chord of the parabola \[{{y}^{2}}=4x\]. A circle drawn with PQ as a diameter passes through the vertex V of the parabola. If Area of \[\Delta PVQ\] = 20 \[uni{{t}^{2}}\] then the co – ordinates of P are
(a)(-16, -8)
(b)(-16, 8)
(c)(16, -8)
(d)(16, 8)

Answer 1

Hint: Suppose points P and Q as \[\left( at_{1}^{2},2a{{t}_{1}} \right)\] and \[\left( at_{2}^{2},2a{{t}_{2}} \right)\] by using the parametric coordinates for \[{{y}^{2}}=4ax\] (put, a= 1). Angle formed in a semi – circle is \[{{90}^{\circ }}\] i.e. angle at vertex by PQ (diameter) is \[{{90}^{\circ }}\]. So, PV and VQ will be perpendicular to each other. Use area of triangle as,
\[=\dfrac{1}{2}\times \] base \[\times \] height

Complete step-by-step answer:
Use following results to solve the problem further: -
Product of slopes of two perpendicular lines is -1 and the distance between two points \[\left( {{x}_{1}},{{y}_{1}} \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)\] is given as
\[=\sqrt{{{\left( {{x}_{1}}-{{x}_{2}} \right)}^{2}}+{{\left( {{y}_{1}}-{{y}_{2}} \right)}^{2}}}\]

Given equation of parabola in the problem is,
\[{{y}^{2}}=4x-(1)\]
We know PQ is a chord of the parabola \[{{y}^{2}}=4ax\] and a circle is drawn with PQ as a diameter and circle is passing through the vertex V of the parabola.
We know \[{{y}^{2}}=4ax\] is symmetric about the x – axis with vertex as (0, 0). So, the coordinate of point V is (0, 0).
So, we can draw diagram with the help of given information as: -

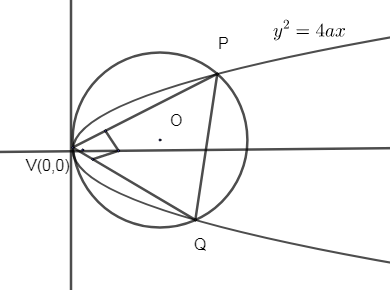
As we know, the angle formed in a semi – circle is \[{{90}^{\circ }}\]. i.e. angle formed by diameter is \[{{90}^{\circ }}\]. As PQ is a diameter of the circle with center O in the diagram, so \[\angle PVQ={{90}^{\circ }}\] as per the property of the semi – circle.
So, we know area of triangle is given as,
\[=\dfrac{1}{2}\times \] base \[\times \] height – (2)
Hence, area of \[\Delta PVQ\],
\[=\dfrac{1}{2}\times \left( PV \right)\times \left( VQ \right)-(3)\]
Let us suppose the coordinates of points P and Q on the parabola \[{{y}^{2}}=4x\] are \[\left( t_{1}^{2},2{{t}_{1}} \right)\] and \[\left( t_{2}^{2},2{{t}_{2}} \right)\] [We know the parametric coordinates for \[{{y}^{2}}=4x\] are \[\left( a{{t}^{2}},2at \right)\]].
As VP and VQ are perpendicular to each other, it means the product of slopes of them will be -1, because of the relation.
Product of slopes of two perpendicular lines = -1. – (4)
We know slope of a line with the help of two coordinates \[\left( {{x}_{1}},{{y}_{1}} \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)\] is given by relation,
Slope \[=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}-(5)\]
Hence, slope of VP \[=\dfrac{2{{t}_{1}}-0}{t_{1}^{2}-0}=\dfrac{2{{t}_{1}}}{t_{1}^{2}}\]
Slope of VP \[=\dfrac{2}{{{t}_{1}}}\]
Similarly, slope of VQ \[=\dfrac{2}{{{t}_{2}}}\]
Hence, we get from equation (4) as,
\[\begin{align}
  & \dfrac{2}{{{t}_{1}}}\times \dfrac{2}{{{t}_{2}}}=-1 \\
 & {{t}_{1}}{{t}_{2}}=-4-(6) \\
\end{align}\]
Now, we need to calculate the distances VP and VQ to get the area from the relation (3).
We know the distance between two points \[\left( {{x}_{1}},{{y}_{1}} \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)\] can be given by distance formula as,
\[=\sqrt{{{\left( {{x}_{1}}-{{x}_{2}} \right)}^{2}}+{{\left( {{y}_{1}}-{{y}_{2}} \right)}^{2}}}-(7)\]
Hence, length of VP
\[\begin{align}
  & VP=\sqrt{{{\left( 2{{t}_{1}}-0 \right)}^{2}}+{{\left( t_{1}^{2}-0 \right)}^{2}}} \\
 & VP=\sqrt{4t_{1}^{2}+t_{1}^{4}}-(8) \\
\end{align}\]
Similarly, length of VQ
\[\begin{align}
  & VQ=\sqrt{{{\left( 2{{t}_{2}}-0 \right)}^{2}}+{{\left( t_{2}^{2}-0 \right)}^{2}}} \\
 & VQ=\sqrt{4t_{2}^{2}+t_{2}^{4}}-(9) \\
\end{align}\]
Now, we know area of \[\Delta PVQ\] can be given from the equations (3), (8) and (9) as,
Area of \[\Delta PVQ\] \[=\dfrac{1}{2}\times \sqrt{4t_{1}^{2}+t_{1}^{4}}\times \sqrt{4t_{2}^{2}+t_{2}^{4}}\]
Area of \[\Delta PVQ\] \[=\dfrac{1}{2}\times \sqrt{\left( 4t_{1}^{2}+t_{1}^{4} \right)\left( 4t_{2}^{2}+t_{2}^{4} \right)}\]
Area of \[\Delta PVQ\] \[=\dfrac{1}{2}\times \sqrt{16t_{1}^{2}t_{2}^{2}+4t_{1}^{2}t_{1}^{4}+4t_{1}^{4}t_{2}^{2}+t_{1}^{4}t_{2}^{4}}\]
Put, \[{{t}_{1}}{{t}_{2}}=-4\], from the equation (6). We get,
Area of \[\Delta PVQ\] = \[\dfrac{1}{2}\sqrt{16\times {{\left( -4 \right)}^{2}}+4t_{1}^{2}t_{2}^{2}\left( t_{2}^{2}+t_{1}^{2} \right)+{{\left( -4 \right)}^{4}}}\]
Area of \[\Delta PVQ\] \[=\dfrac{1}{2}\sqrt{256+4\times {{\left( -4 \right)}^{2}}\left( t_{1}^{2}+t_{2}^{2} \right)+256}\]
Area of \[\Delta PVQ\] \[=\dfrac{1}{2}\sqrt{512+64\left( t_{1}^{2}+t_{2}^{2} \right)}\]
We know the area of \[\Delta PVQ\] from the problem as 20 square units. So, we get,
\[\begin{align}
  & 20=\dfrac{1}{2}\sqrt{512+64\left( t_{1}^{2}+t_{2}^{2} \right)} \\
 & 40=\sqrt{512+64\left( t_{1}^{2}+t_{2}^{2} \right)} \\
\end{align}\]
Squaring both sides of the above equation, we get,
\[\begin{align}
  & 1600=512+64\left( t_{1}^{2}+t_{2}^{2} \right) \\
 & \dfrac{1600-512}{64}=t_{1}^{2}+t_{2}^{2} \\
 & \dfrac{1088}{64}=t_{1}^{2}+t_{2}^{2} \\
 & 17=t_{1}^{2}+t_{2}^{2} \\
\end{align}\]
Put, \[{{t}_{2}}=\dfrac{-4}{{{t}_{1}}}\] from the equation (6). We get,
\[\begin{align}
  & 17=t_{1}^{2}+{{\left( \dfrac{-4}{{{t}_{1}}} \right)}^{2}} \\
 & 17=t_{1}^{2}+\dfrac{16}{t_{1}^{2}} \\
\end{align}\]
Adding 8 to both sides, we get,
\[\begin{align}
  & 17+8=t_{1}^{2}+\dfrac{16}{t_{1}^{2}}+8 \\
 & 25={{\left( {{t}_{1}} \right)}^{2}}+{{\left( \dfrac{4}{{{t}_{1}}} \right)}^{2}}+2\times \dfrac{4}{{{t}_{1}}}\times {{t}_{1}} \\
\end{align}\]
So, we get,
\[{{\left( {{t}_{1}}+\dfrac{4}{{{t}_{1}}} \right)}^{2}}=25\]
Taking square root to both sides, we get,
\[{{t}_{1}}+\dfrac{4}{{{t}_{1}}}=5\] or \[{{t}_{1}}+\dfrac{4}{{{t}_{1}}}=-5\]
Case 1: \[{{t}_{1}}+\dfrac{4}{{{t}_{1}}}=5\]
\[\begin{align}
  & t_{1}^{2}+4=5{{t}_{1}} \\
 & t_{1}^{2}-5{{t}_{1}}+4=0 \\
 & {{t}_{1}}-4{{t}_{1}}-{{t}_{1}}+4=0 \\
 & {{t}_{1}}\left( {{t}_{1}}-4 \right)-1\left( {{t}_{1}}-4 \right)=0 \\
 & \left( {{t}_{1}}-1 \right)\left( {{t}_{1}}-4 \right)=0 \\
\end{align}\]
\[{{t}_{1}}=1\] or \[{{t}_{1}}=4\]
Case 2: \[{{t}_{1}}+\dfrac{4}{{{t}_{1}}}=-5\]
\[\begin{align}
  & t_{1}^{2}+4=-5{{t}_{1}} \\
 & t_{1}^{2}+5{{t}_{1}}+4=0 \\
 & {{t}_{1}}+4{{t}_{1}}+{{t}_{1}}+4=0 \\
 & {{t}_{1}}\left( {{t}_{1}}+4 \right)+1\left( {{t}_{1}}+4 \right)=0 \\
 & \left( {{t}_{1}}+1 \right)\left( {{t}_{1}}+4 \right)=0 \\
\end{align}\]
Hence, coordinates of P, for case 1 are given as \[\left( t_{1}^{2},2{{t}_{1}} \right)\].
Case 1: \[{{t}_{1}}=1\]
Coordinates are (1, 2)
Or
\[{{t}_{1}}=4\]
Coordinates are (16, 8)
Case 2: \[{{t}_{1}}=-1\]
Coordinates are (1, -2)
Or
\[{{t}_{1}}=-4\]
Coordinates are (16, -8)
Hence options (c) and (d) are the possible coordinates of B.

Note: One may use the direct result that if two points are subtending a right angle at vertex (0, 0) then, \[{{t}_{1}}{{t}_{2}}=-4\]. Where points \[\left( at_{1}^{2},2a{{t}_{1}} \right)\] and \[\left( at_{2}^{2},2a{{t}_{2}} \right)\] for the parabola, \[{{y}^{2}}=4ax\].
Another approach for the question would be that we can use formula for calculating area of triangle in terms of the coordinates as
\[=\dfrac{1}{2}\left| \begin{matrix}
   {{x}_{1}} & {{y}_{1}} & 1 \\
   {{x}_{2}} & {{y}_{2}} & 1 \\
   {{x}_{3}} & {{y}_{3}} & 1 \\
\end{matrix} \right|\]
Where \[\left( {{x}_{1}},{{y}_{1}} \right),\left( {{x}_{2}},{{y}_{2}} \right),\left( {{x}_{3}},{{y}_{3}} \right)\] are coordinates of vertices of any triangle.