
Let the refractive index of a denser medium with respect to a rare medium be
(A)
(B)
(C)
(D)
Answer
140.7k+ views
Hint: To find the angle of incidence we have to learn about the total internal reflection and its condition and about the critical angle we should also have knowledge about the Snell’s law to relate the critical angle with the angle of refraction and incidence.
Complete step by step answer
Angle of incidence is defined as the angle between a ray incident on a surface and the line perpendicular to the surface at the point of incidence.
When a ray of light hits a smooth polished surface, the light ray bounces back, it is called the reflection. The angle at which it bounce back is known as the angle of reflection
When light waves move from one medium to another, there is a change in the direction of waves due to the obstacles, so they bend and continue to pass it is known as refraction. The angle at which it bends is known as angle of refraction.
The relation between the angle of incidence and angle of refraction is given by Snell’s law
Snell's law states that the ratio of the sines of the angles of incidence to angle of refraction is equivalent to the ratio of phase velocities in the two media, or to the reciprocal of the ratio of the refractive indices
Where,
Sin i is the angle of incidence
Sin r is the angle of refraction
This relationship between the angles of incidence and refraction and the refractive indices of the two media is known as Snell's Law.
The critical angle is the angle of incidence beyond which light rays passing through a denser medium to the surface of a less dense medium are no longer refracted but totally reflected.
The critical angle is given by
Here
Hence here the total internal reflection takes place.
Given,
The refractive index of a denser medium with respect to a rare medium be
The critical angle is
The angle between refracted and reflected ray is
The angle of incidence is A
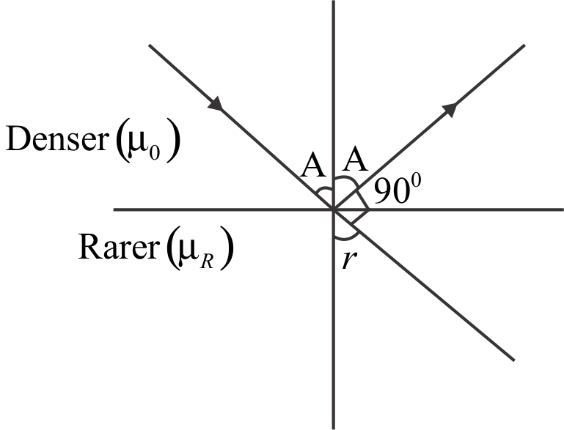
From the diagram
The angle of refraction is
Critical angle is
We have seen that
The critical angle becomes
Substitute the known values
Hence the correct answer is option (A)
Note In the diagram we have noted an angle as A other than the incident angle it is the angle of reflection. The angle of reflection is also A because the angle of reflection is equal to the angle of incidence in total internal reflection.
Complete step by step answer
Angle of incidence is defined as the angle between a ray incident on a surface and the line perpendicular to the surface at the point of incidence.
When a ray of light hits a smooth polished surface, the light ray bounces back, it is called the reflection. The angle at which it bounce back is known as the angle of reflection
When light waves move from one medium to another, there is a change in the direction of waves due to the obstacles, so they bend and continue to pass it is known as refraction. The angle at which it bends is known as angle of refraction.
The relation between the angle of incidence and angle of refraction is given by Snell’s law
Snell's law states that the ratio of the sines of the angles of incidence to angle of refraction is equivalent to the ratio of phase velocities in the two media, or to the reciprocal of the ratio of the refractive indices
Where,
Sin i is the angle of incidence
Sin r is the angle of refraction
This relationship between the angles of incidence and refraction and the refractive indices of the two media is known as Snell's Law.
The critical angle is the angle of incidence beyond which light rays passing through a denser medium to the surface of a less dense medium are no longer refracted but totally reflected.
The critical angle is given by
Here
Hence here the total internal reflection takes place.
Given,
The refractive index of a denser medium with respect to a rare medium be
The critical angle is
The angle between refracted and reflected ray is
The angle of incidence is A

From the diagram
The angle of refraction is
Critical angle is
We have seen that
The critical angle becomes
Substitute the known values
Hence the correct answer is option (A)
Note In the diagram we have noted an angle as A other than the incident angle it is the angle of reflection. The angle of reflection is also A because the angle of reflection is equal to the angle of incidence in total internal reflection.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Young's Double Slit Experiment Step by Step Derivation

Difference Between Circuit Switching and Packet Switching

Difference Between Mass and Weight

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Maths Paper Pattern 2025 – Marking, Sections & Tips

Sign up for JEE Main 2025 Live Classes - Vedantu

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Degree of Dissociation and Its Formula With Solved Example for JEE

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main




