
How much longer is
Answer
470.1k+ views
1 likes
Hint: Firstly, take both the quantities and compare which is larger. Then write them in a subtraction equation and evaluate using the LCM method or else by cross-multiplying the fractions and then subtracting the second number from the first to get the numerator part and multiply the denominators to get a common denominator.
Complete step-by-step answer:
Given quantities are,
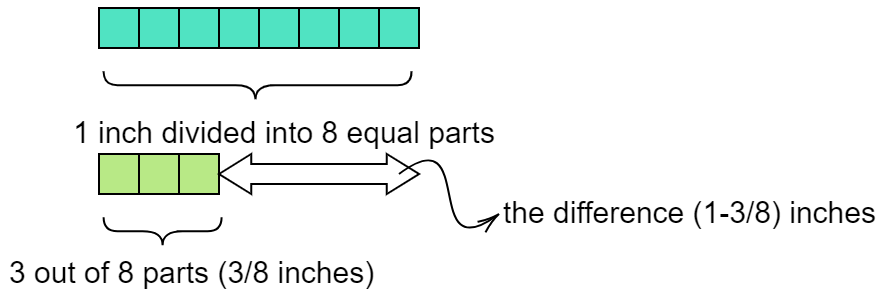
By comparing the quantities, we can say that
Since we must find how much is left of the first quantity after removing the second quantity, we shall write a subtraction equation.
We can write
Now cross-multiply the fractions and then subtract the numerators and then multiply the denominators to get a common denominator.
On further evaluation,
Which is equal to,
Additional Information: Whenever there are different denominators in a subtraction equation, the first step must be to convert it in such a way to get the same denominators. Then only we should proceed to the subtraction operation. The quantity which is given in the question is an Inch which is a unit of length.
Note:
The above question can also be solved using LCM (least common multiples) method.
Firstly, write both the quantities in fraction form.
Now, write the subtraction equation for the both quantities.
Now, find the multiples for the denominators
For
For
On comparing both the multiples, the LCM of
Therefore,
On further evaluation,
Complete step-by-step answer:
Given quantities are,

By comparing the quantities, we can say that
Since we must find how much is left of the first quantity after removing the second quantity, we shall write a subtraction equation.
We can write
Now cross-multiply the fractions and then subtract the numerators and then multiply the denominators to get a common denominator.
On further evaluation,
Which is equal to,
Additional Information: Whenever there are different denominators in a subtraction equation, the first step must be to convert it in such a way to get the same denominators. Then only we should proceed to the subtraction operation. The quantity which is given in the question is an Inch which is a unit of length.
Note:
The above question can also be solved using LCM (least common multiples) method.
Firstly, write both the quantities in fraction form.
Now, write the subtraction equation for the both quantities.
Now, find the multiples for the denominators
For
For
On comparing both the multiples, the LCM of
Therefore,
On further evaluation,
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




