
What is meant by the refractive index?
Answer
473.4k+ views
Hint : We will try to understand the term refractive index which is defined as the ratio of the velocity of light in a vacuum to the velocity of light in the given medium. It is also defined as the measure of the bending of light when it passes from one medium to another medium which is also known as an index of refraction.
Complete step by step answer
We will start with the law of refraction which states that the incident ray, refracted ray, and normal all lie in the same plane. Given below is the figure for the example of the refraction of light.
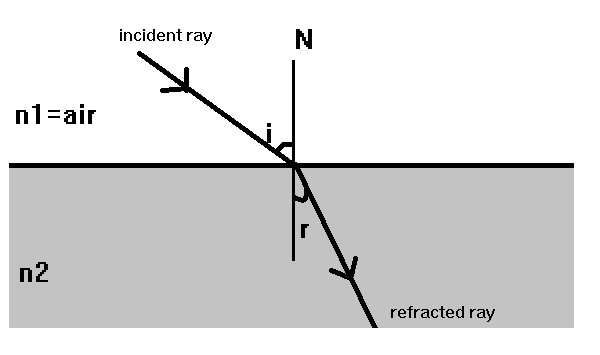
Here $ i $ is the angle of incidence which is the angle between the incident ray and normal $ N $ to the medium, $ r $ is the angle of refraction which is the angle between the refraction ray and normal $ N $ to the medium, and $ {\eta _1} $ , $ {\eta _2} $ can be defined as refractive indices of the given respective medium.
Now the refractive index can be defined as the ratio of the sine of the angle of incidence $ i $ to the sine angle of refraction $ r $
Hence the refractive index $ \eta $ can be given as
$ \Rightarrow \eta = \dfrac{{\sin i}}{{\sin r}} $
The Refractive index as discussed can also be defined as the ratio of the velocity of light $ c $ in a vacuum and the velocity of the light $ v $ in a given medium. Hence
$ \Rightarrow \eta = \dfrac{c}{v} $ .
Note
The velocity of the light in a vacuum is always constant which can be given as $ c = 2.99 \times {10^8}\dfrac{m}{s} $ . Hence the refractive index of any medium always depends on the velocity of light in that medium. The refractive index of air is $ {\eta _{air}} = 1.0003 $ while the refractive index of some medium such as water is $ {\eta _{water}} = 1.333 $ and for crown glass $ {\eta _{crownglass}} = 1.517 $ and for a diamond it is $ {\eta _{diamond}} = 2.417 $ .
Complete step by step answer
We will start with the law of refraction which states that the incident ray, refracted ray, and normal all lie in the same plane. Given below is the figure for the example of the refraction of light.

Here $ i $ is the angle of incidence which is the angle between the incident ray and normal $ N $ to the medium, $ r $ is the angle of refraction which is the angle between the refraction ray and normal $ N $ to the medium, and $ {\eta _1} $ , $ {\eta _2} $ can be defined as refractive indices of the given respective medium.
Now the refractive index can be defined as the ratio of the sine of the angle of incidence $ i $ to the sine angle of refraction $ r $
Hence the refractive index $ \eta $ can be given as
$ \Rightarrow \eta = \dfrac{{\sin i}}{{\sin r}} $
The Refractive index as discussed can also be defined as the ratio of the velocity of light $ c $ in a vacuum and the velocity of the light $ v $ in a given medium. Hence
$ \Rightarrow \eta = \dfrac{c}{v} $ .
Note
The velocity of the light in a vacuum is always constant which can be given as $ c = 2.99 \times {10^8}\dfrac{m}{s} $ . Hence the refractive index of any medium always depends on the velocity of light in that medium. The refractive index of air is $ {\eta _{air}} = 1.0003 $ while the refractive index of some medium such as water is $ {\eta _{water}} = 1.333 $ and for crown glass $ {\eta _{crownglass}} = 1.517 $ and for a diamond it is $ {\eta _{diamond}} = 2.417 $ .
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
The probability that a leap year will have only 52 class 12 maths CBSE

Describe the poetic devices used in the poem Aunt Jennifers class 12 english CBSE

And such too is the grandeur of the dooms We have imagined class 12 english CBSE

What does the god that failed refer to class 12 english CBSE

Which country did Danny Casey play for class 12 english CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE




