
What is the moment of a rod of mass M, length l about an axis perpendicular to it through one end?
Answer
507.6k+ views
Hint: We know that moment of inertia of mass M, length l about the axis passing through its centre can be given by the formula,
Formula used:
Complete step by step answer:
In question we are asked to find the moment of inertia of a rod of mass M and length l, along the axis perpendicular to its end, so first of all we will draw a figure for our simplicity,
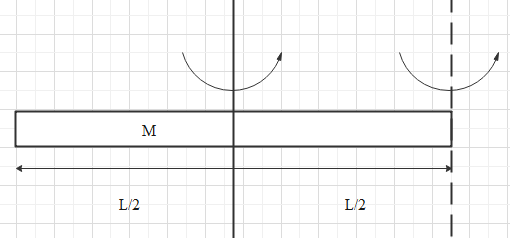
Now, the moment of inertia of rod along the axis passing through its centre can be given by the formula,
Where, M is mass of rod and l is length of rod
Now, to find the moment of inertia along axis perpendicular to one end of rod can be given by parallel axis theorem, which can be given as,
Where, m is mass of rod, a is distance of axis from centre and I is moment of inertia along centre axis.
So, from it can be said that axis passing through one of the ends is at distance
Thus, the moment of inertia of rod along the axis perpendicular to one of its ends is
Note: One can also calculate this by considering the moment of inertia as half of over all moment of inertia at one of the ends, mass becomes double as everything is concentrated at one point and length is considered as double, and by applying the formula of moment of inertia of rod passing through centre of one end, so the expression will be,
Formula used:
Complete step by step answer:
In question we are asked to find the moment of inertia of a rod of mass M and length l, along the axis perpendicular to its end, so first of all we will draw a figure for our simplicity,

Now, the moment of inertia of rod along the axis passing through its centre can be given by the formula,
Where, M is mass of rod and l is length of rod
Now, to find the moment of inertia along axis perpendicular to one end of rod can be given by parallel axis theorem, which can be given as,
Where, m is mass of rod, a is distance of axis from centre and I is moment of inertia along centre axis.
So, from it can be said that axis passing through one of the ends is at distance
Thus, the moment of inertia of rod along the axis perpendicular to one of its ends is
Note: One can also calculate this by considering the moment of inertia as half of over all moment of inertia at one of the ends, mass becomes double as everything is concentrated at one point and length is considered as double, and by applying the formula of moment of inertia of rod passing through centre of one end, so the expression will be,
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




