Question

Moment of inertia of a disc about its own axis is\[I\]. Its moment of inertia about a tangential axis in its plane is
A. \[\dfrac{5I}{2}\]
B. 3I
C. \[\dfrac{3I}{2}\]
D. 2I

Answer 1

- Hint: Write the formula for moment of inertia of disc about its own axis. Calculate the moment of inertia about its diameter using the perpendicular axis theorem. Now use this formula of the moment of inertia of a disc about its diameter to calculate the moment of inertia about a tangential axis using parallel axis theorem.

Complete step-by-step solution -

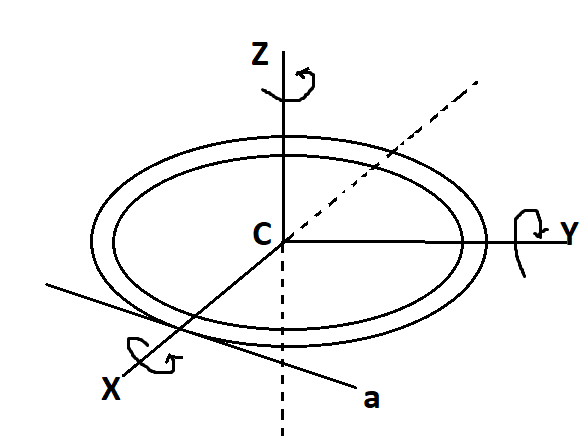
The moment of inertia about the z-axis is given by ${{I}_{z}}$ which is passing through the center of mass and perpendicular to the plane is given by ${{I}_{z}}={{I}_{c}}$. ${{I}_{x}}\And {{I}_{y}}$are moments of inertia of disc about diameter along x and y axes respectively.
Therefore by perpendicular axis theorem, we get
\[{{I}_{z}}={{I}_{x}}+{{I}_{y}}\]
But \[{{I}_{z}}=I\text{ and }{{I}_{x}}={{I}_{y}}={{I}_{d}}\]
Put the value we get,
\[\begin{align}
  & I=2{{I}_{d}} \\
 & {{I}_{d}}=\dfrac{I}{2} \\
\end{align}\]
\[\begin{align}
  & \text{we know that moment of inertia about center of disc is }{{\text{I}}_{c}}=I=\dfrac{M{{R}^{2}}}{2} \\
 & M{{R}^{2}}=2I \\
\end{align}\]
We know that moment of inertia about a is ${{I}_{a}}$. Now by using parallel and perpendicular axis theorem.
We have,
$\begin{align}
  & {{I}_{a}}={{I}_{y}}+M{{R}^{2}} \\
 & {{I}_{a}}={{I}_{d}}+M{{R}^{2}} \\
 & {{I}_{a}}=\dfrac{I}{2}+2I=\dfrac{5}{2}I \\
\end{align}$
Moment of inertia about a tangential axis in its plane is $\dfrac{5}{2}I$

Additional Information:
Perpendicular axis theorem states that moment of inertia of a plane lamina about an axis perpendicular to its plane is equal to the sum of its moment of inertia about two mutually perpendicular axes concurrent with perpendicular axis and lying in the plane of the laminar body.
Parallel axis theorem states that moment of inertia about of a body about any axis is equal to the sum of its moment of inertia about a parallel axis passing through its center of mass and product of its mass and square of the perpendicular distance between the two parallel axes.
Hence, option (A) is correct.

Note: Moment of inertia of disc about x and y-axis are the same only in case of the disc. Note that the thickness of the disc should be uniform. Do not get confused between the theorem of parallel axis and the theorem of perpendicular axis theorem. Moment of inertia about a tangential axis depends on the mass of disc, the radius of disc or diameter as well as a moment of inertia of disc about center.