
Now use numbers 1-6 to make your own magic triangle.

Rule: Numbers on each side must add up to 10.

Answer
475.5k+ views
Hint: Magic triangle is a triangle with vacant places to fill with numbers on corners and on each side. The property of a magic triangle is that the sum of all numbers on each side must be the same. One of the methods we can try is the trial and error as the numbers to be used is smaller in count. Thus we will try to start with a particular corner and then fill one by one checking whether each side adds up to 10 or not.
Complete step-by-step answer:
Step 1: Let us start by naming the triangle as triangle ABC. We have 6 vacant places which are to be filled with numbers 1-6 having 3 corners. Then the triangle with number 1 filled on corner A will be as below:
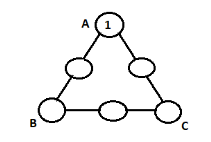
Step 2: As 6 and 4 together add up to 10, we cannot put them on the same side of the triangle. Thus if we choose 6 on side AB, 4 should be placed on side BC or AC. Filling 6 on middle position of side AB, clearly corner B will be filled by 10-(6+1)=3. Thus the triangle will look like,
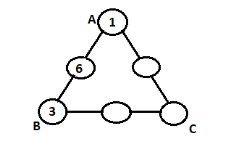
Step 3: Now if we fill 4 on side BC, then 3+4 add up to 7 and the rest of the vacant place of side BC should be filled by 10-7 which is 3 which have already been used for corner B. Thus 4 can only be placed on the middle vacancy of side AC. Then corner C will be filled by 10-(4+1)=5. Thus the triangle will look like,
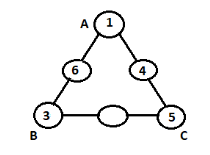
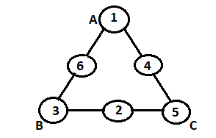
Step 4: Now the only remaining position is middle vacancy of side BC and the remaining number is 2. Let us check the sum of numbers of side BC if 2 is filled, which will be 3+2+5=10. Thus our aim is achieved. Thus the final form of triangle will be,
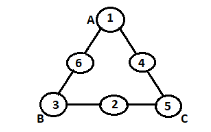
Final answer: The magic triangle using numbers 1-6 with sides adding upto 10 is :
Note: Solving time may increase if we try to fill the numbers randomly. Thus while placing each number, we must rule out the chance of placing other number on the same side.
As we have seen a magic triangle in the above solution, there are some other similar figures having the same property. One of which is Magic square which is a square split either by 9 vacant places inside of 16 according to the solution we require. Let us see one example of a 3*3 magic square:
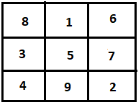
Above is a magic square with 9 vacant places filled with numbers 1-9 with each side and diagonal adding upto 15.
Complete step-by-step answer:
Step 1: Let us start by naming the triangle as triangle ABC. We have 6 vacant places which are to be filled with numbers 1-6 having 3 corners. Then the triangle with number 1 filled on corner A will be as below:

Step 2: As 6 and 4 together add up to 10, we cannot put them on the same side of the triangle. Thus if we choose 6 on side AB, 4 should be placed on side BC or AC. Filling 6 on middle position of side AB, clearly corner B will be filled by 10-(6+1)=3. Thus the triangle will look like,

Step 3: Now if we fill 4 on side BC, then 3+4 add up to 7 and the rest of the vacant place of side BC should be filled by 10-7 which is 3 which have already been used for corner B. Thus 4 can only be placed on the middle vacancy of side AC. Then corner C will be filled by 10-(4+1)=5. Thus the triangle will look like,

Step 4: Now the only remaining position is middle vacancy of side BC and the remaining number is 2. Let us check the sum of numbers of side BC if 2 is filled, which will be 3+2+5=10. Thus our aim is achieved. Thus the final form of triangle will be,

Final answer: The magic triangle using numbers 1-6 with sides adding upto 10 is :

Note: Solving time may increase if we try to fill the numbers randomly. Thus while placing each number, we must rule out the chance of placing other number on the same side.
As we have seen a magic triangle in the above solution, there are some other similar figures having the same property. One of which is Magic square which is a square split either by 9 vacant places inside of 16 according to the solution we require. Let us see one example of a 3*3 magic square:

Above is a magic square with 9 vacant places filled with numbers 1-9 with each side and diagonal adding upto 15.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
The southernmost point of the Indian mainland is known class 7 social studies CBSE

List of coprime numbers from 1 to 100 class 7 maths CBSE

In his early days shivaji moved with AJat leaders BMawali class 7 social science CBSE

Write a summary of the poem the quality of mercy by class 7 english CBSE

How did Douglas overcome his fear of water class 7 english CBSE

Find HCF and LCM of 510 and 92 by applying the prime class 7 maths CBSE




