
What is the phase angle in a series RLC circuit at resonance?
A)
B)
C)
D)
E) None of the above
Answer
392.7k+ views
Hint: A series RLC is an AC circuit that is said to be in electrical resonance when the circuit power factor is unity i.e.,
The phase angle in a RLC circuit is given by
Complete step by step answer:
Let’s draw an AC circuit containing resistor, inductor and capacitor in series.
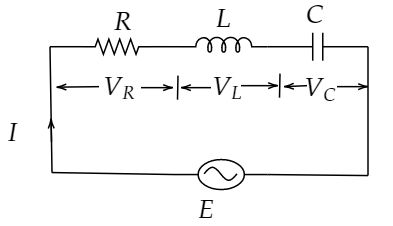
The voltage across resistor
The voltage across inductor
The voltage across capacitor
Consider
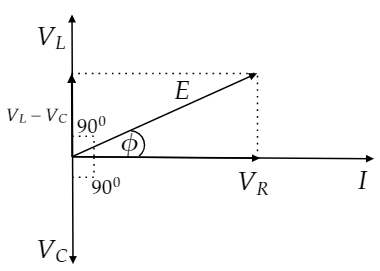
The resultant voltage in the phasor diagram is the applied voltage and it is given by
Or
Or
The quantity
The angle
Or
Or
At resonance, the impedance of the circuit is minimum and is equal to the resistance of the circuit.
i.e.,
Or
Further simplify
Now at resonance, phase angle
Or
Hence, the correct option is (C)
Note: Alternative method for solving the problem:
When the RLC series AC circuit is at resonance, the average power in the circuit is maximum.
Where
At resonance,
Therefore,
Or
The phase angle in a RLC circuit is given by
Complete step by step answer:
Let’s draw an AC circuit containing resistor, inductor and capacitor in series.

The voltage across resistor
The voltage across inductor
The voltage across capacitor
Consider

The resultant voltage in the phasor diagram is the applied voltage and it is given by
Or
Or
The quantity
The angle
Or
Or
At resonance, the impedance of the circuit is minimum and is equal to the resistance of the circuit.
i.e.,
Or
Further simplify
Now at resonance, phase angle
Or
Hence, the correct option is (C)
Note: Alternative method for solving the problem:
When the RLC series AC circuit is at resonance, the average power in the circuit is maximum.
Where
At resonance,
Therefore,
Or
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




