
Plot the graph of
Answer
445.8k+ views
Hint: To draw the graph of
Inverse of a function means a function which returns back the original value applied on the given function. Inverse of a particular function exists if the function is bijective.i.e., for each unique value from the domain of the function, we should have a corresponding unique value from the range of the function.
Complete step-by-step solution:
For a function
Another point to be noted is
Range of
Now, let us proceed with the question,
We have,
Let us assume
Using,
We get
For different values of
For
For
For
For
For
For
So, we can redefine the function
Now, using these inequalities we will draw the graph of
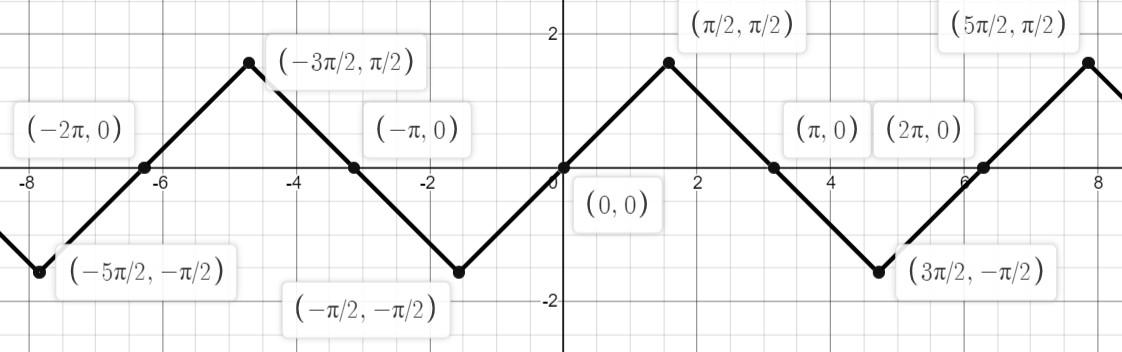
So, for the function
The graph is periodic with a fundamental period of
Note: While drawing the graph of
Inverse of a function means a function which returns back the original value applied on the given function. Inverse of a particular function exists if the function is bijective.i.e., for each unique value from the domain of the function, we should have a corresponding unique value from the range of the function.

Complete step-by-step solution:
For a function
Another point to be noted is
Range of
Now, let us proceed with the question,
We have,
Let us assume
Using,
We get
For different values of
For
For
For
For
For
For
So, we can redefine the function
Now, using these inequalities we will draw the graph of

So, for the function
The graph is periodic with a fundamental period of
Note: While drawing the graph of
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




