Question

PQR is a right-angled isosceles triangle, right-angled at Q, then prove that
\[P{{R}^{2}}=2P{{Q}^{2}}\]

Answer 1

Hint: In this question, we are given that the triangle is isosceles and so we can write PQ = QR. Then, apply the Pythagoras Theorem to the equation and put PQ = QR to get the desired results.

Complete step-by-step answer:
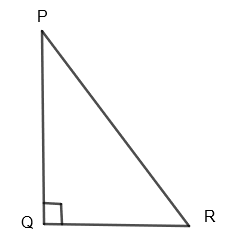
In this question, we are given that PQR is a right-angled isosceles triangle which is right-angled at Q, i.e. \[\angle PQR={{90}^{o}}\]. First of all, we will draw the figure,

In triangle PQR, \[\angle PQR={{90}^{o}}\], so PQ will be equal to QR and less than PR. So in the triangle PQR, we will apply Pythagoras Theorem. Before proceeding with the question, we need to know what Pythagoras theorem is. Pythagoras Theorem explains the relation between the sides of a right-angled triangle. This theorem is used for right-angled triangles. In this theorem, the sum of the squares of the two sides is the square of the third side. Generally, this theorem is used to find the third side of the triangle when two of the sides are given.
So now, we will apply this Pythagoras theorem in the triangle PQR, so we will write it as,
\[P{{Q}^{2}}+Q{{R}^{2}}=P{{R}^{2}}....\left( i \right)\]
As we know that PQR is an isosceles triangle, so we can say that PQ = QR. So now, instead of QR, we will substitute PQ in equation (i), we get,
\[P{{Q}^{2}}+P{{Q}^{2}}=P{{R}^{2}}\]
So, we get, \[2P{{Q}^{2}}=P{{R}^{2}}\]
Hence proved.

Note: Students should be careful while applying the Pythagoras theorem as they should know the conditions before applying it and also they should know the properties of the triangle so that it's easier to answer the question.