Question

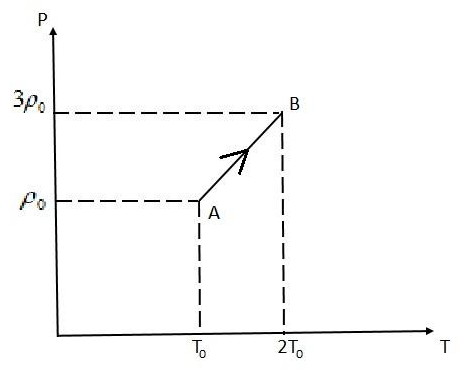
Pressure versus temperature graph of an ideal gas as shown in figure. Density of the gas at point A is \[{\rho _0}\]. Density of point B will be:

A. \[\dfrac{3}{4}{\rho _0}\]
B. \[\dfrac{3}{2}{\rho _0}\]
C. \[\dfrac{4}{3}{\rho _0}\]
D. \[\dfrac{4}{3}{\rho _0}\]

Answer 1

Hint: In this question, first of all observe the given graph carefully to write the sufficient and to get a clear idea of what we have to find. Then using the empirical form of ideal gas and the formulae of density and kinetic theory of gas we get the required answer.

Formulae Used:
1. \[\rho = \dfrac{m}{v}\]
2. \[v = \sqrt {\dfrac{{\gamma P}}{\rho }} \]

Complete answer:
The ideal gas law is also known as the general gas equation. It is the equation of state of a hypothetical ideal gas. The ideal gas law in empirical form is: \[PV = NRT\].
Since we are given that Density of the gas at point A is \[{\rho _0}\].
As we know that the ideal gas law in empirical form is: \[PV = NRT\]
Where P, V and T are the pressure, volume and temperature.
And N is the substance equal to total mass of the gas (m) divided by the molar mass (M)
R is the ideal gas constant.
Now by replacing N with \[\dfrac{m}{M}\], and subsequently introducing density by \[\rho = \dfrac{m}{v}\] we get,
\[PV = \dfrac{m}{M}RT\]
Or \[\dfrac{{PM}}{{RT}} = \rho \]
Also, we know that kinetic theory of gas is-
\[v = \sqrt {\dfrac{{\gamma P}}{\rho }} \]
From this, we are clear that the velocity of the sound in the gas is proportional to \[\sqrt T \] and \[\sqrt P \] where \[\rho \] is constant.
So, we can conclude that
\[\rho \] is proportional to \[\dfrac{P}{T}\].
In this ideal gas case at points A and B, \[\left( {\rho A = {{\left( {\dfrac{P}{T}} \right)}^A}} \right)\] and \[\left( {\rho B = {{\left( {\dfrac{P}{T}} \right)}^B}} \right)\]
Dividing both of them, we get
\[\dfrac{{\rho A}}{{\rho B}} = \dfrac{{{{\left( {\dfrac{P}{T}} \right)}^A}}}{{{{\left( {\dfrac{P}{T}} \right)}^B}}}\]
Here we have \[{\left( {\dfrac{P}{T}} \right)^A} = \dfrac{{{\rho _o}}}{{{T_o}}}\] and l\[{\left( {\dfrac{P}{T}} \right)^B} = \dfrac{{3{\rho _o}}}{{2{T_o}}}\]
So, we have
\[
   \Rightarrow \dfrac{{\rho A}}{{\rho B}} = \dfrac{{\dfrac{{{P_o}}}{{{T_o}}}}}{{\dfrac{{3{P_o}}}{{2{T_o}}}}} \\
   \Rightarrow \dfrac{{\rho A}}{{\rho B}} = \dfrac{2}{3} \\
\]
By cross-multiplying, we get
\[
   \Rightarrow 3\rho A = 2\rho B \\
   \Rightarrow \dfrac{3}{2}\rho A = \rho B \\
\]
We conclude at point B, density will be
\[\rho B = \dfrac{3}{2}{\rho _o}{\text{ }}\left[ {\because \rho A = {\rho _o}} \right]\]
Therefore, the correct option is B. \[\dfrac{3}{2}{\rho _0}\]

Note: In this question, we must know that kinetic theory of gas is a historically significant model of the thermodynamic behavior of gases, with which many principal concepts of thermodynamics were established. Also, one must be clear that (N) is calculated by dividing total mass of the gas (m) to the molar mass (M) in the ideal gas law i.e., \[PV = NRT\].