Question

Prove that if in two triangles, sides of one triangle are proportional to the sides of another triangle, then their corresponding angles are equal and hence the two triangles are similar. \[\]

Answer 1

Hint: We have two triangle $\Delta ABC$and $\Delta DEF$ with proportional sides$\dfrac{AB}{DE}=\dfrac{BC}{EF}=\dfrac{CA}{FD}$. We take points M on DE and N on DF such that$AB=DM,CA=DN$. We use the proportion and fact that when a line divides any two sides of a triangle in the same ratio then the line is parallel to the third side to prove $\Delta DMN\tilde{\ }\Delta DEF$. We then use the construction to prove $\Delta DMN\cong \Delta ABC$ and then prove $\angle A=\angle D,\angle B=\angle E,\angle C=\angle F$ and hence $\Delta ABC\sim \Delta DEF$.\[\]

Complete step by step answer:
As given in the question, we draw two triangles $\Delta ABC$ and $\Delta DEF$ such that the sides of triangle $\Delta ABC$ is proportional to the sides of other triangle $\Delta DEF$which means
\[\dfrac{AB}{DE}=\dfrac{BC}{EF}=\dfrac{CA}{FD}\]
We have to prove the angles opposite to the sides which are making the ratios are equal and hence the triangles are equal which means
\[\begin{align}
  & \angle A=\angle D,\angle B=\angle E,\angle C=\angle F \\
 & \Delta ABC\sim \Delta DEF \\
\end{align}\]
We take two points M on DE and N on DF such that
\[AB=DM,CA=DN.....\left( 1 \right)\]
We join the line segment MN.

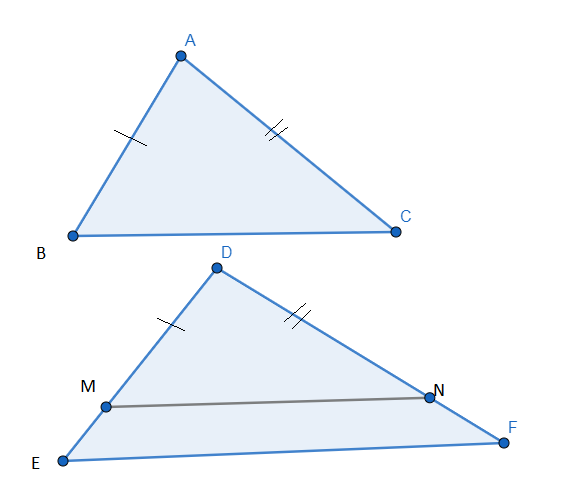
We are given
\[\dfrac{AB}{DE}=\dfrac{CA}{FD}\]
We put the values from equation (1) in the construction and have,
\[\Rightarrow \dfrac{DM}{DE}=\dfrac{DN}{FD}\left( \because AB=DM,CA=DN \right)\]
We take the reciprocal both side and then subtract 1 from both side of the equation to have,
 \[\begin{align}
  & \Rightarrow \dfrac{DE}{DM}=\dfrac{DF}{DN} \\
 & \Rightarrow \dfrac{DE}{DM}-1=\dfrac{DF}{DN}-1 \\
 & \Rightarrow \dfrac{DE-DM}{DM}=\dfrac{DF-DN}{DN} \\
 & \Rightarrow \dfrac{ME}{DM}=\dfrac{FN}{DN}......\left( 2 \right) \\
\end{align}\]

We know that when a line divides any two sides of a triangle in same ratio then the line is parallel to the third side. Here we have from (2) that the line MN divides DE and DF in the same ratio. So we have
\[MN||EF\]
We have $MN||EF$ and the transversal line DE which subtends corresponding angles
$\angle M=\angle E....\left( 3 \right)$
We have $MN||EF$ and the transversal line DF which subtends corresponding angles
\[\angle N=\angle F.....\left( 4 \right)\]
Let us observe the triangles $\Delta DMN$ and $\Delta DEF$. We have from (3) $\angle M=\angle E$, the common angle $\angle D$ and from (4) $\angle N=\angle F$. So by angle-angle-angle (AAA) criterion we have similar triangles$\Delta DMN\tilde{\ }\Delta DEF$. So the corresponding sides will be in proportion which means
\[\dfrac{DM}{DE}=\dfrac{DN}{DF}=\dfrac{MN}{EF}......\left( 5 \right)\]
We are given that
\[\dfrac{AB}{DE}=\dfrac{BC}{EF}=\dfrac{CA}{FD}\]
We replace the values from (1) in construction to have,
\[\dfrac{DM}{DE}=\dfrac{BC}{EF}=\dfrac{DN}{FD}.....\left( 6 \right)\]
We have from (5) and (6)$BC=MN$. Now let us observe the triangles $\Delta DMN$ and $\Delta ABC$. We have from construction $AB=DM,CA=DN$ and previously obtained $BC=MN$. So by side-side-side congruence we have$\Delta DMN\cong \Delta ABC$. Now the angles opposite to equal sides will be equal. So we have
\[\angle A=\angle D,\angle B=\angle M,\angle C=\angle N...\left( 7 \right)\]
We have (3) and (4)
\[\angle M=\angle E,\angle N=\angle F....\left( 8 \right)\]
So we have from (7) and (8)
\[\angle A=\angle D,\angle B=\angle E,\angle C=\angle F\]
So by angle-angle-angle similarity we have the proof as
\[\Delta ABC\sim \Delta DEF\]

Note: We have assumed in the construction that $DE > AB$ and the point M divide DE internally. We can take $DE < AB$ and have M divide DE externally. We note that all congruent triangles are similar but not all similar triangles are congruent. The other similarity condition is SAS where one angle of each triangle is equal and the adjacent sides are in proportion.