Question

Prove that \[\sin \theta < \theta < \tan \theta \] for \[\theta \in (0,\dfrac{\pi }{2})\].

Answer 1

Hint: We will consider a unit circle to prove our given equation. Then we consider a two triangle as per our own interest to find the values of \[\sin \theta \] and \[\tan \theta \].

Complete step-by-step answer:

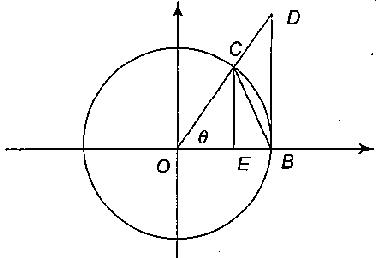
Here we are trying to consider a circle with unit radius with the centre at the origin.
Then we choose a point C on the circumference and another point B on the intersection of the circle and the x axis. Then we draw a perpendicular line from B which is parallel to y axis. We join OC with the perpendicular line in a point D.
Now, the point C is given at random,
From the figure, for any position of C on the circle,
Area of \[\Delta OBC\] < Area of sector OBC< Area of \[\Delta OBD\]
Using the corresponding formulae, the area of the triangle, \[\dfrac{1}{2}\].height. base
so, area of \[\Delta OBC\]\[ = \dfrac{1}{2}.CE.OB\], where CE is the height of the triangle of \[\Delta OBC\]
and area of \[\Delta OBD\]\[ = \dfrac{1}{2}.DB.OB\]
and the area of sector OBC, = \[\dfrac{1}{2}\]. Angle made by the sector OBC. \[ = \dfrac{1}{2}.\theta \]
 so, now we have,
\[ = \dfrac{1}{2}.CE.OB < \dfrac{1}{2}.\theta < \dfrac{1}{2}.DB.OB\]
As, for the triangle OCE, \[\sin \theta = \dfrac{{CE}}{{OC}} = \dfrac{{CE}}{1} = CE\]
And for the triangle ODB, \[\tan \theta = \dfrac{{DB}}{{OB}} = \dfrac{{DB}}{1} = DB\]
As, \[OC = OB = 1\]as this is a unit circle,
   \[ = \dfrac{1}{2}.\sin \theta .1 < \dfrac{1}{2}.\theta < \dfrac{1}{2}.\tan \theta .1\]
Cancelling out from \[\dfrac{1}{2}\] all of them, we get,
\[ = \sin \theta < \theta < \tan \theta \] for \[\theta \in \left( {0,\pi /2} \right)\]
Note: In some cases we have also \[\sin \theta = \theta \].The small-angle approximations can be used to approximate the values of the main trigonometric functions, provided that the angle in question is small and is measured in radians.