Question

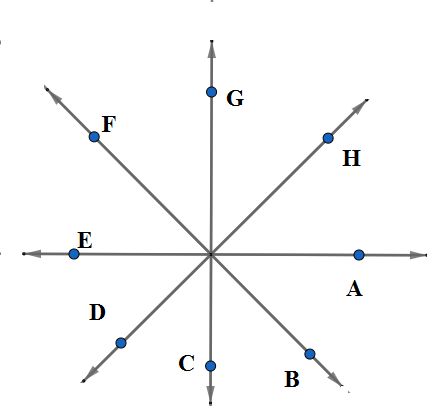
How many rays are represented in figure? Name them. \[\]

Answer 1

Hint: We see in the figure that there are 4 straight lines. We take one point in each line as the initial point and the one other point which is towards the arrow tip to get 2 rays in each straight line. We find how many rays there are in 4 lines.\[\]

Complete step by step answer:
We know that each ray has an initial point where the ray begins and we name the ray with another point on the ray excluding the initial point. If $ A $ is an initial point and $ B $ is any point on the ray then we name the ray as $ \overrightarrow{AB} $ .\[\]

We observe the given figure from the right hand side point A and keep looking in clockwise direction. We see that we have 4 straight lines which are
\[\overleftrightarrow {AE},\overleftrightarrow {BF},\overleftrightarrow {CG},\overleftrightarrow {DH}\]
Let us observe the straight line $ \overleftrightarrow {AE} $ . We take $ A $ as the initial point and take $ E $ as the other point to get the ray $ \overrightarrow{AE} $ . We take $ E $ as the initial point and $ A $ as the other point to get the ray $ \overrightarrow{EA} $ .SO we get two rays from the line $ \overleftrightarrow {AE} $ which are
\[\overrightarrow{AE},\overrightarrow{EA}\]
Let us observe the straight line $ \overleftrightarrow {BF} $ . We take $ B $ as the initial point and take $ F $ as the other point to get the ray $ \overrightarrow{BF} $ . We take $ F $ as the initial point and $ B $ as the other point to get the ray $ \overrightarrow{EA} $ .SO we get two rays from the line $ \overleftrightarrow {BF} $ which are
\[\overrightarrow{BF},\overrightarrow{FB}\]
Let us observe the straight line $ \overleftrightarrow {CG} $ . We take $ C $ as the initial point and take $ G $ as the other point to get the ray $ \overrightarrow{CG} $ . We take $ G $ as the initial point and $ C $ as the other point to get the ray $ \overrightarrow{EA} $ .SO we get two rays from the line $ \overleftrightarrow {CG} $ which are
\[\overrightarrow{CG},\overrightarrow{GC}\]
Let us observe the straight line $ \overleftrightarrow {DH} $ . We take $ D $ as the initial point and take $ H $ as the other point to get the ray $ \overrightarrow{DH} $ . We take $ H $ as the initial point and $ D $ as the other point to get the ray $ \overrightarrow{HD} $ .SO we get two rays from the line $ \overleftrightarrow {DH} $ which are
\[\overrightarrow{DH},\overrightarrow{HD}\]


So the names of all the rays from the figure are
\[\overrightarrow{AE},\overrightarrow{EA},\overrightarrow{BF},\overrightarrow{FB},\overrightarrow{CG},\overrightarrow{GC},\overrightarrow{DH},\overrightarrow{HD}\]
So there are 8 rays. \[\]
Note:
We know that a straight line is a basic geometric figure is made up of points and extending in two directions. A ray is part of the straight line but extended in only one direction. When two rays have a common point then they form an angle. We see in the figure that all the rays pass through the common point and hence at the common point many angles are formed.