
Refractive index of a rectangular glass slab is
(A)
(B)
(C)
(D)
Answer
438.6k+ views
Hint :Refractive index, also called index of refraction, measure of the bending of a ray of light when passing from one medium into another. The refractive index
Complete Step By Step Answer:
We have given the refractive index of slab, angle of incidence and the lateral displacement. We know that when the perpendicular distance between the original path traced by an incident ray and the path traced by the emergent ray coming out from the glass slab is known as lateral displacement. Now, we have to find the distance travelled by light in the slab.
We have the following diagram according to the question:
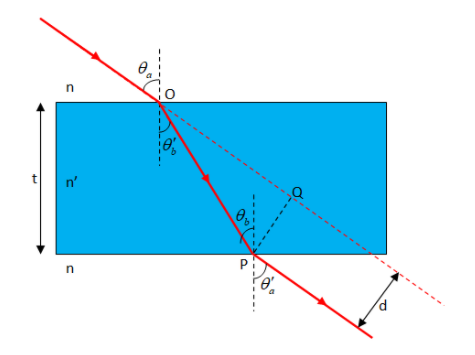
Given,
Refractive index of glass,
Angle of incidence,
Lateral displacement,
To find distance travelled by light in the slab
Here from Snell’s law we have:
Substituting values we get,
From trigonometric ratio we get,
Simplifying we get,
And so,
From geometry we have,
i.e.
Now, we have to find the length
Let the length travelled by light in glass be
From
And hence we have from trigonometric ratio,
Putting respective values we get,
Simplifying further
Hence option B is correct.
Note :
Formula for refractive index and idea of trigonometry is must for solving such questions comfortably. Diagrams are must for such types of questions as it gives a clear understanding. One should keep in mind that similar types of questions could be asked by changing the refractive index or the medium of travel of light. Also the incidence angle could be changed a bit which may lead calculation errors.
Complete Step By Step Answer:
We have given the refractive index of slab, angle of incidence and the lateral displacement. We know that when the perpendicular distance between the original path traced by an incident ray and the path traced by the emergent ray coming out from the glass slab is known as lateral displacement. Now, we have to find the distance travelled by light in the slab.
We have the following diagram according to the question:

Given,
Refractive index of glass,
Angle of incidence,
Lateral displacement,
To find distance travelled by light in the slab
Here from Snell’s law we have:
Substituting values we get,
From trigonometric ratio we get,
Simplifying we get,
And so,
From geometry we have,
i.e.
Now, we have to find the length
Let the length travelled by light in glass be
From
And hence we have from trigonometric ratio,
Putting respective values we get,
Simplifying further
Hence option B is correct.
Note :
Formula for refractive index and idea of trigonometry is must for solving such questions comfortably. Diagrams are must for such types of questions as it gives a clear understanding. One should keep in mind that similar types of questions could be asked by changing the refractive index or the medium of travel of light. Also the incidence angle could be changed a bit which may lead calculation errors.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




