Question

Represent \[\sqrt 7 \] on the number line.

Answer 1

Hint: We solve for the square root of the given number using the division method. Write the whole number under the square root with decimal and two pairs of zeroes after the decimal. Make pairs of two digits each starting from the right side to the left side. Find suitable multiple of the term to divide the given number. Plot the decimal value obtained on the number line.
* A number line is a representation of numbers on a straight line with equal distance between consecutive numbers.

Complete step by step answer:
We expand the number by writing the decimal after the number and 4 zeroes after the decimal.
The number 7 becomes 7.0000
First we pair the digits in the number after the decimal in pair of two each starting from the right hand side. Similarly we pair the digits before the decimal. Single digit left in the end will be a separate pair.
\[7 \cdot 0000 = \overline 7 \cdot \overline {00} \overline {00} \]
We take the highest number whose square will be less than or equal to the first pair i.e. 7
So, we know, \[1 \times 1 = 1,2 \times 2 = 4,3 \times 3 = 9\]
We choose \[2 \times 2 = 4\] because \[4 < 7\]
Now we divide the number by taking this number as divisor and taking the same number as quotient.
\[
  2\mathop{\left){\vphantom{1{\overline 7 .\overline {00} \overline {00} }}}\right.
\!\!\!\!\overline{\,\,\,\vphantom 1{{\overline 7 .\overline {00} \overline {00} }}}}
\limits^{\displaystyle \,\,\, 2} \\
   - 4 \\
  \overline { = 300} \\
 \]
Now the remainder becomes the next dividend and the new divisor is twice the old divisor followed by a digit which makes a number such that square of that number will be less than or equal to the new dividend. We place the decimal in the quotient and then write the next digit in the quotient.
So, we have new dividend as 300 and we can have divisor as \[2 \times 2\underline {} = 4\underline {} \]where blank is filled by the same digit.
Now we try to find a number in the lane of forties whose square is less than or equal to our new dividend.
\[
  41 \times 1 = 41 \\
  42 \times 2 = 84 \\
  43 \times 3 = 129 \\
  44 \times 4 = 176 \\
  45 \times 5 = 225 \\
  46 \times 6 = 276 \\
  47 \times 7 = 329 \\
 \]
We can clearly see that \[46 \times 6 = 276\] suits our requirement because \[276 < 300\]
Now we divide the dividend by the number 46 and the quotient 6 comes after the decimal beside the earlier quotient.
\[
  46\mathop{\left){\vphantom{1{300\overline {00} }}}\right.
\!\!\!\!\overline{\,\,\,\vphantom 1{{300\overline {00} }}}}
\limits^{\displaystyle \,\,\, {2.6}} \\
   - 276 \\
  \overline { = 2400} \\
 \]
So, we have new dividend as 2400 and we can have divisor as \[2 \times 26\underline {} = 52\underline {} \] where blank is filled by the same digit.
Now we try to find a number in the lane of five hundred and twenties whose square is less than or equal to our new dividend.
\[
  521 \times 1 = 521 \\
  522 \times 2 = 1044 \\
  523 \times 3 = 1569 \\
  524 \times 4 = 2096 \\
  525 \times 5 = 2625 \\
 \]
We can clearly see that \[524 \times 4 = 2096\] suits our requirement because \[2096 < 2400\]
Now we divide the dividend by the number 524 and the quotient 4 comes beside the earlier quotient.
\[
  524\mathop{\left){\vphantom{1{2400}}}\right.
\!\!\!\!\overline{\,\,\,\vphantom 1{{2400}}}}
\limits^{\displaystyle \,\,\, {2.64}} \\
   - 2096 \\
  \overline { = 304} \\
 \]
So, we have quotient up to two decimal places.
\[ \Rightarrow \sqrt 7 = 2.64\]Up to two decimal places
Now we plot the decimal number obtained on the number line.
We know \[2 < 2 \cdot 64 < 3\]
We draw a number line having integers at equal distance from each other.

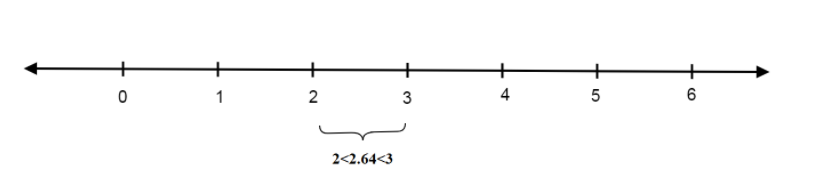
Now we magnify the section between 2 and 3.
We know\[2.5 < 2 \cdot 64 < 2.7\]
We draw a number line having interval 0.1 between the integers 2 and 3.

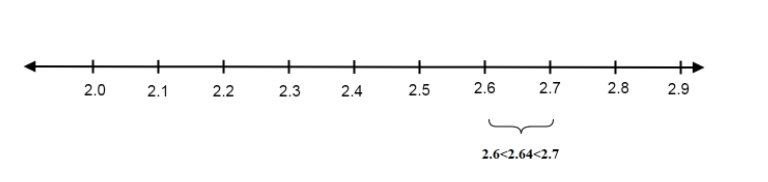
Now we magnify the section between 2.6 and 2.7
We know \[2.63 < 2 \cdot 64 < 2.65\]
We draw a number line having interval 0.01 between the integers 2.6 and 2.6.

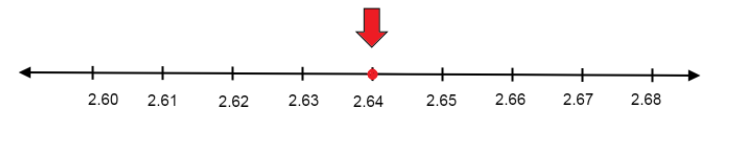
Therefore, \[\sqrt 7 \] represented on the number line is 2.64

Note:
Students might make mistakes in finding the root up to two decimal places as they might not know that we can fix any number of zeroes after the decimal and that will not change the value of the number. Also, when writing the division we need not write a decimal in new dividends as it will just cause confusion.
Also, while plotting the value on the number line, represent the value with a different color to avoid confusion.