Question

What is self-induction? Explain the experiment of self-induction and calculate self- inductance for a solenoid.

Answer 1

Hint: Recall that a current carrying conductor produces a magnetic field, and the strength of this field gives the magnetic flux linked with the conductor. Any change in the current through the conductor changes the flux linked with the conductor. Think of how a change in current would set up an emf across the conductor by virtue of its acquired magnetic flux, and in what polarity this emf would be set up depending on the current flowing through it. To this end, deduce the self-inductance of a solenoid and remember to account for the number of turns of wire in a solenoid.
Formula Used:
Self-inductance $L= \dfrac{\phi}{I}$
Self-inductance of a solenoid $L_{solenoid} = \dfrac{\mu_0N^2A}{l}$

Complete answer:
Let us approach this question in the perspective of Faraday’s first Law of Electromagnetic Induction and Lenz’s law of energy conservation which we can use to deduce the nature of the variation of emf with time, following which we shall define self-induction and calculate the self-inductance of a solenoid.
Faraday’s first law suggests that whenever the magnetic flux linked with a conductor changes, an emf is induced in the conductor. The induced emf exists as long as the change in magnetic flux continues to exist. Recall that magnetic flux is nothing but the magnetic field lines. The potential difference or emf thus produced will induce a current that flows around the conductor.
Lenz’s Law states that the polarity (direction) of the induced emf is always such that it tends to produce a current which opposes the change in magnetic flux that initially produced it.
Now, we know that when current passes through a coil, it produces a magnetic field around it. The flux due to this magnetic field is linked with the coil itself. If the current through the coil changes, the flux linked with it also changes and an emf is induced in the coil. Thus, a momentary change in the current flowing through the coil causes a change in its own flux which results in an emf, called as induced or back emf produced in the coil.
Thus, self-induction is the property of a coil by virtue of which it opposes the growth or decay of the current flowing through it by inducing an emf in the same coil.
Let us consider the following experiment to understand self-induction better.
We have a coil connected to a battery through a key K. Here, the change in flux linked with the coil will be brought about change in current flowing through it.

When the key is closed, due to increasing current in the coil, the magnetic field and consequently the magnetic flux linked with the coil increases. As a result, an induced emf is set up in the coil, in a direction that opposes the growth of current in the coil.
Now, the key is released cutting off the battery from the coil. The current in the coil now starts decreasing. This in turn decreases the magnetic flux linked with the coil, and this change in flux induces an emf in the coil, in a direction that opposes the decay of current in the coil. This is nothing but the emf that the coil induces by itself, i.e., self-induction.
Thus, we see that the magnetic flux $\phi$ linked with the coil is directly proportional to the strength of the current (I) flowing through it, i.e.,
$\phi \propto I \Rightarrow \phi = LI$, where L, which is the constant of proportionality is called as the coefficient of self-induction or self-inductance.
Now, let us look at the self-inductance of a solenoid. A solenoid is essentially made of turns of wire, that consists of multiple loops or coils put together,

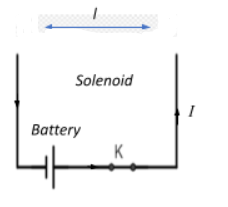
Consider a long solenoid of length l, cross-sectional area A, total number of turns N, number of turns per unit length n connected to a battery as shown in the diagram. We assume that the length of the solenoid is very large when compared with the radius of the cross-section.
Let I be the current flowing through the coils of the solenoid. This current proportionally produces a uniform magnetic field inside the solenoid whose strength is given as:
$B\propto nI \Rightarrow B = \mu_0nI$, where $\mu_0$ is the magnetic permeability of free space.
Now, the magnetic flux linked with each turn or loop of the solenoid is given as:
$\phi = B\times A = \mu_0 nIA$
If n is the number of turns per unit length l of the solenoid, then the total number of turns in the solenoid is given by $N = nl$.
Therefore, the total magnetic flux linked with the entire solenoid is given as:
$\phi = \mu_0nIA \times N = \mu_0nIA \times nl = \mu_0n^2IAl$
But from our qualitative definition of self-inductance we had $\phi = LI$
Equating the two, we get:
$LI = \mu_0n^2IAl$
$\Rightarrow$ Self inductance $L = \mu_0n^2Al = \mu_0.\dfrac{N^2}{l^2}Al = \dfrac{\mu_0N^2A}{l}$
Therefore, the self-inductance of a solenoid is found to be $L_{solenoid} = \dfrac{\mu_0N^2A}{l}$

Note:
Note that self-inductance is also known as electrical inertia since just like mechanical inertia which opposes the state of rest or motion of a body, self-inductance opposes the growth or decay of the current in the circuit.
Additionally, it is possible to have self-inductance without mutual inductance but mutual inductance cannot exist without self-inductance.