
Show graphically how charge on a capacitor and current through the capacitor varies with time during charging and discharging of the capacitor.
Answer
482.4k+ views
Hint: To solve the given question we must have the knowledge of how the charge on a capacitor and the current through it varies with time when the capacitor is charging and discharging. Write the corresponding equations, which will make it easy to interpret the graphs.
Complete answer:
A capacitor is a device that stores charge on it when a potential difference is created across it. When the positive charge is getting deposited on the capacitor, we say that the capacitor is charging. And when the positive charge on the capacitor is decreasing, we say that capacitor is discharging. Now, consider a capacitor with capacitance C connected across a cell of emf E. Let the circuit have some resistance R. When the circuit is switched on, the capacitor gets charged gradually. The charge (q) on the capacitor at time t, when it is charging is given as
where
The graph for the charge (q) on the capacitor with respect to time is as shown below:
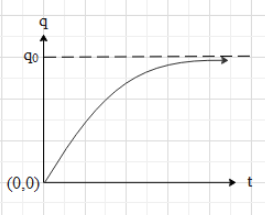
From the formula as also from the graph we can conclude that the charge on the capacitor during charging increases exponentially with respect to time. At time tending to infinity, the charge on the capacitor becomes equal to
While the capacitor is charging is charging, the current (i) through it at time t is given as
where
The graph for the current (i) through the capacitor with respect to time is as shown below:
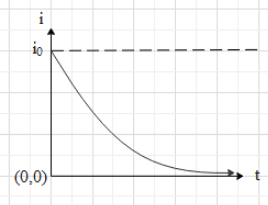
From the formula and from the graph, we conclude that when the capacitor is charging, current through it decreases exponentially with respect to time. Initially (when the circuit switched on), the current is maximum and equal to
Let us now see what happens to the charge and current when the capacitor is discharging.
Suppose we charge a capacitor with a cell and disconnect the cell. After the cell is disconnected, the capacitor will discharge. Let the initial charge on it is
Then charge on the capacitor is given as
The graph for the charge on a capacitor while it is discharging is given as:
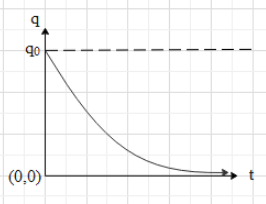
For these, we can conclude that again the charge on the capacitor is an exponential function of time. However, this time it decreases with time with a maximum value at time
The current through the capacitor when it is discharging is given as
The graph for the above is given as:
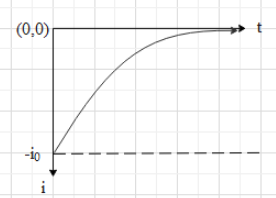
For these, we conclude that during discharging of a capacitor current (magnitude) in the circuit decreases exponentially with time.
Note: Student may misunderstand the last graph. They may think that the current is increasing in this case. It is correct that the value of current is becoming less negative with time, but note that its magnitude is decreasing. And we must only consider its magnitude. Note that the negative sign in equation (*) only shows that the capacitor is discharging.
Complete answer:
A capacitor is a device that stores charge on it when a potential difference is created across it. When the positive charge is getting deposited on the capacitor, we say that the capacitor is charging. And when the positive charge on the capacitor is decreasing, we say that capacitor is discharging. Now, consider a capacitor with capacitance C connected across a cell of emf E. Let the circuit have some resistance R. When the circuit is switched on, the capacitor gets charged gradually. The charge (q) on the capacitor at time t, when it is charging is given as
where
The graph for the charge (q) on the capacitor with respect to time is as shown below:

From the formula as also from the graph we can conclude that the charge on the capacitor during charging increases exponentially with respect to time. At time tending to infinity, the charge on the capacitor becomes equal to
While the capacitor is charging is charging, the current (i) through it at time t is given as
where
The graph for the current (i) through the capacitor with respect to time is as shown below:

From the formula and from the graph, we conclude that when the capacitor is charging, current through it decreases exponentially with respect to time. Initially (when the circuit switched on), the current is maximum and equal to
Let us now see what happens to the charge and current when the capacitor is discharging.
Suppose we charge a capacitor with a cell and disconnect the cell. After the cell is disconnected, the capacitor will discharge. Let the initial charge on it is
Then charge on the capacitor is given as
The graph for the charge on a capacitor while it is discharging is given as:

For these, we can conclude that again the charge on the capacitor is an exponential function of time. However, this time it decreases with time with a maximum value at time
The current through the capacitor when it is discharging is given as
The graph for the above is given as:

For these, we conclude that during discharging of a capacitor current (magnitude) in the circuit decreases exponentially with time.
Note: Student may misunderstand the last graph. They may think that the current is increasing in this case. It is correct that the value of current is becoming less negative with time, but note that its magnitude is decreasing. And we must only consider its magnitude. Note that the negative sign in equation (*) only shows that the capacitor is discharging.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




