
Show how
Answer
500.4k+ views
2 likes
Hint: We will first consider the given number which we have to represent on the number line. We will first draw the figure and draw the number line in it. We will mark two points 0 as O and 2 as A. After this draw a perpendicular at A and name the line as AX then mark a point at 1 unit on the perpendicular and mark it as the point B. Now, we will apply the Pythagoras theorem on the triangle OAB, and obtain the value of OB. Now, taking O as the center, we will cut the arc which will pass through the point B. The point on the right side of the point A that touches the number line will be the required point .
Complete step-by-step answer:
The objective is to represent
We will first consider the number line which is made on the
After this consider two points on the number line, zero is represented by O and 2 is represented as A. Now, draw a perpendicular on the point A and will name the line as AX.
Next, we will take 1 unit on the line AX and mark that point as B.
Now, we will apply the Pythagoras theorem on the triangle OAB and can find the value of line OB.
Thus, we have,
Hence,
Thus, we get the line OB as
Next, we will take O as the center and OB as
Thus,
Hence, the figure is shown as follows:
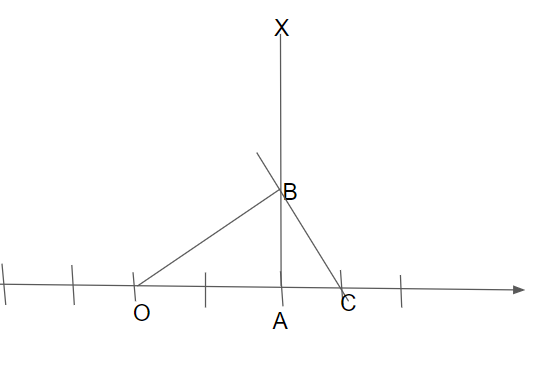
Thus, we can conclude that point C represents
Note: Constructing a figure is important. The value of line OB can be evaluated using the Pythagoras theorem only as triangle OAB is a right-angled triangle. The arc cutting the point B will touch the point C on the number line.
Complete step-by-step answer:
The objective is to represent
We will first consider the number line which is made on the
After this consider two points on the number line, zero is represented by O and 2 is represented as A. Now, draw a perpendicular on the point A and will name the line as AX.
Next, we will take 1 unit on the line AX and mark that point as B.
Now, we will apply the Pythagoras theorem on the triangle OAB and can find the value of line OB.
Thus, we have,
Hence,
Thus, we get the line OB as
Next, we will take O as the center and OB as
Thus,
Hence, the figure is shown as follows:

Thus, we can conclude that point C represents
Note: Constructing a figure is important. The value of line OB can be evaluated using the Pythagoras theorem only as triangle OAB is a right-angled triangle. The arc cutting the point B will touch the point C on the number line.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

What does R mean in math class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE





