
Show that if the diagonals of a quadrilateral are equal and bisect each other at right angles, then it is a square.
Answer
522.3k+ views
Hint: Proceed the solution of this question, keeping all the properties of square in mind as all the sides of square are equal, its diagonal bisect each other at
Complete Step-by-Step solution:
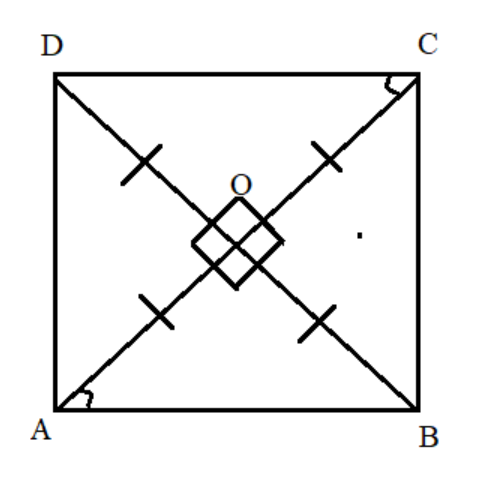
Given: In quadrilateral ABCD, diagonals AC and BD are equal (AC=BD) and bisect each other at right angles therefore we can say, AO=CO, OB = OD and ∠ AOB=∠ COD =∠ BOC=∠ AOD =
To prove: ABCD is a square
Proof:
In ∆AOB and ∆COD, we have
AO = CO [As Given in the question]
OB = OD [As Given in the question]
∠AOB = ∠COD [Vertically opposite angles and also given in the question as
SAS (Side-Angle-Side) rule-
In a triangle, if any two sides and angle included between the sides of one triangle are equivalent to the corresponding two sides and the angle between the sides of the other triangle, then those two triangles are said to be congruent by SAS rule
So, ∆AOB ≅ ∆COD, by S.A.S axiom of congruency
CPCT Rule
It stands for Corresponding parts of Congruent triangles. This theorem states that if two triangles which are congruent to each other are taken then the corresponding angles and the sides of the triangles are also equal to each other.
By C.P.C.T, we have
AB = CD …………. (1)
and ∠OAB = ∠OCD but these are alternate angles
Alternate angles- Two angles (∠OAB &∠OCD), formed when a transversal line(AC) crosses two other parallel lines (AB & CD), that lie on opposite sides of the transversal line and on opposite relative sides of the other lines. Then those angles are equal and called alternate angles.
So we have seen that, for alternate angles, the line must be parallel. So in the given figure, AB will be parallel to CD.
AB || CD …………. (2)
Similarly, In △AOD≅△COB
AO = CO [As Given in the question]
OD = OB [As Given in the question]
∠AOD = ∠COB [Vertically opposite angles and also given in the question as
So, ∆AOD ≅ ∆COB, by S.A.S axiom of congruency
By C.P.C.T, we have
AD = CB …………. (3)
and ∠ODA = ∠OBC but these are alternate angles
so, AD || CB ……… (4)
Now in △AOB and △COB.
AO=CO [As Given in the question]
OB=OB [common side in both △AOB and △COB]
∠AOB≅∠COB [each
So, ∆AOB ≅ ∆COB, by S.A.S axiom of congruency
By C.P.C.T, we have
AB = CB …………. (5)
Thus using (1), (3), (5)
Hence AB=BC=CD=DA
So all the sides of the quadrilateral become equal.
Now using (2), (4)
AB || CD &, AD || CB
So we can say that the opposite pair of sides (AB, CD) &, (AD, CB) are parallel.
And also in question it is given that, its diagonal intersect each other at
So the quadrilateral whose all four sides are equal and opposite sides are parallel and its diagonal intersecting at
Note: In this particular question, we can also prove it in a different way as we know that a parallelogram with one right vertex angle and two adjacent equal sides is also a square, so first we can prove it a parallelogram then to prove it is a square we can be prove its one right vertex angle and two adjacent equal sides using SAS (Side-Angle-Side) congruence rule of triangle and CPCT Rule.
Complete Step-by-Step solution:

Given: In quadrilateral ABCD, diagonals AC and BD are equal (AC=BD) and bisect each other at right angles therefore we can say, AO=CO, OB = OD and ∠ AOB=∠ COD =∠ BOC=∠ AOD =
To prove: ABCD is a square
Proof:
In ∆AOB and ∆COD, we have
AO = CO [As Given in the question]
OB = OD [As Given in the question]
∠AOB = ∠COD [Vertically opposite angles and also given in the question as
SAS (Side-Angle-Side) rule-
In a triangle, if any two sides and angle included between the sides of one triangle are equivalent to the corresponding two sides and the angle between the sides of the other triangle, then those two triangles are said to be congruent by SAS rule
So, ∆AOB ≅ ∆COD, by S.A.S axiom of congruency
CPCT Rule
It stands for Corresponding parts of Congruent triangles. This theorem states that if two triangles which are congruent to each other are taken then the corresponding angles and the sides of the triangles are also equal to each other.
By C.P.C.T, we have
AB = CD …………. (1)
and ∠OAB = ∠OCD but these are alternate angles
Alternate angles- Two angles (∠OAB &∠OCD), formed when a transversal line(AC) crosses two other parallel lines (AB & CD), that lie on opposite sides of the transversal line and on opposite relative sides of the other lines. Then those angles are equal and called alternate angles.
So we have seen that, for alternate angles, the line must be parallel. So in the given figure, AB will be parallel to CD.
AB || CD …………. (2)
Similarly, In △AOD≅△COB
AO = CO [As Given in the question]
OD = OB [As Given in the question]
∠AOD = ∠COB [Vertically opposite angles and also given in the question as
So, ∆AOD ≅ ∆COB, by S.A.S axiom of congruency
By C.P.C.T, we have
AD = CB …………. (3)
and ∠ODA = ∠OBC but these are alternate angles
so, AD || CB ……… (4)
Now in △AOB and △COB.
AO=CO [As Given in the question]
OB=OB [common side in both △AOB and △COB]
∠AOB≅∠COB [each
So, ∆AOB ≅ ∆COB, by S.A.S axiom of congruency
By C.P.C.T, we have
AB = CB …………. (5)
Thus using (1), (3), (5)
Hence AB=BC=CD=DA
So all the sides of the quadrilateral become equal.
Now using (2), (4)
AB || CD &, AD || CB
So we can say that the opposite pair of sides (AB, CD) &, (AD, CB) are parallel.
And also in question it is given that, its diagonal intersect each other at
So the quadrilateral whose all four sides are equal and opposite sides are parallel and its diagonal intersecting at
Note: In this particular question, we can also prove it in a different way as we know that a parallelogram with one right vertex angle and two adjacent equal sides is also a square, so first we can prove it a parallelogram then to prove it is a square we can be prove its one right vertex angle and two adjacent equal sides using SAS (Side-Angle-Side) congruence rule of triangle and CPCT Rule.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




