
Show that the semi-vertical angle of the cone of the maximum volume and given slant height is
Answer
489.3k+ views
Hint: We will use the formula for the volume of the cone, which is given by
Complete step-by-step solution
The volume of the cone is given by
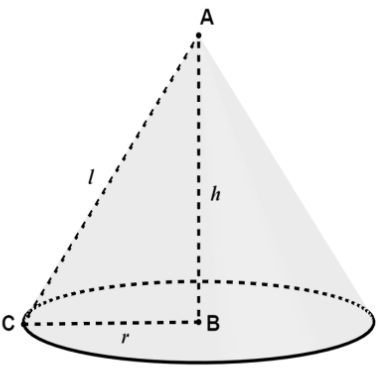
We have a triangle formed by the radius, height, and slant height of the cone. The radius and height are perpendicular to each other. In the right-angled triangle
Now, we want to maximize the volume of the cone. The volume is maximum when
We know that
We know that
We also know that
Now, we will equate the above equation to zero.
So, this implies that either
We know that if
This implies that
Note: It is important to understand that we get the critical points of a function
Complete step-by-step solution
The volume of the cone is given by

We have a triangle formed by the radius, height, and slant height of the cone. The radius and height are perpendicular to each other. In the right-angled triangle
Now, we want to maximize the volume of the cone. The volume is maximum when
We know that
We know that
We also know that
Now, we will equate the above equation to zero.
So, this implies that either
We know that if
This implies that
Note: It is important to understand that we get the critical points of a function
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




