Question

Solve the following inequality:
\[({x^2} - 2x)(2x - 2) - 9\dfrac{{2x - 2}}{{{x^2} - 2x}} \leqslant 0\]

Answer 1

Hint: We have to find the value of \[x\] from the given expression of inequality \[({x^2} - 2x)(2x - 2) - 9\dfrac{{2x - 2}}{{{x^2} - 2x}} \leqslant 0\]. We solve this question using the concept of solving linear equations of inequality. We will first simplify the given equation by taking \[(2x - 2)\] common from the left-hand side of the inequality. Then we factorise the obtained expression into its factors. And then by further solving we will find the range for the values of \[x\].

Complete step by step answer:
Given, \[({x^2} - 2x)(2x - 2) - \dfrac{{9\left( {2x - 2} \right)}}{{{x^2} - 2x}} \leqslant 0\]
By taking \[(2x - 2)\] common from left-hand side of the inequality, we get
\[ \Rightarrow (2x - 2)\left[ {({x^2} - 2x) - \dfrac{9}{{{x^2} - 2x}}} \right] \leqslant 0\]
On solving, we get
\[ \Rightarrow (2x - 2)\left[ {\dfrac{{{{({x^2} - 2x)}^2} - 9}}{{({x^2} - 2x)}}} \right] \leqslant 0\]
On rewriting the above inequality, we get
\[ \Rightarrow (2x - 2)\left[ {\dfrac{{{{({x^2} - 2x)}^2} - {{(3)}^2}}}{{({x^2} - 2x)}}} \right] \leqslant 0\]
Simplifying the numerator using the formula: \[{a^2} - {b^2} = \left( {a - b} \right)\left( {a + b} \right)\], we get
\[ \Rightarrow (2x - 2)\left[ {\dfrac{{\left( {{x^2} - 2x - 3} \right)\left( {{x^2} - 2x + 3} \right)}}{{({x^2} - 2x)}}} \right] \leqslant 0\]
Adding and subtracting in \[1\] in \[\left( {{x^2} - 2x + 3} \right)\], we get
\[ \Rightarrow (2x - 2)\left[ {\dfrac{{\left( {{x^2} - 2x - 3} \right)\left( {{x^2} - 2x + 1 + 3 - 1} \right)}}{{({x^2} - 2x)}}} \right] \leqslant 0\]
As \[\left( {{x^2} - 2x + 1} \right) = {\left( {x - 1} \right)^2}\], we can write the above inequality as
\[ \Rightarrow (2x - 2)\left[ {\dfrac{{\left( {{x^2} - 2x - 3} \right)\left( {{{\left( {x - 1} \right)}^2} + 2} \right)}}{{({x^2} - 2x)}}} \right] \leqslant 0\]
On further factorising and we get
\[ \Rightarrow (2x - 2)\left[ {\dfrac{{\left( {{x^2} + x - 3x - 3} \right)\left( {{{\left( {x - 1} \right)}^2} + 2} \right)}}{{({x^2} - 2x)}}} \right] \leqslant 0\]
Taking \[x\] common and on simplifying we get,
\[ \Rightarrow (2x - 2)\left[ {\dfrac{{\left( {x + 1} \right)\left( {x - 3} \right)\left( {{{\left( {x - 1} \right)}^2} + 2} \right)}}{{({x^2} - 2x)}}} \right] \leqslant 0\]
As \[{\left( {x - 1} \right)^2} + 2 > 0\], we can divide both the sides by \[\left( {{{\left( {x - 1} \right)}^2} + 2} \right)\], therefore we get
\[ \Rightarrow (2x - 2)\dfrac{{\left( {x + 1} \right)\left( {x - 3} \right)}}{{({x^2} - 2x)}} \leqslant 0\]
Taking \[2\] common from the numerator and \[x\] from denominator and on simplifying we get
\[ \Rightarrow \dfrac{{2(x - 1)\left( {x + 1} \right)\left( {x - 3} \right)}}{{x(x - 2)}} \leqslant 0\]
Dividing both the sides by \[2\], we get
\[ \Rightarrow \dfrac{{(x - 1)\left( {x + 1} \right)\left( {x - 3} \right)}}{{x(x - 2)}} \leqslant 0\]
Now we will put all the values of \[x\] on number line for which equality holds,

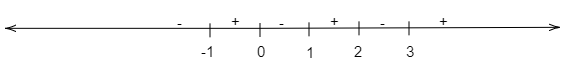
Let’s take any value of \[x\] greater than \[3\], say \[x = 4\], we get the value of \[\dfrac{{(x - 1)\left( {x + 1} \right)\left( {x - 3} \right)}}{{x(x - 2)}}\] as
\[ \Rightarrow \dfrac{{(4 - 1)\left( {4 + 1} \right)\left( {4 - 3} \right)}}{{4(4 - 2)}} = \dfrac{{15}}{8} > 0\]
On taking any value greater than \[3\], we get the value of inequality greater than zero.
Now taking any number between \[3\] and \[2\], say \[2.5\], we get
\[ \Rightarrow \dfrac{{(2.5 - 1)\left( {2.5 + 1} \right)\left( {2.5 - 3} \right)}}{{\left( {2.5} \right)(2.5 - 2)}} = - 2.1 < 0\]
Therefore, on taking any value between \[3\] and \[2\], we will get the value of inequality less than zero.
Now taking any number between \[2\] and \[1\], say \[1.5\], we get
\[ \Rightarrow \dfrac{{(1.5 - 1)\left( {1.5 + 1} \right)\left( {1.5 - 3} \right)}}{{\left( {1.5} \right)(1.5 - 2)}} = 2.5 > 0\]
Therefore, on taking any value between \[2\] and \[1\], we will get the value of inequality greater than zero.
Now, taking any number between \[1\] and \[0\], say \[0.5\], we get
\[ \Rightarrow \dfrac{{(0.5 - 1)\left( {0.5 + 1} \right)\left( {0.5 - 3} \right)}}{{\left( {0.5} \right)(0.5 - 2)}} = - 2.5 < 0\]
Therefore, on taking any value between \[1\] and \[0\], we will get the value of inequality less than zero.
Now, taking any number between \[0\] and \[ - 1\], say \[ - 0.5\], we get
\[ \Rightarrow \dfrac{{( - 0.5 - 1)\left( { - 0.5 + 1} \right)\left( { - 0.5 - 3} \right)}}{{\left( { - 0.5} \right)( - 0.5 - 2)}} = 2.1 > 0\]
Therefore, on taking any value between \[0\] and \[ - 1\], we will get the value of inequality greater than zero.
Now, taking any number less than \[ - 1\], say \[ - 2\], we get
\[ \Rightarrow \dfrac{{( - 2 - 1)\left( { - 2 + 1} \right)\left( { - 2 - 3} \right)}}{{\left( { - 2} \right)( - 2 - 2)}} = - \dfrac{{15}}{8} < 0\]
Therefore, on taking any number less than \[ - 1\], we will get the value of inequality less than zero.
Also, at \[x = - 1,0,1,2,3\] equality holds.
Therefore, the interval of \[x\] for which \[({x^2} - 2x)(2x - 2) - 9\dfrac{{2x - 2}}{{{x^2} - 2x}} \leqslant 0\] is when
\[ \Rightarrow x \in ( - \infty , - 1] \cup \left[ {0,1} \right] \cup \left[ {2,3} \right]\]

Note:
The solution range of inequality gives us each and every value of \[x\] which satisfies the equation. Here, one point to note is that square bracket \[\left[ {} \right]\] states that the end elements of the range will satisfy the given expression whereas the round bracket \[\left( {} \right)\] states that the end elements will not satisfy the given expression.