
Solve the following system of the linear equations graphically and shade the region between two lines and x – axis:
(i) 3x + 2y – 4 = 0; 2x – 3y – 7 = 0
(ii) 3x + 2y – 11 = 0; 2x – 3y + 10 = 0
Answer
518.1k+ views
Hint: In this question, first of all, get the two points passing from each line and draw the lines in x – y plane. Substitute x and y equal to 0 to get these points or any number of four choices. Now properly, see from the graph the point of intersection of this line which would be the solution of the equations.
Complete step-by-step answer:
In this question, we have to solve the following system of equations graphically.
(i) 3x + 2y – 4 = 0; 2x – 3y – 7 = 0
(ii) 3x + 2y – 11 = 0; 2x – 3y + 10 = 0
In this question, first of all, we will plot two lines and then check their point of the intersection by checking the point in the graph paper.
Let us consider the first set of the equation:
(i)
3x + 2y – 4 = 0…..(a)
2x – 3y – 7 = 0…..(b)
We know that to plot a line, we need at least two points through which it passes. So, by substituting x = 0 in equation (a), we get,
So, we get x = 0 and y = 2.
Now, let us substitute y = 0 in equation (a), we get,
So, we get,
So, for this line, we get,
By plotting these points in the graph, we get,
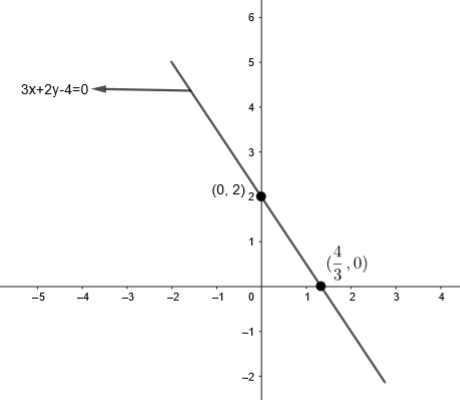
Similarly, by substituting x = 0 in equation (b), we get,
So, we get, x = 0 and
By substituting y = 0 in equation (b), we get,
So, we get,
So, for this line, we get,
By plotting these points in the graph, we get,
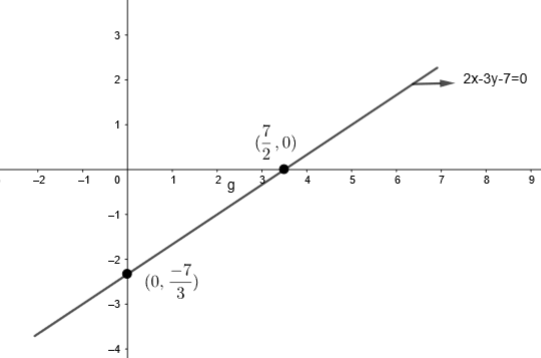
Now, we will draw both the lines in one graph, we get,
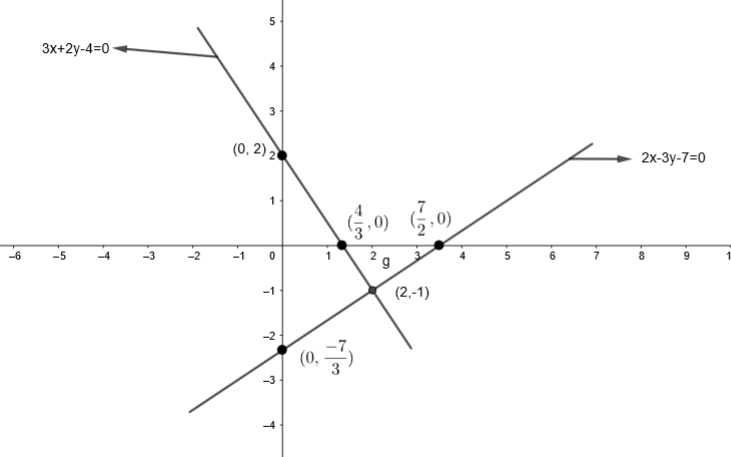
So, from the graph, we get the solution or intersection of lines as (x, y) = (2, – 1).
Similarly, let us consider the second set of the equations.
(ii)
3x + 2y – 11 = 0….(I)
2x – 3y + 10 = 0……(II)
By substituting x = 0 in equation (I), we get,
So, we get, x = 0 and
Now, by substituting y = 0 in equation (I), we get,
So, we get,
So, for this line, we get,
By plotting these points in the graph, we get,
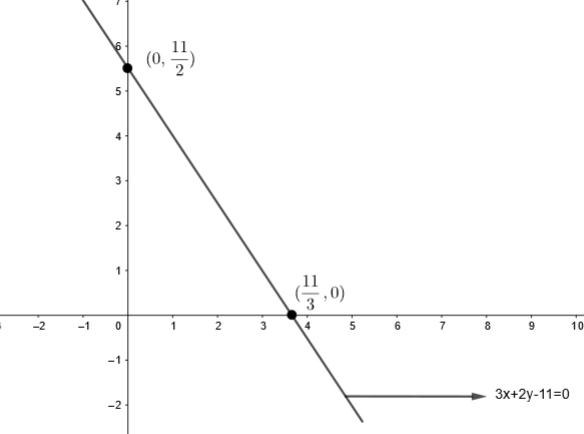
Now, by substituting y = 0 in equation (II), we get,
So, we get, x = – 5 and y = 0.
By substituting x = 0 in equation (II), we get,
So, we get, x = 0 and
So, for this line, we get,
By plotting these points in the graph, we get,
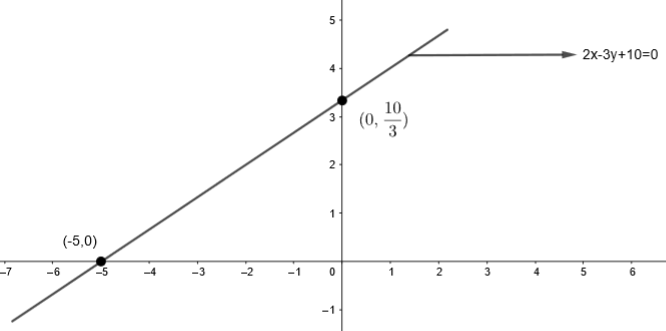
Now we will draw both the lines in one graph, we get,
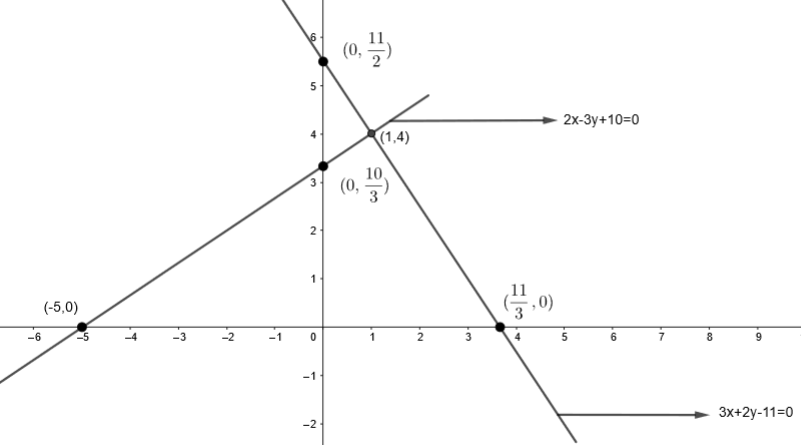
So, from the graph, we get the solution or intersection of the lines as (x, y) = (1, 4).
Note: In this question, it is very important to take the scale while drawing both the lines of each set assume because then only we will get the correct point of intersection. Also, students should cross-check their answer by substituting the point of intersection in both the equations of that set and checking if the value of x and y are satisfying them or not.
Complete step-by-step answer:
In this question, we have to solve the following system of equations graphically.
(i) 3x + 2y – 4 = 0; 2x – 3y – 7 = 0
(ii) 3x + 2y – 11 = 0; 2x – 3y + 10 = 0
In this question, first of all, we will plot two lines and then check their point of the intersection by checking the point in the graph paper.
Let us consider the first set of the equation:
(i)
3x + 2y – 4 = 0…..(a)
2x – 3y – 7 = 0…..(b)
We know that to plot a line, we need at least two points through which it passes. So, by substituting x = 0 in equation (a), we get,
So, we get x = 0 and y = 2.
Now, let us substitute y = 0 in equation (a), we get,
So, we get,
So, for this line, we get,
| x | 0 | |
| y | 2 | 0 |
By plotting these points in the graph, we get,

Similarly, by substituting x = 0 in equation (b), we get,
So, we get, x = 0 and
By substituting y = 0 in equation (b), we get,
So, we get,
So, for this line, we get,
| x | 0 | |
| y | 0 |
By plotting these points in the graph, we get,

Now, we will draw both the lines in one graph, we get,

So, from the graph, we get the solution or intersection of lines as (x, y) = (2, – 1).
Similarly, let us consider the second set of the equations.
(ii)
3x + 2y – 11 = 0….(I)
2x – 3y + 10 = 0……(II)
By substituting x = 0 in equation (I), we get,
So, we get, x = 0 and
Now, by substituting y = 0 in equation (I), we get,
So, we get,
So, for this line, we get,
| x | 0 | |
| y | 0 |
By plotting these points in the graph, we get,

Now, by substituting y = 0 in equation (II), we get,
So, we get, x = – 5 and y = 0.
By substituting x = 0 in equation (II), we get,
So, we get, x = 0 and
So, for this line, we get,
| x | 0 | -5 |
| y | 0 |
By plotting these points in the graph, we get,

Now we will draw both the lines in one graph, we get,

So, from the graph, we get the solution or intersection of the lines as (x, y) = (1, 4).
Note: In this question, it is very important to take the scale while drawing both the lines of each set assume because then only we will get the correct point of intersection. Also, students should cross-check their answer by substituting the point of intersection in both the equations of that set and checking if the value of x and y are satisfying them or not.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

What are the public facilities provided by the government? Also explain each facility

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE

The capital of British India was transferred from Calcutta class 10 social science CBSE

Identify the phrase s in the following sentence The class 10 english CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE




