
State and explain Biot- savart’s law.
Answer
496.2k+ views
3 likes
Hint: The Biot–Savart’s law is an equation describing the magnetic field generated by a constant electric current. It relates the magnetic field to the magnitude, direction, length, and electric current.
Complete step by step answer:
Biot-savart’s law is used to determine the strength of a magnetic field at any point due to a current-carrying conductor.
Consider a very small element AB of the length
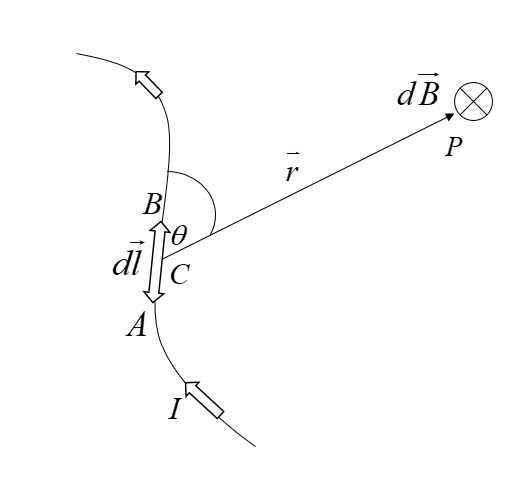
i)
ii)
iii)
iv)
On combining (i) to (iv), we get
i.e.
Where k is the constant of proportionality.
In SI units,
Additional information:
Some of the Biot-savart’s law applications are:
- We can use it to calculate magnetic responses even at the atomic or molecular level.
- It is also used in aerodynamic theory to calculate the velocity induced by vortex lines.
Note:
Biot-savart’s law is similar to coulomb’s law in electrostatics. This law is applicable for very small conductors too which vary current. It is also applicable for symmetrical current distribution. Biot–Savart law has evenness with both Ampere's circuital law and Gauss's theorem.
Complete step by step answer:
Biot-savart’s law is used to determine the strength of a magnetic field at any point due to a current-carrying conductor.
Consider a very small element AB of the length

i)
ii)
iii)
iv)
On combining (i) to (iv), we get
i.e.
Where k is the constant of proportionality.
In SI units,
Additional information:
Some of the Biot-savart’s law applications are:
- We can use it to calculate magnetic responses even at the atomic or molecular level.
- It is also used in aerodynamic theory to calculate the velocity induced by vortex lines.
Note:
Biot-savart’s law is similar to coulomb’s law in electrostatics. This law is applicable for very small conductors too which vary current. It is also applicable for symmetrical current distribution. Biot–Savart law has evenness with both Ampere's circuital law and Gauss's theorem.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




