
State and prove Brewster’s law.
Answer
431.6k+ views
3 likes
Hint: Brewster’s law states that an unpolarized light ray gets polarized maximum when it falls at a certain angle of incidence, called the Brewster’s angle onto an interface of another medium. The reflected and refracted rays are perpendicular to each other. The reflected ray is completely polarized.
Formula used:
When light travels from a medium of optical density
According to the laws of reflection, when light is reflected by a surface, the angle of incidence
Complete step by step answer:
Brewster’s law is a statement that says that when unpolarized light falls on an interface, the reflected light is completely polarized if the angle of incidence is a specific angle called the Brewster’s angle. In this case the angle made by the refracted ray and the reflected ray is
Let us draw a diagram to understand this better.
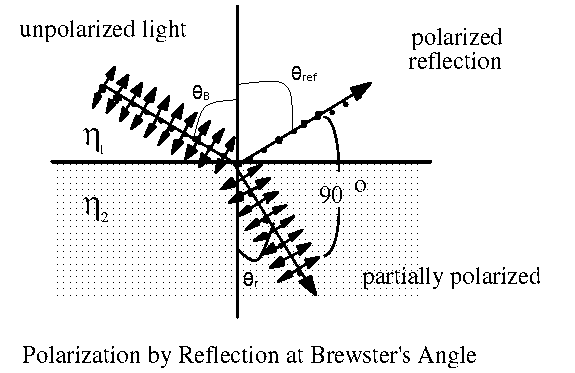
We will draw up a relation between the properties of the two media and the Brewster’s angle.
As shown in the figure, an unpolarized light ray falls on an interface between two media of respective optical densities
Now, when light travels from a medium of optical density
According to the laws of reflection, when light is reflected by a surface, the angle of incidence
Hence, using (1), we get,
From the figure’s geometry, we get,
Now, according to (2),
Using (3) and (5), we get,
where
Therefore, we have found a relation for the Brewster’s angle of incidence and the optical properties of the two media.
Note: Students must be familiar with the derivation of the brewster’s angle since it is very important and many theoretical as well as numerical questions are based upon this concept.
The glare of sunlight from the reflection of water is due to the polarized nature of the light reaching our eyes. If polarized sunglasses are used, the glare can be reduced since the polarized sunglasses only allow light of a certain polarization to enter and minimize the glare. This technology is the reason why authentic polarized sunglasses are so expensive.
Formula used:
When light travels from a medium of optical density
According to the laws of reflection, when light is reflected by a surface, the angle of incidence
Complete step by step answer:
Brewster’s law is a statement that says that when unpolarized light falls on an interface, the reflected light is completely polarized if the angle of incidence is a specific angle called the Brewster’s angle. In this case the angle made by the refracted ray and the reflected ray is
Let us draw a diagram to understand this better.

We will draw up a relation between the properties of the two media and the Brewster’s angle.
As shown in the figure, an unpolarized light ray falls on an interface between two media of respective optical densities
Now, when light travels from a medium of optical density
According to the laws of reflection, when light is reflected by a surface, the angle of incidence
Hence, using (1), we get,
From the figure’s geometry, we get,
Now, according to (2),
Using (3) and (5), we get,
where
Therefore, we have found a relation for the Brewster’s angle of incidence and the optical properties of the two media.
Note: Students must be familiar with the derivation of the brewster’s angle since it is very important and many theoretical as well as numerical questions are based upon this concept.
The glare of sunlight from the reflection of water is due to the polarized nature of the light reaching our eyes. If polarized sunglasses are used, the glare can be reduced since the polarized sunglasses only allow light of a certain polarization to enter and minimize the glare. This technology is the reason why authentic polarized sunglasses are so expensive.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




