
State Gauss’ Law in electrostatics. Using the law, derive an expression for the electric field due to a uniformly charged thin spherical shell at a point outside the shell.
Answer
430.5k+ views
Hint: Gauss’ Law in electrostatics relates the electric flux passing through a closed surface with the charge enclosed inside it. This law is very useful for deriving the electric field due to various charged bodies of different shapes. We can derive the electric field due to a uniformly thin charged spherical shell at a point outside it by drawing a closed Gaussian surface passing through this point.
Formula used:
Complete step-by-step solution -
Gauss’ law in electrostatics states that the electric flux passing through a closed surface is equal to the ratio of the charge enclosed by the surface to the permittivity of the medium.
Hence, mathematically,
where
The flux
Where
Putting (2) in (1), we get,
To derive the electric field due to a uniformly charged thin spherical shell at a point outside the shell, we will use Gauss’ Law. Hence, let us proceed to do that.
Let us take a uniformly charged thin spherical shell of radius
We will draw a closed Gaussian surface in the form of a circle which is concentric with the spherical shell and has a radius
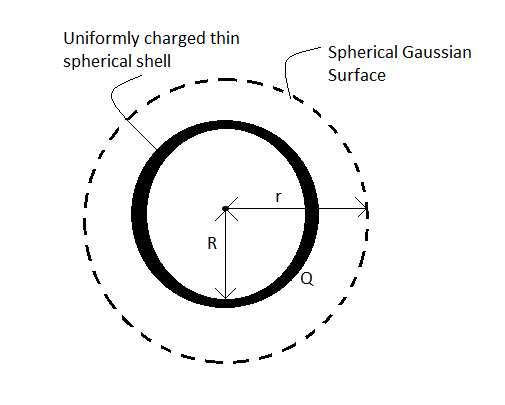
To get the electric field
Hence, using (3), we get,
Now, for a spherical surface of radius
Also, the charge enclosed in the Gaussian surface in this case is nothing but the total charge on the uniformly charged thin spherical shell. Therefore,
Therefore, using (5) and (6) in (4), we get,
Hence, we have derived the electric field at a point outside a uniformly charged thin spherical shell.
Note: Students must remember that the electric field inside the uniformly charged thin spherical is zero. This is because if we draw a Gaussian surface inside the shell, there will be no charge enclosed inside it and hence, from Gauss’ law, the electric field inside it will also be zero. Hence, this also proves the fact that if a charge is given to a thin spherical shell, all of the charge will come out and spread uniformly on the outer surface of the shell.
Formula used:
Complete step-by-step solution -
Gauss’ law in electrostatics states that the electric flux passing through a closed surface is equal to the ratio of the charge enclosed by the surface to the permittivity of the medium.
Hence, mathematically,
where
The flux
Where
Putting (2) in (1), we get,
To derive the electric field due to a uniformly charged thin spherical shell at a point outside the shell, we will use Gauss’ Law. Hence, let us proceed to do that.
Let us take a uniformly charged thin spherical shell of radius
We will draw a closed Gaussian surface in the form of a circle which is concentric with the spherical shell and has a radius

To get the electric field
Hence, using (3), we get,
Now, for a spherical surface of radius
Also, the charge enclosed in the Gaussian surface in this case is nothing but the total charge on the uniformly charged thin spherical shell. Therefore,
Therefore, using (5) and (6) in (4), we get,
Hence, we have derived the electric field at a point outside a uniformly charged thin spherical shell.
Note: Students must remember that the electric field inside the uniformly charged thin spherical is zero. This is because if we draw a Gaussian surface inside the shell, there will be no charge enclosed inside it and hence, from Gauss’ law, the electric field inside it will also be zero. Hence, this also proves the fact that if a charge is given to a thin spherical shell, all of the charge will come out and spread uniformly on the outer surface of the shell.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




