
What is the sum of the measures of the angles of a convex quadrilateral? Will this property hold if the quadrilateral is not convex? (Make a non-convex quadrilateral and try?)
Answer
459.3k+ views
Hint: We use the concept of convex and non-convex quadrilateral and break each quadrilateral into two halves. Use the property of the sum of interior angles of a triangle to find the sum of interior angles in each triangle. Add the angles and write the sum of angles of the quadrilateral.
Complete step-by-step answer:
Let us draw a convex quadrilateral ABCD having a diagonal AC.

In quadrilateral we have four angles \[\angle A,\angle B,\angle C,\angle D\]
So, the sum of interior angles of quadrilateral ABCD is given as \[\angle A + \angle B + \angle C + \angle D\].................… (1)
We have diagonal AC which divides quadrilateral ABCD into two triangles, \[\vartriangle ABC\] and \[\vartriangle ADC\]
We apply the property of the sum of interior angles in each triangle.
In\[\vartriangle ABC\],
\[\angle BAC + \angle B + \angle BCA = {180^ \circ }\]..............… (2)
In\[\vartriangle ADC\],
\[\angle CAD + \angle D + \angle ACD = {180^ \circ }\]................… (3)
Add equations (3) and (4)
\[ \Rightarrow \angle BAC + \angle B + \angle BCA + \angle CAD + \angle D + \angle ACD = {180^ \circ } + {180^ \circ }\]
\[ \Rightarrow \left( {\angle BAC + \angle CAD} \right) + \angle B + \left( {\angle BCA + \angle ACD} \right) + \angle D = {360^ \circ }\]
Since, we know \[\left( {\angle BAC + \angle CAD} \right) = \angle A;\left( {\angle BCA + \angle ACD} \right) = \angle B\]
\[ \Rightarrow \angle A + \angle B + \angle C + \angle D = {360^ \circ }\]
From equation (1), LHS is the sum of all interior angles of quadrilateral ABCD
\[\therefore \]Sum of interior angles of a convex quadrilateral is \[{360^ \circ }\].
Now let us assume PQRS as a non-convex quadrilateral, having diagonal PR
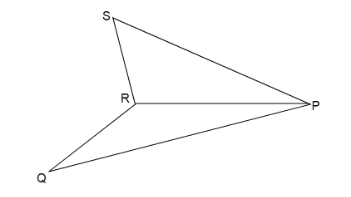
In quadrilateral we have four angles \[\angle P,\angle Q,\angle R,\angle S\]
So, the sum of interior angles of quadrilateral ABCD is given as \[\angle P + \angle Q + \angle R + \angle S\]............… (4)
Again we divide the quadrilateral PQRS into two triangles, \[\vartriangle PQR\] and \[\vartriangle PSR\]
We apply the property of the sum of interior angles in each triangle.
In \[\vartriangle PQR\],
\[\angle PRQ + \angle Q + \angle RPQ = {180^ \circ }\]............… (5)
In\[\vartriangle PSR\],
\[\angle PRS + \angle S + \angle RPS = {180^ \circ }\]...........… (6)
Add equations (5) and (6)
\[ \Rightarrow \angle PRQ + \angle Q + \angle RPQ + \angle PRS + \angle S + \angle RPS = {180^ \circ } + {180^ \circ }\]
\[ \Rightarrow \left( {\angle PRQ + \angle PRS} \right) + \angle Q + \left( {\angle RPQ + \angle RPS} \right) + \angle S = {360^ \circ }\]
Since, we know \[\left( {\angle PRQ + \angle PRS} \right) = \angle R;\left( {\angle RPQ + \angle RPS} \right) = \angle P\]
\[ \Rightarrow \angle P + \angle Q + \angle R + \angle S = {360^ \circ }\]
From equation (2), LHS is the sum of all interior angles of quadrilateral PQRS
\[\therefore \]Sum of interior angles of a non-convex quadrilateral is also \[{360^ \circ }\].
So, the property holds true even if the quadrilateral is non-convex.
Note: Convex Quadrilateral: A quadrilateral which has each interior angle less than\[{180^ \circ }\]and both the diagonals lie inside the quadrilateral is called a convex quadrilateral.
Non-convex quadrilateral: A quadrilateral having one interior angle greater than \[{180^ \circ }\] and diagonal lies outside the closed shape of the quadrilateral is called a non-convex of concave quadrilateral.
* In any triangle ABC, with angles A, B and C the sum of interior angles adds up to \[{180^ \circ }\]
\[\angle A + \angle B + \angle C = {180^ \circ }\]
Complete step-by-step answer:
Let us draw a convex quadrilateral ABCD having a diagonal AC.

In quadrilateral we have four angles \[\angle A,\angle B,\angle C,\angle D\]
So, the sum of interior angles of quadrilateral ABCD is given as \[\angle A + \angle B + \angle C + \angle D\].................… (1)
We have diagonal AC which divides quadrilateral ABCD into two triangles, \[\vartriangle ABC\] and \[\vartriangle ADC\]
We apply the property of the sum of interior angles in each triangle.
In\[\vartriangle ABC\],
\[\angle BAC + \angle B + \angle BCA = {180^ \circ }\]..............… (2)
In\[\vartriangle ADC\],
\[\angle CAD + \angle D + \angle ACD = {180^ \circ }\]................… (3)
Add equations (3) and (4)
\[ \Rightarrow \angle BAC + \angle B + \angle BCA + \angle CAD + \angle D + \angle ACD = {180^ \circ } + {180^ \circ }\]
\[ \Rightarrow \left( {\angle BAC + \angle CAD} \right) + \angle B + \left( {\angle BCA + \angle ACD} \right) + \angle D = {360^ \circ }\]
Since, we know \[\left( {\angle BAC + \angle CAD} \right) = \angle A;\left( {\angle BCA + \angle ACD} \right) = \angle B\]
\[ \Rightarrow \angle A + \angle B + \angle C + \angle D = {360^ \circ }\]
From equation (1), LHS is the sum of all interior angles of quadrilateral ABCD
\[\therefore \]Sum of interior angles of a convex quadrilateral is \[{360^ \circ }\].
Now let us assume PQRS as a non-convex quadrilateral, having diagonal PR

In quadrilateral we have four angles \[\angle P,\angle Q,\angle R,\angle S\]
So, the sum of interior angles of quadrilateral ABCD is given as \[\angle P + \angle Q + \angle R + \angle S\]............… (4)
Again we divide the quadrilateral PQRS into two triangles, \[\vartriangle PQR\] and \[\vartriangle PSR\]
We apply the property of the sum of interior angles in each triangle.
In \[\vartriangle PQR\],
\[\angle PRQ + \angle Q + \angle RPQ = {180^ \circ }\]............… (5)
In\[\vartriangle PSR\],
\[\angle PRS + \angle S + \angle RPS = {180^ \circ }\]...........… (6)
Add equations (5) and (6)
\[ \Rightarrow \angle PRQ + \angle Q + \angle RPQ + \angle PRS + \angle S + \angle RPS = {180^ \circ } + {180^ \circ }\]
\[ \Rightarrow \left( {\angle PRQ + \angle PRS} \right) + \angle Q + \left( {\angle RPQ + \angle RPS} \right) + \angle S = {360^ \circ }\]
Since, we know \[\left( {\angle PRQ + \angle PRS} \right) = \angle R;\left( {\angle RPQ + \angle RPS} \right) = \angle P\]
\[ \Rightarrow \angle P + \angle Q + \angle R + \angle S = {360^ \circ }\]
From equation (2), LHS is the sum of all interior angles of quadrilateral PQRS
\[\therefore \]Sum of interior angles of a non-convex quadrilateral is also \[{360^ \circ }\].
So, the property holds true even if the quadrilateral is non-convex.
Note: Convex Quadrilateral: A quadrilateral which has each interior angle less than\[{180^ \circ }\]and both the diagonals lie inside the quadrilateral is called a convex quadrilateral.
Non-convex quadrilateral: A quadrilateral having one interior angle greater than \[{180^ \circ }\] and diagonal lies outside the closed shape of the quadrilateral is called a non-convex of concave quadrilateral.
* In any triangle ABC, with angles A, B and C the sum of interior angles adds up to \[{180^ \circ }\]
\[\angle A + \angle B + \angle C = {180^ \circ }\]
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Trending doubts
When people say No pun intended what does that mea class 8 english CBSE

Which king started the organization of the Kumbh fair class 8 social science CBSE

What is BLO What is the full form of BLO class 8 social science CBSE

Advantages and disadvantages of science

What are the 12 elements of nature class 8 chemistry CBSE

Write a letter to the Municipal Commissioner to inform class 8 english CBSE




