
What is the sum of the measures of the angles of a convex quadrilateral? Will this property hold if the quadrilateral is not convex? (Make a non-convex quadrilateral and try?)
Answer
483k+ views
Hint: We use the concept of convex and non-convex quadrilateral and break each quadrilateral into two halves. Use the property of the sum of interior angles of a triangle to find the sum of interior angles in each triangle. Add the angles and write the sum of angles of the quadrilateral.
Complete step-by-step answer:
Let us draw a convex quadrilateral ABCD having a diagonal AC.

In quadrilateral we have four angles
So, the sum of interior angles of quadrilateral ABCD is given as
We have diagonal AC which divides quadrilateral ABCD into two triangles,
We apply the property of the sum of interior angles in each triangle.
In
In
Add equations (3) and (4)
Since, we know
From equation (1), LHS is the sum of all interior angles of quadrilateral ABCD
Now let us assume PQRS as a non-convex quadrilateral, having diagonal PR
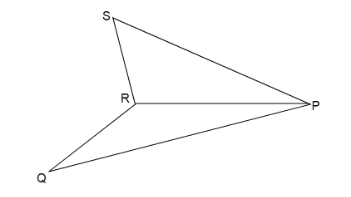
In quadrilateral we have four angles
So, the sum of interior angles of quadrilateral ABCD is given as
Again we divide the quadrilateral PQRS into two triangles,
We apply the property of the sum of interior angles in each triangle.
In
In
Add equations (5) and (6)
Since, we know
From equation (2), LHS is the sum of all interior angles of quadrilateral PQRS
So, the property holds true even if the quadrilateral is non-convex.
Note: Convex Quadrilateral: A quadrilateral which has each interior angle less than
Non-convex quadrilateral: A quadrilateral having one interior angle greater than
* In any triangle ABC, with angles A, B and C the sum of interior angles adds up to
Complete step-by-step answer:
Let us draw a convex quadrilateral ABCD having a diagonal AC.

In quadrilateral we have four angles
So, the sum of interior angles of quadrilateral ABCD is given as
We have diagonal AC which divides quadrilateral ABCD into two triangles,
We apply the property of the sum of interior angles in each triangle.
In
In
Add equations (3) and (4)
Since, we know
From equation (1), LHS is the sum of all interior angles of quadrilateral ABCD
Now let us assume PQRS as a non-convex quadrilateral, having diagonal PR

In quadrilateral we have four angles
So, the sum of interior angles of quadrilateral ABCD is given as
Again we divide the quadrilateral PQRS into two triangles,
We apply the property of the sum of interior angles in each triangle.
In
In
Add equations (5) and (6)
Since, we know
From equation (2), LHS is the sum of all interior angles of quadrilateral PQRS
So, the property holds true even if the quadrilateral is non-convex.
Note: Convex Quadrilateral: A quadrilateral which has each interior angle less than
Non-convex quadrilateral: A quadrilateral having one interior angle greater than
* In any triangle ABC, with angles A, B and C the sum of interior angles adds up to
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE




