Question

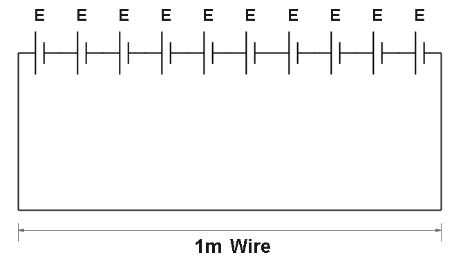
Ten identical cells connected in series are needed to heat a wire of length $1\,m$ and radius $r$ by ${10^ \circ }C$ in time$t$ . How many cells will be required to heat the wire of length $2\,m$ of the same radius by the same temperature in time $t$ ?

Answer 1

Hint: Here we have to find the number of cells required to heat the wire of given length and to solve this type of question, one must know the concept of specific heat. Here we will put the given data in the formula of heat capacity and then we will compare the obtained relation to get the required solution.

Formula used:
$R = \rho \dfrac{l}{A}$
Where, $r$ is the resistance of the wire, $\rho $ is the resistivity of the material, $l$ is the length of the material and $a$ is the area of the cross section.

Complete step by step answer:
Let us assume, $\rho $ is the resistivity of the wire, $r$ is the radius of the wire and $E$ be the emf of each cell. According to the question, the length of the wire is $1\,m$.

And $10$ cells each having an emf of $E$ are connected in series to heat the wire by,
$\Delta t = {10^ \circ }C$ in time $t$ .
As we know that, wire will take to generate the heat by $\dfrac{{{V^2}}}{R} \times t$
So, we can write, $\dfrac{{{V^2}}}{R} \times t = ms\Delta T$
And we know that mass is directly proportional to length. So, we can write,
$\dfrac{{{{\left( {10E} \right)}^2}}}{R} \times t = ms\Delta T$ -----(1)

And resistance of the same wire of length of $2m$ is,
$R' = \dfrac{{\rho (2)}}{a} \\
\Rightarrow R' = 2R \\ $
Now, let $n$ be the number of cells having emf $E$ are connected in series to heat the same wire which is of length $2m$ is,
$\dfrac{{{{\left( {nE} \right)}^2}t}}{{2R}} = \left( {2m} \right)s\Delta T$ -----(2)
And now dividing the equation (2) by (1),
\[\Rightarrow \dfrac{{\dfrac{{{{\left( {nE} \right)}^2}t}}{{2R}} = \left( {2m} \right)s\Delta T}}{{\dfrac{{{{\left( {10E} \right)}^2}}}{R} \times t = ms\Delta T}} \\
\Rightarrow \dfrac{{{n^2}}}{{200}} = 2 \\
\Rightarrow {n^2} = 400 \\
\therefore n = 20 \\ \]
Hence, $20$ cells will be required to heat the wire of length $2m$ of the same radius by the same temperature in time $t$.

Note: Remember the formula of specific heat and when an electric current is conducted through a conductor, heat is generated due to the conductor's impediment to the current flowing inside. The amount of effort required to overcome this impediment to electric current generates heat in that particular conductor.