
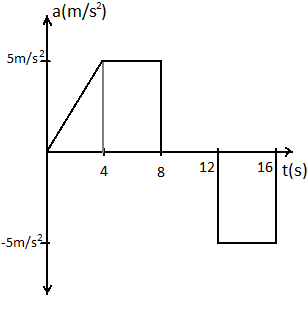
The acceleration of a train between two stations is shown in the figure. The maximum speed of the train is:
A) $60m{s^{ - 1}}$
B) $30m{s^{ - 1}}$
C) $120m{s^{ - 1}}$
D) $90m{s^{ - 1}}$
Answer
143.7k+ views
Hint: Define acceleration and try to recall the mathematical expression of acceleration. From the graph and from the relation between velocity and acceleration we can easily estimate the maximum speed the train can reach.
Complete step by step solution:
Let’s have some nostalgia and revisit our classes of kinematics. Our physics teacher was yelling that acceleration is the rate of change of velocity.
For a small change in velocity \[dv\] over a time $dt$ acceleration of a body can be represented mathematically as,
$a = \dfrac{{dv}}{{dt}}$ ……….. (1)
Further simplifying,
$ \Rightarrow dv = a \times dt$
For the maximum velocity, we can integrate both sides of the above equation.
Taking integration both sides, we get
$ \Rightarrow v = \int {adt} $ ………. (2)
This is the expression for velocity in terms of acceleration.
Now we know that integrating a function/ curve, we get the area under that function/ curve. Thus from equation (2), we can conclude that the area under the acceleration-time graph is defined as the velocity of the train/ moving object.
Now from the diagram given in the question, we can see that after $12s$ mark, the acceleration of the train is negative, so in order to calculate the maximum velocity, we can take the area under the graph before the 12s mark.
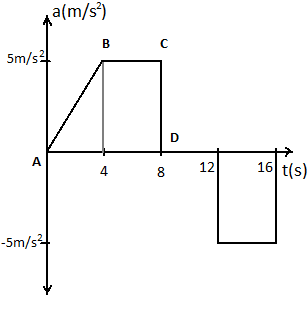
From the above diagram the area of the section $ABCD$ gives us the maximum velocity.
Area of $ABCD$ is the sum of area of right angled $\vartriangle ABE$ and the rectangle $BCDE$.
Area of $\vartriangle ABE$ is $\dfrac{1}{2} \times 4 \times 5 = 10$
Area of rectangle $BCDE$ is $5 \times 4 = 20$
Thus the maximum velocity of the train would be $10 + 20 = 30m{s^{ - 1}}$
So the correct answer is option B.
Note: Using this technique we can solve problems where we are provided with a graph in simple kinematics, for example, in a velocity time graph, the area under the graph represents the displacement of the moving body. Because the rate of change of displacement is defined as velocity.
Complete step by step solution:
Let’s have some nostalgia and revisit our classes of kinematics. Our physics teacher was yelling that acceleration is the rate of change of velocity.
For a small change in velocity \[dv\] over a time $dt$ acceleration of a body can be represented mathematically as,
$a = \dfrac{{dv}}{{dt}}$ ……….. (1)
Further simplifying,
$ \Rightarrow dv = a \times dt$
For the maximum velocity, we can integrate both sides of the above equation.
Taking integration both sides, we get
$ \Rightarrow v = \int {adt} $ ………. (2)
This is the expression for velocity in terms of acceleration.
Now we know that integrating a function/ curve, we get the area under that function/ curve. Thus from equation (2), we can conclude that the area under the acceleration-time graph is defined as the velocity of the train/ moving object.
Now from the diagram given in the question, we can see that after $12s$ mark, the acceleration of the train is negative, so in order to calculate the maximum velocity, we can take the area under the graph before the 12s mark.

From the above diagram the area of the section $ABCD$ gives us the maximum velocity.
Area of $ABCD$ is the sum of area of right angled $\vartriangle ABE$ and the rectangle $BCDE$.
Area of $\vartriangle ABE$ is $\dfrac{1}{2} \times 4 \times 5 = 10$
Area of rectangle $BCDE$ is $5 \times 4 = 20$
Thus the maximum velocity of the train would be $10 + 20 = 30m{s^{ - 1}}$
So the correct answer is option B.
Note: Using this technique we can solve problems where we are provided with a graph in simple kinematics, for example, in a velocity time graph, the area under the graph represents the displacement of the moving body. Because the rate of change of displacement is defined as velocity.
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Degree of Dissociation and Its Formula With Solved Example for JEE

Physics Average Value and RMS Value JEE Main 2025

Other Pages
Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




