
The angle of deviation when light is incident at an angle of
Answer
504.6k+ views
Hint: By using the formula of refractive index for prism, we get the correct answer. The angle of deviation of a ray of light passing through a prism depends upon its material as well as on the angle of incidence. Here it means it depends upon the refractive index of a material of prism.
Complete answer step by step:
The given prism is equilateral so the three angles are of
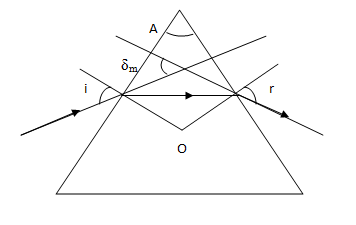
The angle of incident is given,
We know that the equation for refractive index of prism,
where µ is the refractive index of prism and
Substituting given values in above equation we get,
We know
Multiplying both sides by
We know,
Therefore, the angle of deviation when light is incident at an angle of
Hence the correct option is B.
Note: The given prism is equilateral, so the angle of the prism is known and also the angle of incidence is given with the refractive index of the prism. Here, Snell’s equation is used and substituted values of ‘i' and ‘r’. And they are
So, if we know which material is used and what is the angle of incident, we can solve any problem of prism.
Complete answer step by step:
The given prism is equilateral so the three angles are of

The angle of incident is given,
We know that the equation for refractive index of prism,
where µ is the refractive index of prism and
Substituting given values in above equation we get,
We know
Multiplying both sides by
We know,
Therefore, the angle of deviation when light is incident at an angle of
Hence the correct option is B.
Note: The given prism is equilateral, so the angle of the prism is known and also the angle of incidence is given with the refractive index of the prism. Here, Snell’s equation is used and substituted values of ‘i' and ‘r’. And they are
So, if we know which material is used and what is the angle of incident, we can solve any problem of prism.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write a short note on Franklands reaction class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




