Question

The area of the circle inscribed in an equilateral triangle is 154 $c{{m}^{2}}$. Find the perimeter of the triangle.( Use $\pi =\dfrac{22}{7}$ and $\sqrt{3}=1.73$)

Answer 1

Hint: Here, first of all we will use the concept of area of a circle. The area of a circle is given as $A=\pi \times {{\left( radius \right)}^{2}}$. Also, the points where the angular bisectors of an equilateral triangle meet is the incentre of the triangle. These angular bisectors are also the altitude and median whose point of intersection divides the medians in the ratio 2:1. Also, the altitude of an equilateral triangle has length equal to $\dfrac{\sqrt{3}}{2}$ times its side.

Complete step-by-step answer:

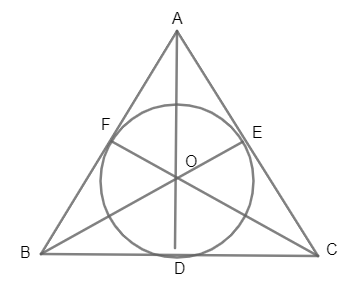
Here, we are given that the area of the circle is 154 $c{{m}^{2}}$.
Let the radius of the circle be = r.
Then, we have:
$\begin{align}
  & \pi {{r}^{2}}=154c{{m}^{2}} \\
 & \Rightarrow {{r}^{2}}=\dfrac{154}{\pi }c{{m}^{2}} \\
 & \Rightarrow {{r}^{2}}=\dfrac{154}{\dfrac{22}{7}}c{{m}^{2}} \\
 & \Rightarrow {{r}^{2}}=49c{{m}^{2}} \\
 & \Rightarrow r=\sqrt{49}cm=7cm \\
\end{align}$
Let ‘h’ be the altitude of the given triangle, that is, AD = BE = CF = h.
Now, we know that the angular bisector of an equilateral triangle bisects the opposite side at right angle and also the point of their intersection, that is, the incentre ( here incentre is O) divides the median in the ratio 2:1.
Therefore, we can write:
$\angle ADB={{90}^{0}}$ and $OD=\dfrac{1}{3}AD........\left( 1 \right)$.
Also, OD is the radius of the circle, that is r and AD = h.
So, on putting the required values in equation (1), we get:
$\begin{align}
  & r=\dfrac{h}{3} \\
 & \Rightarrow h=3r \\
\end{align}$
We have already found that the value of r is 7 cm. So, we have:
$h=3\times 7=21cm........\left( 2 \right)$
Now, let each side of the equilateral triangle be ‘a’. Since, the altitude of an equilateral triangle is $\dfrac{\sqrt{3}}{2}$ times the length of its side, we can write:
$\begin{align}
  & h=\dfrac{\sqrt{3}}{2}a \\
 & \Rightarrow a=\dfrac{2h}{\sqrt{3}} \\
\end{align}$
On putting the value of h from equation (2), we get:
$\begin{align}
  & a=\dfrac{2\times 21}{\sqrt{3}}cm \\
 & \Rightarrow a=\dfrac{42}{\sqrt{3}}cm=\dfrac{42\times \sqrt{3}}{\sqrt{3}\times \sqrt{3}}cm=\dfrac{42\sqrt{3}}{3}cm=14\sqrt{3}cm \\
\end{align}$
So, the perimeter of the triangle ABC= $3\times a=3\times 14\sqrt{3}cm=42\times 1.73cm=72.66cm$.

Note: Here, it is not necessary that students take OD as the radius and AD as the altitude. Any one of AD, BE or CF can be taken as altitude and OD, OE and OF as radius respectively because the given triangle is equilateral and hence AD = BE = CF and OD = OE = OF because they are the radius of the same circle.