
The area under acceleration – time graph gives
A. Change in acceleration.
B. Change in velocity.
C. Change in displacement.
D. Change in deceleration.
Answer
499.2k+ views
Hint: Acceleration is defined as change in velocity (
Formulae Used:
Where
Complete step-by-step solution:
Since instantaneous acceleration is given by
Rearranging the terms
Integrating both sides , we get
Since, the area of the curve between acceleration – time graph shows the change in velocity of the moving object.
Hence, option ( B ) is the correct answer.
In case of uniform motion velocity remains constant , and displacement is the linear function of velocity as
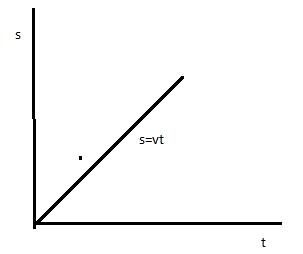
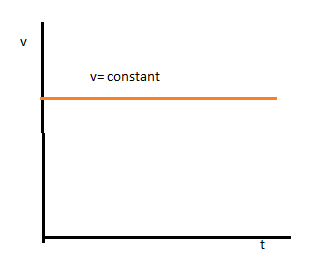
In case of uniformly accelerated motion the velocity is increasing with respect to time. The velocity is increasing with respect to time and the displacement is also increasing with respect to time.
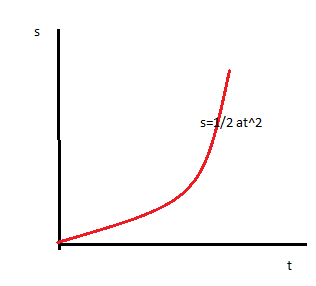
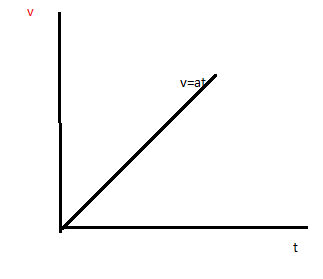
Note:- Here is a diagram that shows the relation between displacement, velocity & acceleration.
Slope of velocity - time curve gives the value of instantaneous acceleration & the slope of displacement - time curve gives the value of instantaneous velocity.
Formulae Used:
Where
Complete step-by-step solution:
Since instantaneous acceleration is given by
Rearranging the terms
Integrating both sides , we get
Since, the area of the curve between acceleration – time graph shows the change in velocity of the moving object.
Hence, option ( B ) is the correct answer.
In case of uniform motion velocity remains constant , and displacement is the linear function of velocity as


In case of uniformly accelerated motion the velocity is increasing with respect to time. The velocity is increasing with respect to time and the displacement is also increasing with respect to time.


Note:- Here is a diagram that shows the relation between displacement, velocity & acceleration.
Slope of velocity - time curve gives the value of instantaneous acceleration & the slope of displacement - time curve gives the value of instantaneous velocity.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




