Question

The base of a right prism is a pentagon whose sides are in the ratio \[1:\sqrt{2}:\sqrt{2}:1:2\] and height is 10 cm. If the longest side of base is 6, the volume of the prism is

Answer 1

Hint: Let us assume the sides of the pentagon as \[x,\sqrt{2}x,\sqrt{2}x,x,2x\]. Now we have to find the largest side among \[x,\sqrt{2}x,\sqrt{2}x,x,2x\]. Now we have to obtain a relation between the largest side in terms of x and 6. This will give the value of x. Now we should draw a pentagon of required sides. Now we should divide the pentagon into a triangle and rectangle. Now we should the area of the triangle and area of the rectangle. Now we will add the obtained area. This will give us the area of the pentagon. We know that area of prism is equal to area of base and height of prism. In this way, we will find the volume of the prism.

Complete step by step solution:
In the question, we were given that the sides of the pentagon are in the ratio \[1:\sqrt{2}:\sqrt{2}:1:2\].
Let us assume the sides of the pentagon as \[x,\sqrt{2}x,\sqrt{2}x,x,2x\]. Among these sides of pentagon, it is clear that 2x is the largest side.
From the question, it is clear that 6 is the length of the longest side.
So, we get
\[\begin{align}
  & \Rightarrow 2x=6 \\
 & \Rightarrow x=3....(1) \\
\end{align}\]
From equation (1), it is clear that the sides of the pentagon are equal to \[3,3\sqrt{2},3\sqrt{2},3,6\].

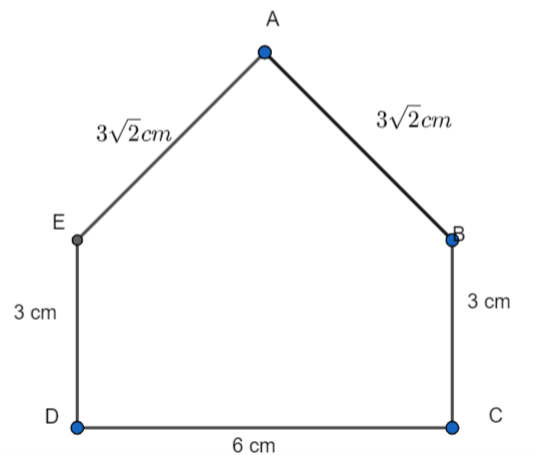
The above diagram represents the required pentagon.
Let us join points E and B in the required pentagon.

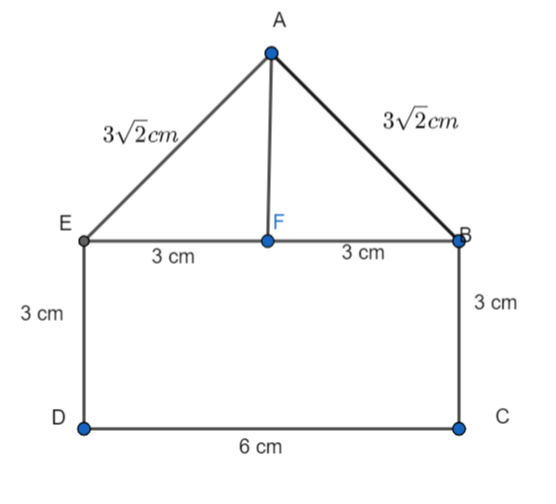
Now it is clear that the pentagon is divided into a triangle and a rectangle.
We know that if the length of the rectangle is equal to l and breadth of a rectangle is equal to b, then the area of the rectangle is equal to lb.
In the pentagon, the length of the pentagon is equal to 6 cm and the breadth of the pentagon is equal to 3 cm.
Let us assume the area of the rectangle in the pentagon is equal to A.
\[\begin{align}
  & \Rightarrow A=(3)(6)c{{m}^{2}} \\
 & \Rightarrow A=18c{{m}^{2}}....(1) \\
\end{align}\]
From the \[\Delta ABE\], it is clear that the length of AE is equal to \[3\sqrt{2}cm\], length of AB is equal to \[3\sqrt{2}cm\] and length of BE is equal to 6 cm. So, the \[\Delta ABE\] is said to be an isosceles triangle.
We know that in an isosceles triangle, the median and altitude will coincide. So, it is clear that the line drawn perpendicular from the vertex A to the side BE meet at the midpoint of the side BE. Let us assume the mid – point of the side BE is F.

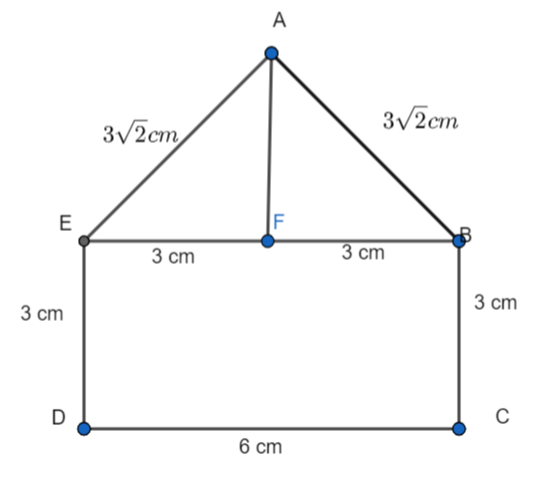
From the Pythagoras theorem,
From \[\Delta AFE\], we get
\[\begin{align}
  & \Rightarrow A{{F}^{2}}+E{{F}^{2}}=A{{E}^{2}} \\
 & \Rightarrow A{{F}^{2}}={{(3\sqrt{2})}^{2}}-{{(3)}^{2}} \\
 & \Rightarrow A{{F}^{2}}=18-9 \\
 & \Rightarrow A{{F}^{2}}=9 \\
 & \Rightarrow AF=\sqrt{9}cm \\
 & \Rightarrow AF=3cm \\
\end{align}\]
So, the height of the triangle \[\Delta ABE\] is equal to 3 cm.
From the diagram, it is clear that the base of \[\Delta ABE\] is equal to 6 cm.
We know the area of the triangle is equal to half of the product of base and height.
Let us assume the area of \[\Delta ABE\] is equal to B.
\[\begin{align}
  & \Rightarrow B=\dfrac{1}{2}\left( 6 \right)\left( 3 \right) \\
 & \Rightarrow B=9c{{m}^{2}}....(2) \\
\end{align}\]
As the area of the triangle is B and the area of the rectangle is equal t0 A, then the area of pentagon is equal to sum of A and B.
From equation (1) and equation (2), we get
Area of pentagon \[=A+B=27c{{m}^{2}}\]
We know that the volume of a prism is equal to the product of area of base and height.
We know the area of the base is equal to \[45c{{m}^{2}}\] and height of the prism is equal to 10 cm.
So, the volume of pentagonal prism is equal to the area of pentagon and height of prism.
Let us assume the volume of pentagonal prism is equal to V.
\[\begin{align}
  & \Rightarrow V=(27)(10)c{{m}^{3}} \\
 & \Rightarrow V=270c{{m}^{3}} \\
\end{align}\]
So, the volume of the pentagonal base is equal to \[270c{{m}^{3}}\].

Note: The area of the triangle mentioned in this solution can be found in an alternative way.
We know that the sides of \[\Delta ABE\] are AE, EB and EA whose lengths are \[3\sqrt{2},6,3\sqrt{2}\] respectively.
Let us assume
\[\begin{align}
  & a=3\sqrt{2}....(1) \\
 & b=6....(2) \\
 & c=3\sqrt{2}....(3) \\
\end{align}\]
We know that if a, b and c are the sides of a triangle, then the area of the triangle is equal to \[\sqrt{s(s-a)(s-b)(s-c)}\] where s is semi-perimeter of the triangle.
From equation (1), equation (2) and equation (3), we get
\[\begin{align}
  & \Rightarrow s=\dfrac{a+b+c}{2} \\
 & \Rightarrow s=\dfrac{3\sqrt{2}+6+3\sqrt{2}}{2} \\
 & \Rightarrow s=\dfrac{6\sqrt{2}+6}{2} \\
 & \Rightarrow s=3+3\sqrt{2}....(4) \\
\end{align}\]
From equation (1), equation (2), equation (3) and equation (4), we can find the area of the triangle of \[\Delta ABE\].
So,
\[\begin{align}
  & \Rightarrow \text{Area of }\Delta ABE=\sqrt{\left( 3+3\sqrt{2} \right)\left( 3 \right)(-3+3\sqrt{2})(3)} \\
 & \Rightarrow \text{Area of }\Delta ABE=\sqrt{(9)(9)}=9c{{m}^{2}} \\
\end{align}\]