Answer
458.4k+ views
Hint: To solve this question, we should know the basic trigonometric relations between angles and sides of a right-angled triangle. In the question, we can infer that AB is perpendicular to OA which implies that $\Delta OAB$ is a right-angled triangle. We can infer that for the given angle $\theta $, the side AB is the opposite side, the side OA is the adjacent side and OB is the hypotenuse. We can write that $\tan \theta =\dfrac{\text{Opposite side}}{\text{Adjacent side}}=\dfrac{AB}{OA}=\dfrac{AB}{r}$ and $\sec \theta =\dfrac{\text{Hypotenuse}}{\text{Adjacent side}}=\dfrac{OB}{OA}=\dfrac{OB}{r}$. We know that the required perimeter is the sum of the lengths of AB, PB, arc PA. We can get the length AB from the above relations, PB from the relation that $PB=OB-OP=OB-r$. The length of the arc of a circle of radius r and the included angle $\theta $ is given by the formula $\text{arc length}=\dfrac{\theta }{{{360}^{\circ }}}\times 2\pi r$. By summing up the values, we will get the required perimeter.
Complete step-by-step solution:
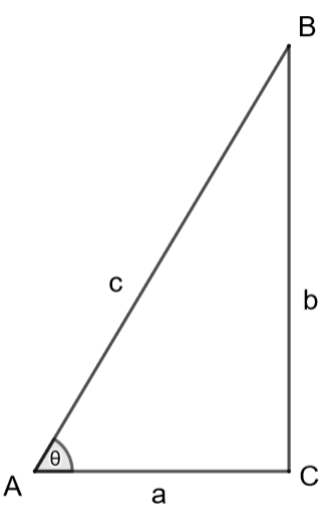
For the given triangle ABC, the angle $\angle A=\theta $ and angle $\angle C={{90}^{\circ }}$. We can infer that the triangle is a right-angled triangle.
For the given angle $\theta $, we can write that AC = a is the adjacent side, BC = b is the opposite side and AB = c is the hypotenuse.
From the basic definitions of trigonometric t=ratios, we can write that
$\tan \theta =\dfrac{\text{Opposite side}}{\text{Adjacent side}}=\dfrac{b}{a}$
$\sec \theta =\dfrac{\text{Hypotenuse}}{\text{Adjacent side}}=\dfrac{c}{a}$
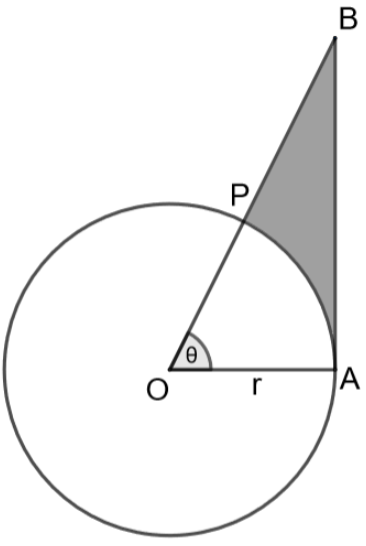
Let us consider the triangle OAB from the above diagram,
As given in the question, we can write that angle $\angle A={{90}^{\circ }}$, the triangle is a right angled triangle. For the given angle $\theta $, we can write that the side AB is opposite side, the side OA is adjacent side and OB is hypotenuse. We can write that
$\begin{align}
& \tan \theta =\dfrac{\text{Opposite side}}{\text{Adjacent side}}=\dfrac{AB}{OA}=\dfrac{AB}{r} \\
& AB=r\tan \theta \to \left( 1 \right) \\
\end{align}$
$\begin{align}
& \sec \theta =\dfrac{\text{Hypotenuse}}{\text{Adjacent side}}=\dfrac{OB}{OA}=\dfrac{OB}{r} \\
& OB=r\sec \theta \to \left( 2 \right) \\
\end{align}$
The length of the arc of a circle of radius r and the included angle $\theta $ is given by the formula $\text{arc length}=\dfrac{\theta }{{{360}^{\circ }}}\times 2\pi r$.
The length of the arc PA which includes an angle $\theta $ at the centre is
$PA=\dfrac{\theta }{{{360}^{\circ }}}\times 2\pi r=\dfrac{{{\theta }^{\circ }}}{180}\times \pi r\to \left( 3 \right)$
We can write the length of the side PB as $PB=OB-OP$
As we know that OP = r, we can write that
$PB=OB-r\to \left( 4 \right)$
We know that the required perimeter is the sum of AB, PB, arc PA. Mathematically,
$Perimeter=AB+PB+arc\left( PA \right)$
From the equations 1, 2, 3, 4, we can write that
$\begin{align}
& Perimeter=r\tan \theta +OB-r+\dfrac{{{\theta }^{\circ }}}{180}\times \pi r \\
& Perimeter=r\tan \theta +r\sec \theta -r+\dfrac{{{\theta }^{\circ }}}{180}\times \pi r \\
\end{align}$
By taking r common in the whole R.H.S, we get that
$\begin{align}
& Perimeter=r\left( \tan \theta +\sec \theta +\dfrac{{{\theta }^{\circ }}}{180}\times \pi -1 \right) \\
& Perimeter=r\left( \tan \theta +\sec \theta +\dfrac{\pi {{\theta }^{\circ }}}{180}-1 \right) \\
\end{align}$
$\therefore $ Hence proved that the required perimeter is $Perimeter=r\left( \tan \theta +\sec \theta +\dfrac{\pi {{\theta }^{\circ }}}{180}-1 \right)$.
Note: Students can make a mistake while calculating the length of the arc by taking 180 in place of 360 because of a small confusion. To avoid this, the trick is to remember that circle is also an arc of angle ${{360}^{\circ }}$ and circumference is $2\pi r$. We can infer that for an angle of ${{360}^{\circ }}$, the perimeter is $2\pi r$, then for an angle of $\theta $we can apply the unitary method and get the formula for the length of the arc as $\dfrac{\theta }{{{360}^{\circ }}}\times 2\pi r$.
Complete step-by-step solution:

For the given triangle ABC, the angle $\angle A=\theta $ and angle $\angle C={{90}^{\circ }}$. We can infer that the triangle is a right-angled triangle.
For the given angle $\theta $, we can write that AC = a is the adjacent side, BC = b is the opposite side and AB = c is the hypotenuse.
From the basic definitions of trigonometric t=ratios, we can write that
$\tan \theta =\dfrac{\text{Opposite side}}{\text{Adjacent side}}=\dfrac{b}{a}$
$\sec \theta =\dfrac{\text{Hypotenuse}}{\text{Adjacent side}}=\dfrac{c}{a}$

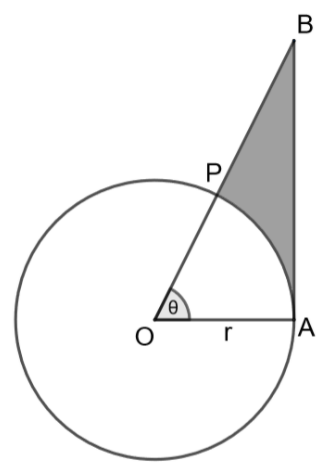
Let us consider the triangle OAB from the above diagram,
As given in the question, we can write that angle $\angle A={{90}^{\circ }}$, the triangle is a right angled triangle. For the given angle $\theta $, we can write that the side AB is opposite side, the side OA is adjacent side and OB is hypotenuse. We can write that
$\begin{align}
& \tan \theta =\dfrac{\text{Opposite side}}{\text{Adjacent side}}=\dfrac{AB}{OA}=\dfrac{AB}{r} \\
& AB=r\tan \theta \to \left( 1 \right) \\
\end{align}$
$\begin{align}
& \sec \theta =\dfrac{\text{Hypotenuse}}{\text{Adjacent side}}=\dfrac{OB}{OA}=\dfrac{OB}{r} \\
& OB=r\sec \theta \to \left( 2 \right) \\
\end{align}$
The length of the arc of a circle of radius r and the included angle $\theta $ is given by the formula $\text{arc length}=\dfrac{\theta }{{{360}^{\circ }}}\times 2\pi r$.
The length of the arc PA which includes an angle $\theta $ at the centre is
$PA=\dfrac{\theta }{{{360}^{\circ }}}\times 2\pi r=\dfrac{{{\theta }^{\circ }}}{180}\times \pi r\to \left( 3 \right)$
We can write the length of the side PB as $PB=OB-OP$
As we know that OP = r, we can write that
$PB=OB-r\to \left( 4 \right)$
We know that the required perimeter is the sum of AB, PB, arc PA. Mathematically,
$Perimeter=AB+PB+arc\left( PA \right)$
From the equations 1, 2, 3, 4, we can write that
$\begin{align}
& Perimeter=r\tan \theta +OB-r+\dfrac{{{\theta }^{\circ }}}{180}\times \pi r \\
& Perimeter=r\tan \theta +r\sec \theta -r+\dfrac{{{\theta }^{\circ }}}{180}\times \pi r \\
\end{align}$
By taking r common in the whole R.H.S, we get that
$\begin{align}
& Perimeter=r\left( \tan \theta +\sec \theta +\dfrac{{{\theta }^{\circ }}}{180}\times \pi -1 \right) \\
& Perimeter=r\left( \tan \theta +\sec \theta +\dfrac{\pi {{\theta }^{\circ }}}{180}-1 \right) \\
\end{align}$
$\therefore $ Hence proved that the required perimeter is $Perimeter=r\left( \tan \theta +\sec \theta +\dfrac{\pi {{\theta }^{\circ }}}{180}-1 \right)$.
Note: Students can make a mistake while calculating the length of the arc by taking 180 in place of 360 because of a small confusion. To avoid this, the trick is to remember that circle is also an arc of angle ${{360}^{\circ }}$ and circumference is $2\pi r$. We can infer that for an angle of ${{360}^{\circ }}$, the perimeter is $2\pi r$, then for an angle of $\theta $we can apply the unitary method and get the formula for the length of the arc as $\dfrac{\theta }{{{360}^{\circ }}}\times 2\pi r$.
Recently Updated Pages
Who among the following was the religious guru of class 7 social science CBSE

what is the correct chronological order of the following class 10 social science CBSE

Which of the following was not the actual cause for class 10 social science CBSE

Which of the following statements is not correct A class 10 social science CBSE

Which of the following leaders was not present in the class 10 social science CBSE

Garampani Sanctuary is located at A Diphu Assam B Gangtok class 10 social science CBSE

Trending doubts
Which are the Top 10 Largest Countries of the World?

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

How do you graph the function fx 4x class 9 maths CBSE

Find the value of the expression given below sin 30circ class 11 maths CBSE

In what year Guru Nanak Dev ji was born A15 April 1469 class 11 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

What is BLO What is the full form of BLO class 8 social science CBSE