
The capacitance of two concentric spherical shells of radii
(A)
(B)
(C)
(D)
Answer
483.3k+ views
Hint: To solve this question, we need to consider a charge on the system of spherical shells. Then we have to find out the potential difference in the system with the help of the charge distribution in the system. Finally, we have to apply the basic formula of the capacitance to get the final answer.
Formula used: The formulae which are used to solve this question are given by
Complete step by step answer
Let us consider the two spherical shells of radii
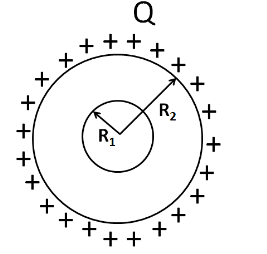
Due to the positive charge on the outer sphere, an equal amount of positive charge will appear at the outer surface of the inner sphere, as shown in the figure below.
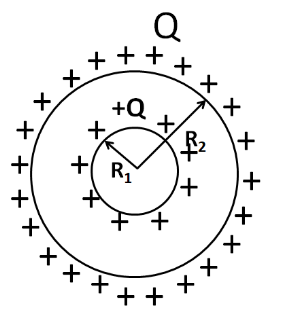
So the potential of the outer spherical shell becomes
Also the potential of the inner spherical shell is
So the net potential difference between the two spherical shells is
From (1) and (2)
On simplifying we get
Now we know that the capacitance is given by
Substituting (3) we have
On simplifying, we finally get
Thus, the capacitance of the given two concentric spherical shells is equal to
Hence, the correct answer is option C.
Note
This question could also be attempted by considering the electric field at an arbitrary point between the two spherical shells to find out the net potential difference. Then applying the formula for the capacitance as above will give the final answer.
Formula used: The formulae which are used to solve this question are given by
Complete step by step answer
Let us consider the two spherical shells of radii

Due to the positive charge on the outer sphere, an equal amount of positive charge will appear at the outer surface of the inner sphere, as shown in the figure below.

So the potential of the outer spherical shell becomes
Also the potential of the inner spherical shell is
So the net potential difference between the two spherical shells is
From (1) and (2)
On simplifying we get
Now we know that the capacitance is given by
Substituting (3) we have
On simplifying, we finally get
Thus, the capacitance of the given two concentric spherical shells is equal to
Hence, the correct answer is option C.
Note
This question could also be attempted by considering the electric field at an arbitrary point between the two spherical shells to find out the net potential difference. Then applying the formula for the capacitance as above will give the final answer.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




