
The change in current through a junction diode is
Answer
466.5k+ views
Hint: Dynamic resistance is also known as AC resistance, as typical resistance formula, it depends on change in current and voltage. Here, we take these from the operating point q in the V-I characteristics of a diode. For every small change in voltage it experienced a large change in current.
Formula used:
Complete answer:
As we know about resistance, it is the opposing force against flow of current. In case of a diode there are 2 types of resistances. Static and dynamic. Static resistance is the resistance when the diode is applied to DC and Dynamic resistance is the resistance offered by the diode when AC is applied. It is also known as AC resistance.
When we examine the V-I characteristics of p-n junction diode as shown in the picture,
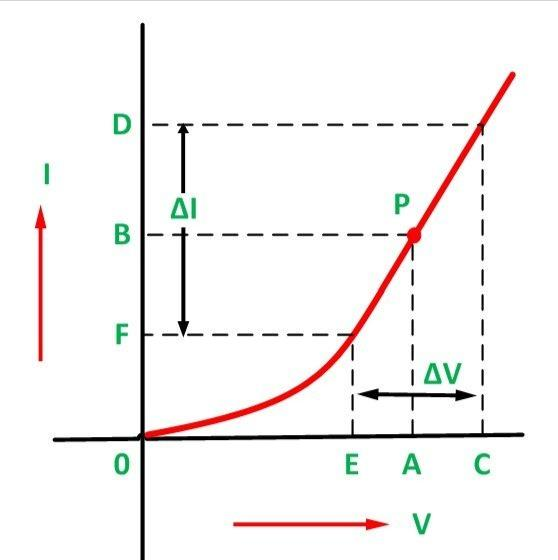
The ratio of change in voltage
I.e.,
In the question, it is given that,
Change in voltage,
So, we found that the dynamic resistance of the diode at the given voltage is
Therefore, the correct answer is option A .
Note:
We can also do the dynamic resistance problem only by knowing diode current too. The formula is given as,
Here,
This formula is derived from the diode current equation,
Formula used:
Complete answer:
As we know about resistance, it is the opposing force against flow of current. In case of a diode there are 2 types of resistances. Static and dynamic. Static resistance is the resistance when the diode is applied to DC and Dynamic resistance is the resistance offered by the diode when AC is applied. It is also known as AC resistance.
When we examine the V-I characteristics of p-n junction diode as shown in the picture,

The ratio of change in voltage
I.e.,
In the question, it is given that,
Change in voltage,
So, we found that the dynamic resistance of the diode at the given voltage is
Therefore, the correct answer is option A .
Note:
We can also do the dynamic resistance problem only by knowing diode current too. The formula is given as,
Here,
This formula is derived from the diode current equation,
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Draw ray diagrams each showing i myopic eye and ii class 12 physics CBSE

Why do the transition elements have higher enthalpies class 12 chemistry CBSE

What are the advantages of parallel combination over class 12 physics CBSE

What are the causes of overloading class 12 physics CBSE

How do you convert from joules to electron volts class 12 physics CBSE

There is hypertension due to smoking Give scientific class 12 biology CBSE




