
The compound
(Given that the atomic weight of
A)
B)
C)
D) None of the above
Answer
472.2k+ views
Hint: The density of compound
Where , Z is the number of atoms in the crustal structure.
Here,
Complete Solution :
We are provided the following data in the problem.
The molar mass of copper chloride
- The edge length ‘a’ of the copper chloride compound is equal to ,
Here ,we wan to determine the density in terms of cm thus ,convert the meters into the cm as ,
Therefore edge length becomes:
- We have to find the Density of the crystal structure.
The density of the crystal structure is related to the edge length .The relation is given as follows:
Where , d is the density of the crystal structure
Z is the number of atoms per unit structure
M is the molar mass of the compound
‘a’ is the edge length of the crystal structure and
- We know that the number of units per cell is 4, it means that it has a face-centered cubic (fcc) structure.
Lets substitute the values in the formula of density, then it can be written as:
Therefore , Density of copper chloride is
So, the correct answer is “Option A”.
Note: In copper chloride
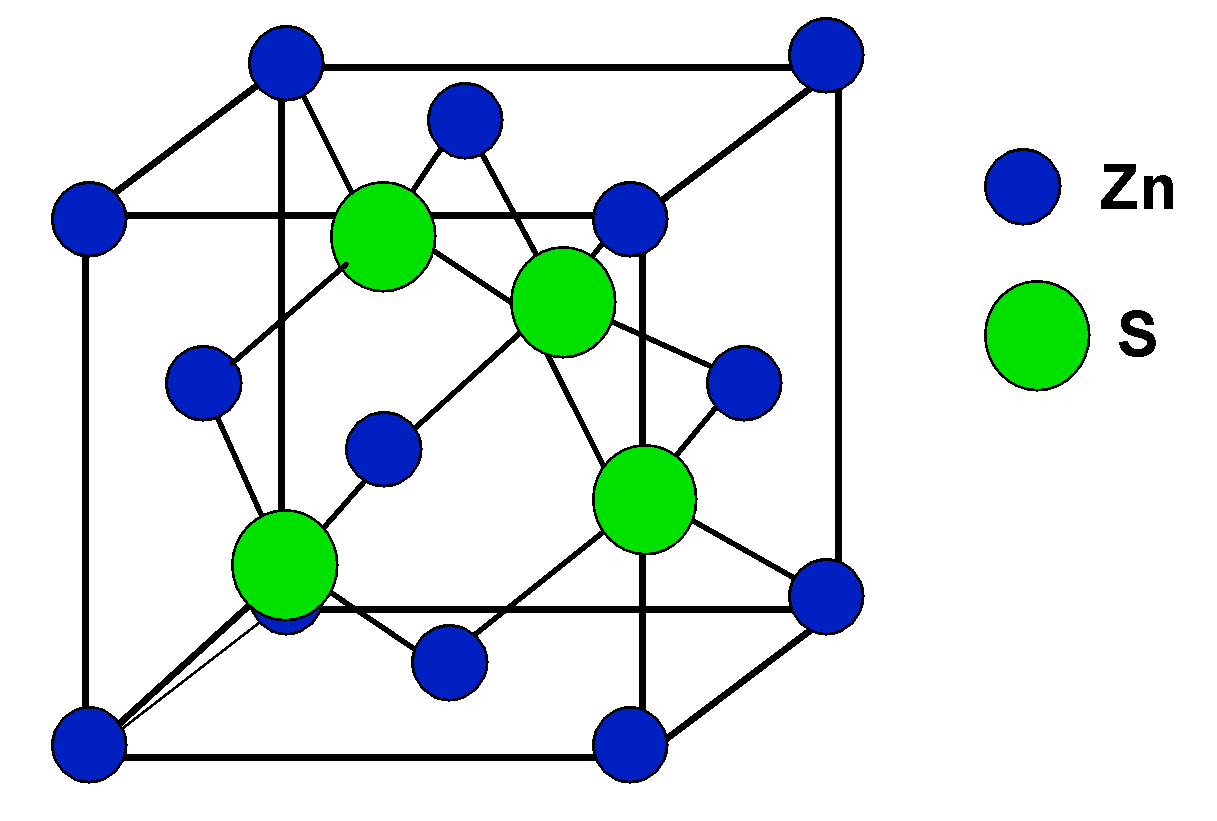
There is no need to get confused by the zinc blende structure, it is just a trick. The structure of the zinc blend
It relates to the fcc structure of the unit cell, and that will help in identifying the value of Z, i.e. the number of units per cell, which is 4.
Where , Z is the number of atoms in the crustal structure.
Here,
Complete Solution :
We are provided the following data in the problem.
The molar mass of copper chloride
- The edge length ‘a’ of the copper chloride compound is equal to ,
Here ,we wan to determine the density in terms of cm thus ,convert the meters into the cm as ,
Therefore edge length becomes:
- We have to find the Density of the crystal structure.
The density of the crystal structure is related to the edge length .The relation is given as follows:
Where , d is the density of the crystal structure
Z is the number of atoms per unit structure
M is the molar mass of the compound
‘a’ is the edge length of the crystal structure and
- We know that the number of units per cell is 4, it means that it has a face-centered cubic (fcc) structure.
Lets substitute the values in the formula of density, then it can be written as:
Therefore , Density of copper chloride is
So, the correct answer is “Option A”.
Note: In copper chloride

There is no need to get confused by the zinc blende structure, it is just a trick. The structure of the zinc blend
It relates to the fcc structure of the unit cell, and that will help in identifying the value of Z, i.e. the number of units per cell, which is 4.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write a short note on Franklands reaction class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




