
The contribution of particle at the edge centre of a particular unit cell is:
(A)
(B)
(C) 1
(D)
Answer
504k+ views
2 likes
Hint: The contribution of a particle always depends on the number of unit cells it is being shared with, which depends on its position. An edge of a cubic unit cell is common between 4 different unit cells.
Complete step by step answer:
-First of all we will talk about what a unit cell is.
Basically a unit cell is the smallest repeating unit of a crystal lattice or we can say that building block of the crystal. And the arrangement in 3D form of the atoms, molecules and ions inside of any crystal is known as a crystal lattice. Many unit cells combine together to form a crystal lattice. Each lattice point is occupied by an atom, molecules or an ion. Based on these arrangement there are 3 types of unit cells:
(1) Primitive cubic unit cell: In this atoms are present at all the corners of the unit cell.
(2) Body-centered cubic unit cell: In this atoms are present at the corners as well as the body centre of the unit cell.
(3) Face-centered cubic unit cell: Here atoms are present at the corners and the face centres of the unit cell.
-In each of the above given unit cells we can calculate the contribution of each atom to that particular unit cell and also the total number of atoms present in it.
The contribution of each atom to that unit cell depends on the factor that it is being shared by how many unit cells. If it is present in only one unit cell like the body-centered atom then its contribution will be 1, if that atom is shared by 2 unit cells then it contributes
-Now let us check the contribution of atoms at different lattice positions.
For atoms at the body centre: it is not entirely present in 1 unit cell so contribution will be = 1.
For atoms at the corners of the unit cell: When many unit cells join to form a crystal lattice then the corners of 8 unit cells meet at one point. It would look like 4 unit cells above and 4 exactly below it, sharing the corner at the centre. So, an atom at the corner is shared by 8 unit cells and thus its contribution is
For atoms at the face centres: Each face is always shared by 2 unit cells. Hence any atom at the centre of the face will also be shared by 2 unit cells and so its contribution will be
For an atom at the edge centre: An edge of the cubic unit cell is always shared between 4 unit cells. Hence an atom at the edge centre will be shared between 4 unit cells and its contribution to one unit cell will be
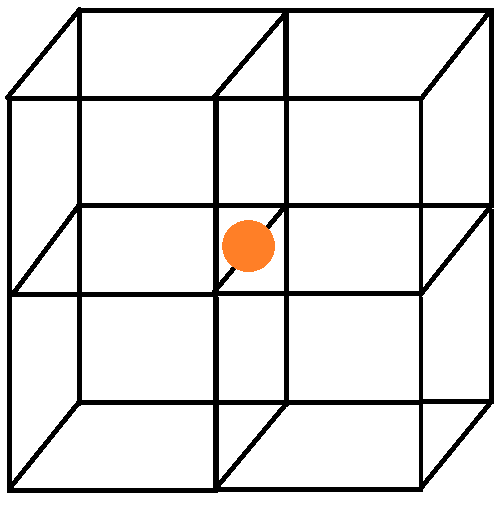
So, the correct answer is “Option B”.
Note: To find out the total number of atoms in a unit cell, we multiply the contributions of atoms at various lattice positions to the number of such atoms and then add them all. For example: for a simple cubic cell which has atoms at all the corners, total number of atoms here = 8 corners ×
Complete step by step answer:
-First of all we will talk about what a unit cell is.
Basically a unit cell is the smallest repeating unit of a crystal lattice or we can say that building block of the crystal. And the arrangement in 3D form of the atoms, molecules and ions inside of any crystal is known as a crystal lattice. Many unit cells combine together to form a crystal lattice. Each lattice point is occupied by an atom, molecules or an ion. Based on these arrangement there are 3 types of unit cells:
(1) Primitive cubic unit cell: In this atoms are present at all the corners of the unit cell.
(2) Body-centered cubic unit cell: In this atoms are present at the corners as well as the body centre of the unit cell.
(3) Face-centered cubic unit cell: Here atoms are present at the corners and the face centres of the unit cell.
-In each of the above given unit cells we can calculate the contribution of each atom to that particular unit cell and also the total number of atoms present in it.
The contribution of each atom to that unit cell depends on the factor that it is being shared by how many unit cells. If it is present in only one unit cell like the body-centered atom then its contribution will be 1, if that atom is shared by 2 unit cells then it contributes
-Now let us check the contribution of atoms at different lattice positions.
For atoms at the body centre: it is not entirely present in 1 unit cell so contribution will be = 1.
For atoms at the corners of the unit cell: When many unit cells join to form a crystal lattice then the corners of 8 unit cells meet at one point. It would look like 4 unit cells above and 4 exactly below it, sharing the corner at the centre. So, an atom at the corner is shared by 8 unit cells and thus its contribution is
For atoms at the face centres: Each face is always shared by 2 unit cells. Hence any atom at the centre of the face will also be shared by 2 unit cells and so its contribution will be
For an atom at the edge centre: An edge of the cubic unit cell is always shared between 4 unit cells. Hence an atom at the edge centre will be shared between 4 unit cells and its contribution to one unit cell will be

So, the correct answer is “Option B”.
Note: To find out the total number of atoms in a unit cell, we multiply the contributions of atoms at various lattice positions to the number of such atoms and then add them all. For example: for a simple cubic cell which has atoms at all the corners, total number of atoms here = 8 corners ×
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




