
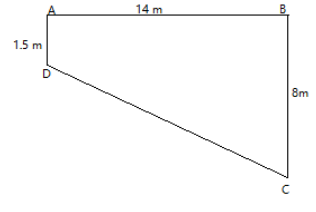
The cross-section

Answer
503.4k+ views
1 likes
Hint: Construct the line
Complete step-by-step answer:
We will first construct the line
We know that the area of the triangle is
From the figure it is evident that,
Therefore
Substituting the value 6.5 for
Thus, area of the triangle
Also, the area of the rectangle is given by the formula
Substituting the value of base with
Area of
Substituting the value 14 for
Area of
The area of the cross-section
Area of
Area of
Thus the area of the cross section
Note: The formula of the area of the trapezium can also be used alternatively. The area of the trapezium is given by
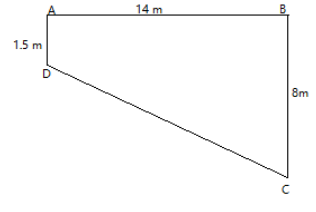
Complete step-by-step answer:
We will first construct the line

We know that the area of the triangle is
From the figure it is evident that,
Therefore
Substituting the value 6.5 for
Thus, area of the triangle
Also, the area of the rectangle is given by the formula
Substituting the value of base with
Area of
Substituting the value 14 for
Area of
The area of the cross-section
Area of
Area of
Thus the area of the cross section
Note: The formula of the area of the trapezium can also be used alternatively. The area of the trapezium is given by
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
EMI starts from ₹3,108.34 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

Describe the factors why Mumbai is called the Manchester class 9 social science CBSE

Explain the importance of pH in everyday life class 9 chemistry CBSE

What is the N in statistics class 9 maths CBSE

What is the Full Form of ICSE / ISC ?

What are the major achievements of the UNO class 9 social science CBSE




