
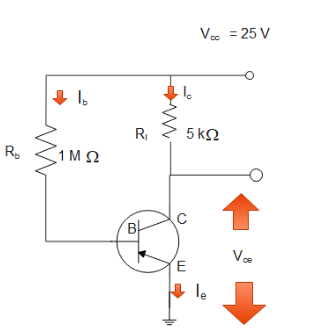
The current gain of a common emitter transistor circuit shown in figure is

Answer
414k+ views
Hint: The way to solve these types of questions are using the standard formulas of DC Load line and Voltage Divider Bias formulas. We have been given the value of
Complete step by step answer:
We know that by the formula of voltage divider Bias:
Thus, we can get base current from the equation:
Thus,
Now, we know that collector current is related to base current by the formula:
hence,
Now by using
Thus
Now we can easily plot the Q point by using the values of
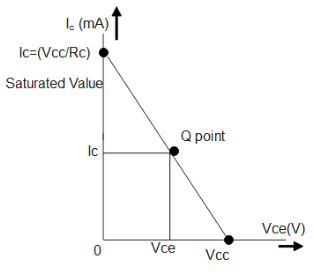
Note: These sums come only with this one type of questions, just find the variables needed and graph it, if the value of
Complete step by step answer:
We know that by the formula of voltage divider Bias:
Thus, we can get base current from the equation:
Thus,
Now, we know that collector current is related to base current by the formula:
hence,
Now by using
Thus
Now we can easily plot the Q point by using the values of

Note: These sums come only with this one type of questions, just find the variables needed and graph it, if the value of
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




