
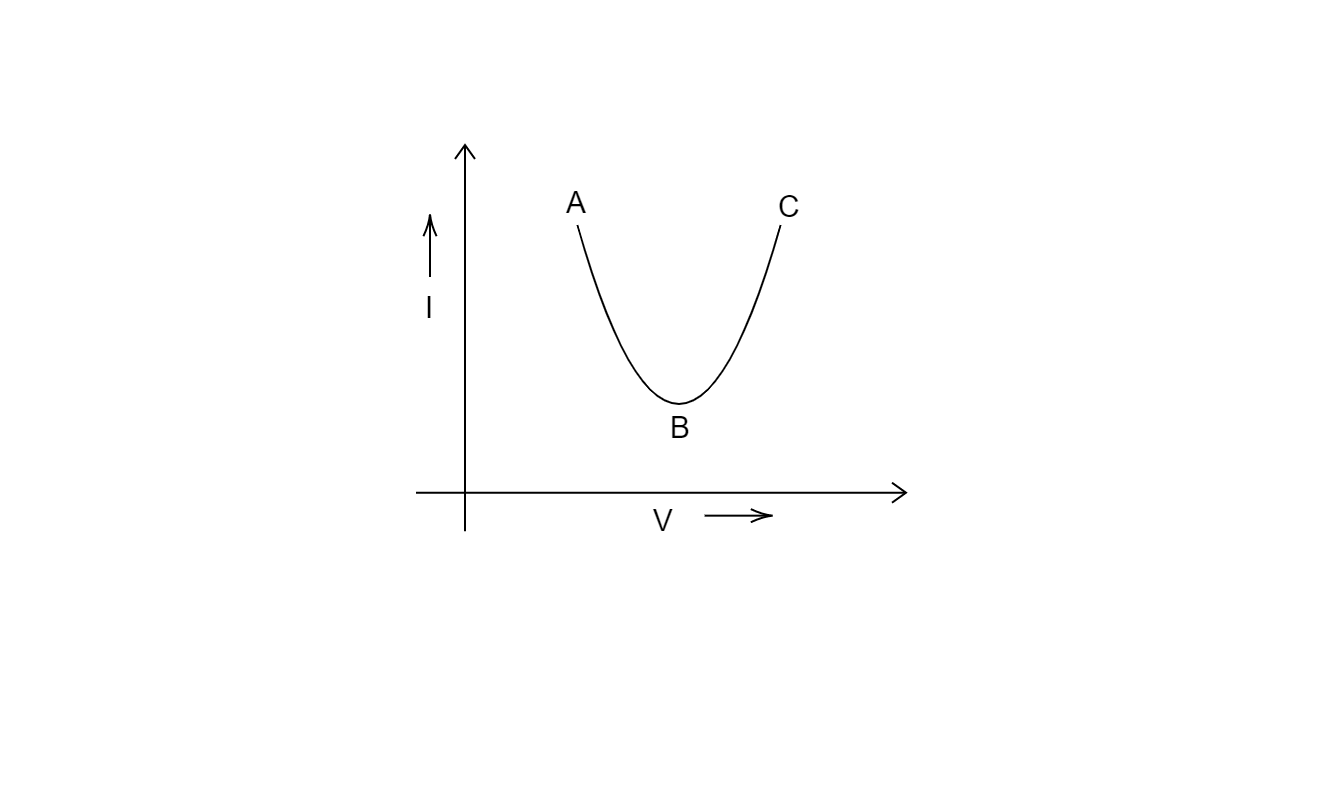
The current-voltage graph for a device is shown in figure. The resistance is negative in region:
(a) AB
(b) BC
(c) ABC
(d) None of these

Answer
487.5k+ views
Hint: Resistance is given as
Complete step by step answer:
From Ohm’s Law we have,
And the graph is I vs V so the slope will be,
On further simplification,
Now from the graph we see that for part AB the slope is negative, for point B slope is zero and for part BC the slope is positive. Therefore, the part for which the resistance is negative is AB.
The correct answer is option A.
Note:Be careful in evaluating the slope. Read the axes carefully whether V is on x-axis or y-axis. Many times students evaluate slope m=R=V/I in a hurry. Well the answer remains the same and correct in case of multiple choice. But in case of subjective questions, marks will be deducted.
Complete step by step answer:
From Ohm’s Law we have,
And the graph is I vs V so the slope will be,
On further simplification,
Now from the graph we see that for part AB the slope is negative, for point B slope is zero and for part BC the slope is positive. Therefore, the part for which the resistance is negative is AB.
The correct answer is option A.
Note:Be careful in evaluating the slope. Read the axes carefully whether V is on x-axis or y-axis. Many times students evaluate slope m=R=V/I in a hurry. Well the answer remains the same and correct in case of multiple choice. But in case of subjective questions, marks will be deducted.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




